In an equilateral triangle ABC, G is the centroid. Each side of the triangle is 6 cm. The length of AG is
2√2cm
3√2cm
2√3cm
3√3cm
To find the length of AG in the equilateral triangle ABC, we can follow these steps:
Step 1: Understand the properties of an equilateral triangle
In an equilateral triangle, all sides are equal, and the centroid (G) divides each median in a 2:1 ratio. The median connects a vertex to the midpoint of the opposite side.
Step 2: Calculate the length of the median
For an equilateral triangle with side length a, the length of the median m can be calculated using the formula:
m=√32⋅a
Given that the side length a=6 cm, we can substitute this value into the formula:
m=√32⋅6=3√3 cm
Step 3: Determine the length of AG
Since G is the centroid, it divides the median AD in a ratio of 2:1. This means that:
AG=23⋅AD
Substituting the length of AD (which is the median we calculated):
AG=23⋅3√3=2√3 cm
Final Answer
The length of AG is 2√3 cm.
---
In an equilateral triangle, all sides are equal, and the centroid (G) divides each median in a 2:1 ratio. The median connects a vertex to the midpoint of the opposite side.
For an equilateral triangle with side length a, the length of the median m can be calculated using the formula:
m=√32⋅a
Given that the side length a=6 cm, we can substitute this value into the formula:
m=√32⋅6=3√3 cm
Since G is the centroid, it divides the median AD in a ratio of 2:1. This means that:
AG=23⋅AD
Substituting the length of AD (which is the median we calculated):
AG=23⋅3√3=2√3 cm
The length of AG is 2√3 cm.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Each side of an equilateral triangle is 10 cm long. The height of the triangle is
A10√3 cmB5√3 cmC10√2 cmD5 cmFind the ratio of the areas of an equilateral triangle ABC and square EFGC, if G is the centroid of the triangle ABC.
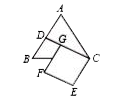 A27:16B1:4C4:3√3D3√3:4
A27:16B1:4C4:3√3D3√3:4Each side of an equilateral triangle measures 8 cm. The area of the triangle is
A8√3cm2B√34×82cm2C32√3cm2D48 cm2
Each side of an equilateral triangle is 6 cm. Its altitude is
View SolutionIn an equilateral triangle of side 3√3 cm then length of the altitude is
View SolutionLet G be the centroid of the equilateral triangle ABC of perimeter 24 cm. Then the length of AG is
View SolutionAn equilateral triangle ABC is inscribed in a circle of radius 20√3 cm
What is the length of the side of the triangle?
View SolutionIn an equilateral triangle ABC of side 10cm, the side BC is trisected at D. Then the length (in cm) of AD is
View Solution
