One end of a string of length L is tied to the celling of lift accelerating upwards with an accelerating 2g The other end of the string is free. The linear mass density of the string varies linearly from 0 of λ from bottom to top :-
The velocity of the wave in the string will be 0
The acceleration of the wave in the string will be 3g/4 every where
The time taken by a pulse to reach from bottom to top will be √8L/3g
The time taken by a pulse to reach from bottom to top will be √4L/3g
The correct Answer is:B, C
To solve the problem step by step, we will follow the reasoning presented in the video transcript.
Step 1: Understanding the Problem
We have a string of length L tied to the ceiling of a lift that is accelerating upwards with an acceleration of 2g. The linear mass density of the string varies linearly from 0 at the top to λ at the bottom. We need to find the velocity of the wave in the string.
Step 2: Determine the Linear Mass Density
The linear mass density μ(y) at a distance y from the bottom of the string can be expressed as:
μ(y)=λLy
This is because the mass density varies linearly from 0 to λ as we move from the top to the bottom of the string.
Step 3: Calculate the Tension in the String
To find the tension T in the string, we consider a small element of the string of length dy at a distance y from the bottom. The mass of this small element is:
dm=μ(y)⋅dy=λLy⋅dy
The forces acting on this element are the tension T acting upwards and the weight of the element acting downwards, which is dm⋅g. Since the lift is accelerating upwards with 2g, we can set up the equation:
T−dm⋅g=dm⋅(2g)
Substituting dm:
T−(λLy⋅dy)g=(λLy⋅dy)(2g)
Rearranging gives:
T=3(λLy⋅dy)g
Step 4: Substitute for the Tension
Now we can express the tension T in terms of y:
T=3λgyL
Step 5: Calculate the Velocity of the Wave
The velocity v of the wave in the string is given by the formula:
v=√Tμ
Substituting the expressions for T and μ:
v=
⎷3λgyLλLy=√3gy1=√3gy
Step 6: Find the Acceleration of the Wave
The acceleration a of the wave can be found by differentiating the velocity with respect to y:
a=dvdt=vdvdy
Calculating dvdy:
dvdy=3g2√3gy
Thus, the acceleration becomes:
a=√3gy⋅3g2√3gy=3g2
Step 7: Time Taken for a Pulse to Travel from Bottom to Top
To find the time t taken for a pulse to travel from the bottom to the top of the string, we can use:
v=dydt
Rearranging gives:
dt=dy√3gy
Integrating from 0 to L:
t=∫L0dy√3gy=23√g(√y)L0=23√g√L
Thus, the total time taken is:
t=8L3g
Final Answers
1. The velocity of the wave in the string is √3gy.
2. The time taken for a pulse to travel from the bottom to the top of the string is 8L3g.
We have a string of length L tied to the ceiling of a lift that is accelerating upwards with an acceleration of 2g. The linear mass density of the string varies linearly from 0 at the top to λ at the bottom. We need to find the velocity of the wave in the string.
The linear mass density μ(y) at a distance y from the bottom of the string can be expressed as:
μ(y)=λLy
This is because the mass density varies linearly from 0 to λ as we move from the top to the bottom of the string.
To find the tension T in the string, we consider a small element of the string of length dy at a distance y from the bottom. The mass of this small element is:
dm=μ(y)⋅dy=λLy⋅dy
The forces acting on this element are the tension T acting upwards and the weight of the element acting downwards, which is dm⋅g. Since the lift is accelerating upwards with 2g, we can set up the equation:
T−dm⋅g=dm⋅(2g)
Substituting dm:
T−(λLy⋅dy)g=(λLy⋅dy)(2g)
Rearranging gives:
T=3(λLy⋅dy)g
Now we can express the tension T in terms of y:
T=3λgyL
The velocity v of the wave in the string is given by the formula:
v=√Tμ
Substituting the expressions for T and μ:
v=
⎷3λgyLλLy=√3gy1=√3gy
The acceleration a of the wave can be found by differentiating the velocity with respect to y:
a=dvdt=vdvdy
Calculating dvdy:
dvdy=3g2√3gy
Thus, the acceleration becomes:
a=√3gy⋅3g2√3gy=3g2
To find the time t taken for a pulse to travel from the bottom to the top of the string, we can use:
v=dydt
Rearranging gives:
dt=dy√3gy
Integrating from 0 to L:
t=∫L0dy√3gy=23√g(√y)L0=23√g√L
Thus, the total time taken is:
t=8L3g
1. The velocity of the wave in the string is √3gy.
2. The time taken for a pulse to travel from the bottom to the top of the string is 8L3g.
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-03)
31 Videos
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[A]
29 Videos
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-01)
53 Videos
- SEMICONDUCTORSBOOK - ALLENCHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
51 Videos
Similar Questions
One end of a string of length L is tied to the ceiling of a lift accelerating upwards with an acceleration 2g. The other end o the string is free. The linear mass density of the string varies linearly from 0 to λ from bottom to top. The acceleration of a wave pulled through out the string is pg4. Find p.
View SolutionOne end of a string of length L is tied to the ceiling of a lift acceleration upwards with an acceleration 2g. The other end of the string is free. The linear mass density of the string varies linearly from 0→λ from bottom to top. Choose the correct option(s)
View SolutionA stone of mass m tied to a string of length l is rotated in a circle with the other end of the string as the centre. The speed of the stone is v. If the string breaks, the stone will move
View SolutionA mass M is lowered with the help of a string by a distance h at a distance acceleration g/2. The work done by the string will be
View SolutionA body of mass M is hanging by an inextensible string of mass m. If the free end of the string accelerates up with constant acceleration a. find the variation of tension in the string a function of the distance measured from the mass M (bottom of the string).

View SolutionA block of mass m tied to a string is lowered by a distance d, at a constant acceleration of g/3. The work done by the string is
View SolutionA block of mass m tied to a string is lowered by a distance d, at a constant acceleration of g/3. The work done by the string is
View SolutionA block of mass m tied to a string is lowered by a distance d, at a constant acceleration of g/3. The work done by the string is
View SolutionThe acceleration of the 2 kg block if the free end of string is pulled with a force of 20 N as shown is
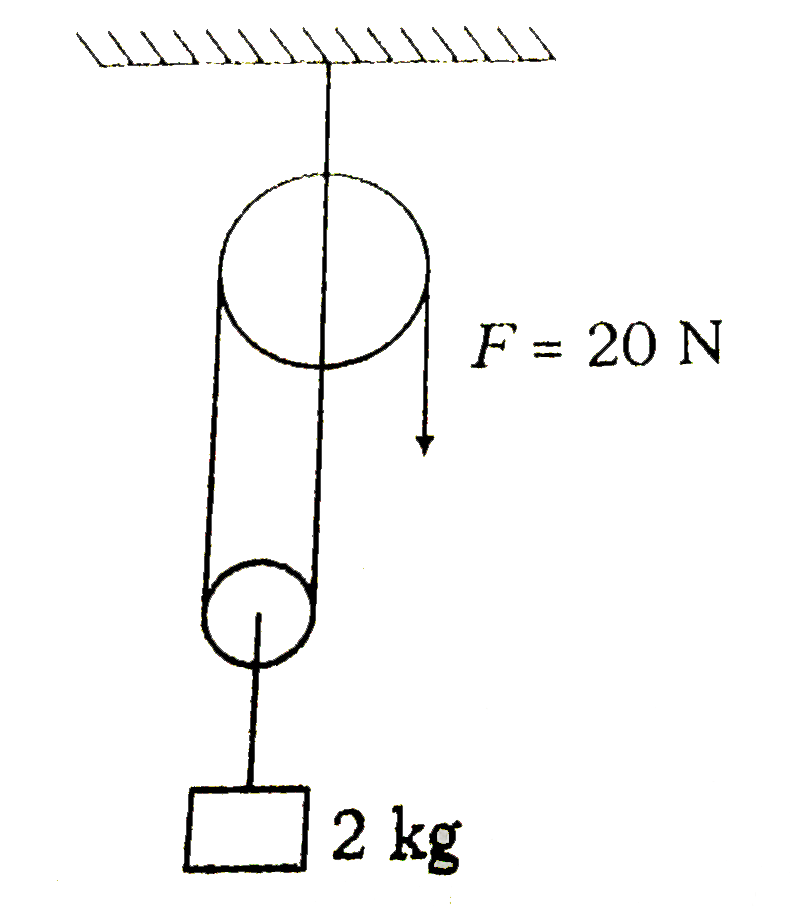
View SolutionA mass of 100 gm is tied to one end of a string 2 m long. The body is revolving in a horizontal circle making a maximum of 200 revolutions per min. The other end of the string is fixed at the centre of the circle of revolution. The maximum tension that the string can bear is (approximately)
View Solution
