P,Q and R are three particle of a medium which lie on the x-axis. A sin wave of wavelength λ is travelling through the medium in the x-direction. P and Q always have the same speed. Whilem P and R always have the same velocity. The minimum distance between :-
P and Q is λ2
P and Q is λ
P and R is λ2
P and R is λ
The correct Answer is:A, D
To solve the problem, we need to analyze the motion of the three particles P, Q, and R in relation to the sine wave traveling through the medium.
1. Understanding the Setup:
- We have three particles P, Q, and R on the x-axis.
- A sine wave of wavelength λ is traveling in the positive x-direction.
- P and Q have the same speed, while P and R have the same velocity.
2. Speed vs. Velocity:
- Speed is a scalar quantity and does not have a direction, while velocity is a vector quantity that includes direction.
- Since P and Q have the same speed, they can be moving in different directions but at the same rate.
- Since P and R have the same velocity, they must be moving in the same direction and at the same rate.
3. Determining the Speeds:
- Let's denote the speed of P and Q as v.
- Since they are moving with the same speed, we can say vP=vQ=v.
- For P and R, since they have the same velocity, we can denote their velocity as vP=vR.
4. Distance Between Particles:
- The distance between P and Q can be determined based on their speeds and the time they have been moving.
- Since P and Q have the same speed, the distance between them will depend on their initial positions and the time they have been moving.
- The distance between P and R will be determined by their velocities.
5. Minimum Distance Calculation:
- Given that the wavelength of the sine wave is λ, and since P and R have the same velocity, the distance between them can be considered as λ when they are in phase with the wave.
- Therefore, the minimum distance between P and R is equal to the wavelength λ.
6. Conclusion:
- The minimum distance between the particles P, Q, and R is determined by their respective speeds and velocities in relation to the sine wave.
- The minimum distance between P and R is λ.
Final Answer:
The minimum distance between particles P, Q, and R is λ.
---
- We have three particles P, Q, and R on the x-axis.
- A sine wave of wavelength λ is traveling in the positive x-direction.
- P and Q have the same speed, while P and R have the same velocity.
- Speed is a scalar quantity and does not have a direction, while velocity is a vector quantity that includes direction.
- Since P and Q have the same speed, they can be moving in different directions but at the same rate.
- Since P and R have the same velocity, they must be moving in the same direction and at the same rate.
- Let's denote the speed of P and Q as v.
- Since they are moving with the same speed, we can say vP=vQ=v.
- For P and R, since they have the same velocity, we can denote their velocity as vP=vR.
- The distance between P and Q can be determined based on their speeds and the time they have been moving.
- Since P and Q have the same speed, the distance between them will depend on their initial positions and the time they have been moving.
- The distance between P and R will be determined by their velocities.
- Given that the wavelength of the sine wave is λ, and since P and R have the same velocity, the distance between them can be considered as λ when they are in phase with the wave.
- Therefore, the minimum distance between P and R is equal to the wavelength λ.
- The minimum distance between the particles P, Q, and R is determined by their respective speeds and velocities in relation to the sine wave.
- The minimum distance between P and R is λ.
The minimum distance between particles P, Q, and R is λ.
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-03)
31 Videos
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[A]
29 Videos
- WAVES AND OSCILLATIONSBOOK - ALLENCHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-01)
53 Videos
- SEMICONDUCTORSBOOK - ALLENCHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
51 Videos
Similar Questions
A,B and C are the three particles of a medium which are equally sparated and lie along the x-axis. When a sinusoidal transverse wave of wavelength k propagates along the x-axix, the following observation are made:
(i) A and B have the same speed. (ii) A and C have the same velocity.
Find (a) the minium distance between A and B and (b) the minimum distance between A and C.

View SolutionA sine wave is travelling ina medium. The minium distance between the two particles, always having same speed is
View SolutionIn sine wave , minimum distance between 2 particles always having same speed is
View SolutionThe displacement of a particle in a medium due to a wave travelling in the x−direction through the medium is given by y=Asin(αt−βx), where t= time, and α and β are constants:
View SolutionThe diagram below shows three different modes of vibration P, Q and R of the same string of a given length.
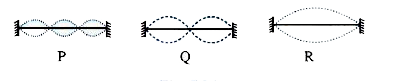
What is the ratio of the wavelength of vibrations P and R?
View SolutionThree particles start from the origin at the same time, one with a velocity v1 along the x-axis, second along the y-axis with a velocity v2 and third particle moves along the line x = y. The velocity of third particle, so that three may always lie on the same line is:
View SolutionWhen two waves of the same amplitude and frequency but having a phase difference of ϕ, travelling with the same speed in the same direction (positive x), interfere, then
View SolutionThe adjacent diagram shows three different modes of vibrations P, Q and R of the same string.
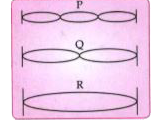
State the ratio of wavelengths of P and R.
View SolutionThe adjacent diagram shows three different modes of vibrations P, Q and R of the same string.
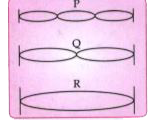
The sound of which string will have maximum shrillness?
View SolutionIn the following displacement (x) vs time (t) graph, at which among the point P,Q and R is the object speed increasing ?

View Solution
