Question

A small particle of mass m is fixed to the perimeter of a ring of same mass and radius r. The system comprising of particle and ring is placed on a horizontal plane. Friction is negligible on horizontal plane. Initially particle is at top most point, then the system is released from rest. Answer next two question when the particle is at the same height as the centre of ring aftr being released from top most point. Assume that the ring stays in vertical plane during its motion under consideration

Mark the CORRECT option(s):

Options

A. Speed of centre of mass of system is `sqrt((gr)/(4))`
B. Angular velocity of system is `omega=sqrt((g)/(r))`
C. Contact point of ring with ground is instantaneously at rest
D. Centre of ring is instantaneously at rest

Answer

A::B::D

Explanation

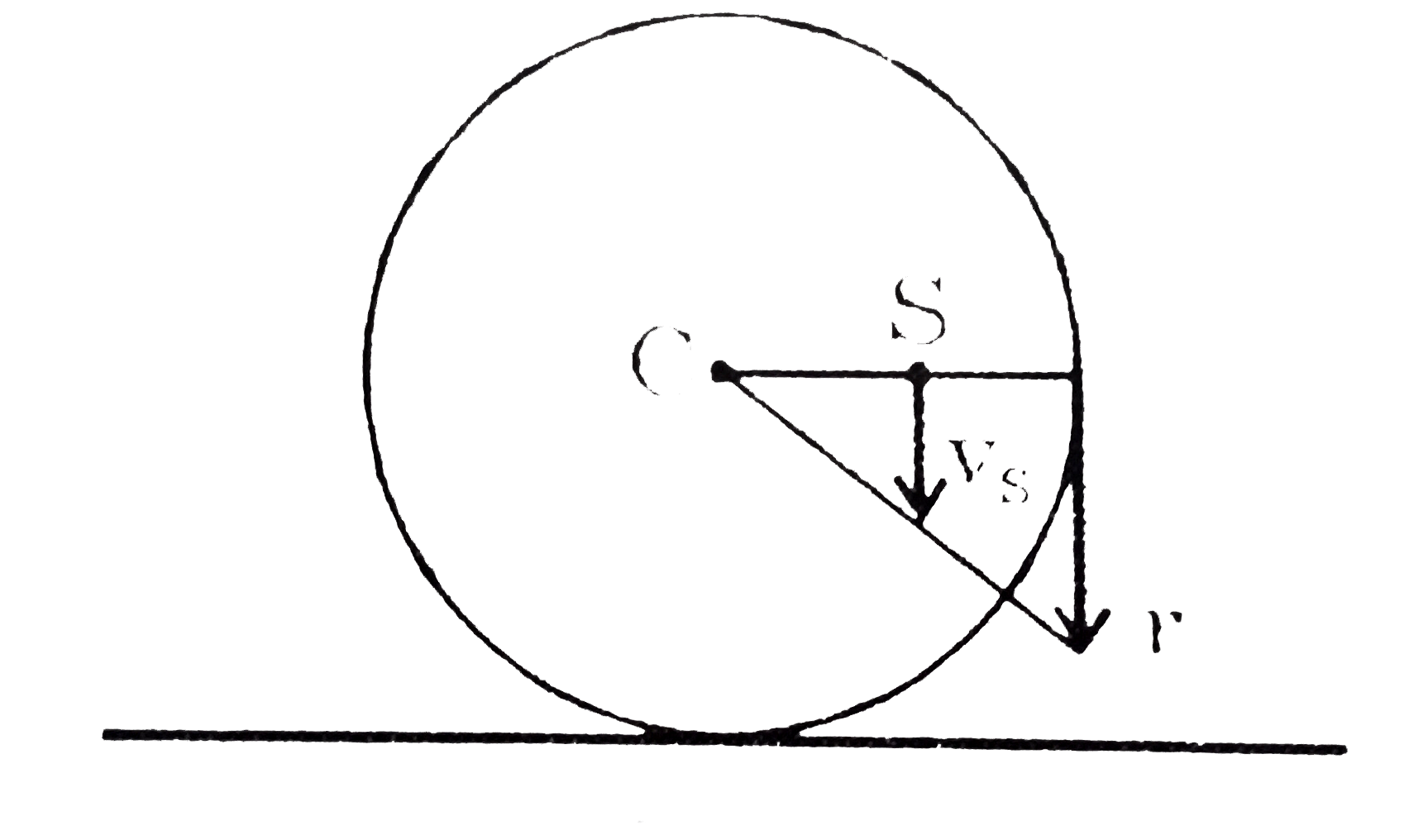
In the absence of horizontal external force centre of mass of system S falls vertically. Since mass of ring and particle are equal their motion about CM of system is symmetrical. At given instant centre of ring will be instantaneous centre
From work energy theorem
`mgr=(1)/(2)mv_(1)^(2)+(1)/(2)mr^(2)omega^(2)`
also `v_(1)=romega`
thus `v_(1)=sqrt(gr)`
`|vec(a)_(C )|=|vec(a)_(1)|=(v_(rel)^(2))/((r//2))=(v_(1)//2)^(2)/(r//2)=(g)/(2)`
`[v_(rel)=v_(1)-v_(S)=(v_(1))/(2)]`
At the given instant acceleration of particle is equal to the centripetal acceleration of its rotaion about centre of mass.

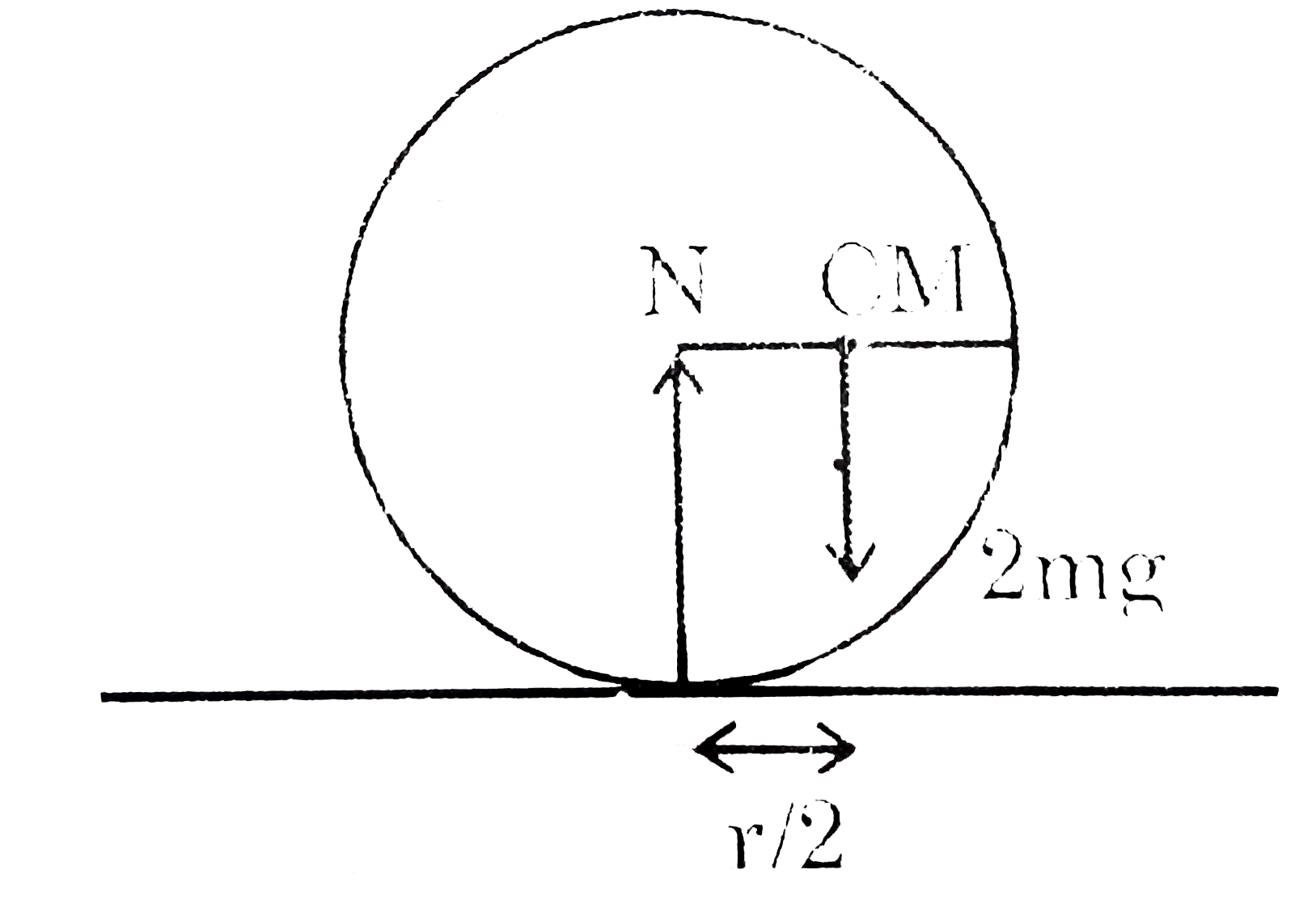
`N,(r)/(2)=(3)/(2) mr^(2)alpha " " ....(i)`
`a_(s)=(r)/(2)alpha" "...(ii)`
`a_(s)=(2mg-N)/(2m)" "...(iii)`
Thus `N=(3)/(2)mg`