Consider three identical metal spheres and . Sphere carries charge , sphere carries charge and sphere carries no charge . Spheres A and B are touched together and then separated. Sphere is then touched to sphere and separated from it . Finally the sphere is touched to sphere and separated from it . Find the final charge on the sphere .
The correct Answer is:
To find the final charge on sphere C after the described interactions, we will follow these steps:
Step 1: Touch spheres A and B
- Sphere A has a charge of +6q and sphere B has a charge of −3q.
- When two conductive spheres are touched, they share their total charge equally.
- The total charge before touching is:
Qtotal=+6q+(−3q)=+3q
- Since the spheres are identical, the charge on each after they are separated will be:
Q′A=Q′B=Qtotal2=3q2=1.5q
Step 2: Touch sphere C to sphere A
- Now, sphere A has a charge of +1.5q and sphere C has a charge of 0.
- The total charge when A and C are touched is:
Qtotal=1.5q+0=1.5q
- After touching, the charge will again be shared equally:
Q′′A=Q′C=Qtotal2=1.5q2=0.75q
- Therefore, after this step:
- Charge on sphere A: 0.75q
- Charge on sphere C: 0.75q
Step 3: Touch sphere C to sphere B
- Now, sphere B has a charge of +1.5q and sphere C has a charge of +0.75q.
- The total charge when B and C are touched is:
Qtotal=1.5q+0.75q=2.25q
- After touching, the charge will again be shared equally:
Q′′B=Q′′C=Qtotal2=2.25q2=1.125q
- Therefore, after this step:
- Charge on sphere B: 1.125q
- Charge on sphere C: 1.125q
Final Result
The final charge on sphere C is:
Final charge on sphere C=1.125q
- Sphere A has a charge of +6q and sphere B has a charge of −3q.
- When two conductive spheres are touched, they share their total charge equally.
- The total charge before touching is:
Qtotal=+6q+(−3q)=+3q
- Since the spheres are identical, the charge on each after they are separated will be:
Q′A=Q′B=Qtotal2=3q2=1.5q
- Now, sphere A has a charge of +1.5q and sphere C has a charge of 0.
- The total charge when A and C are touched is:
Qtotal=1.5q+0=1.5q
- After touching, the charge will again be shared equally:
Q′′A=Q′C=Qtotal2=1.5q2=0.75q
- Therefore, after this step:
- Charge on sphere A: 0.75q
- Charge on sphere C: 0.75q
- Now, sphere B has a charge of +1.5q and sphere C has a charge of +0.75q.
- The total charge when B and C are touched is:
Qtotal=1.5q+0.75q=2.25q
- After touching, the charge will again be shared equally:
Q′′B=Q′′C=Qtotal2=2.25q2=1.125q
- Therefore, after this step:
- Charge on sphere B: 1.125q
- Charge on sphere C: 1.125q
The final charge on sphere C is:
Final charge on sphere C=1.125q
Topper's Solved these Questions
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:Exersice-04[B]
Explore 16 Videos
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:Exercise-05(A)
Explore 35 Videos
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:Comprehension 9
Explore 2 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLENChapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-7
Explore 8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Book:ALLENChapter:PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Exercise:EXERCISE-IV
Explore 8 Videos
Similar Questions
Consider two concentric metal spheres. The outer sphere is given a charge Q>0, then
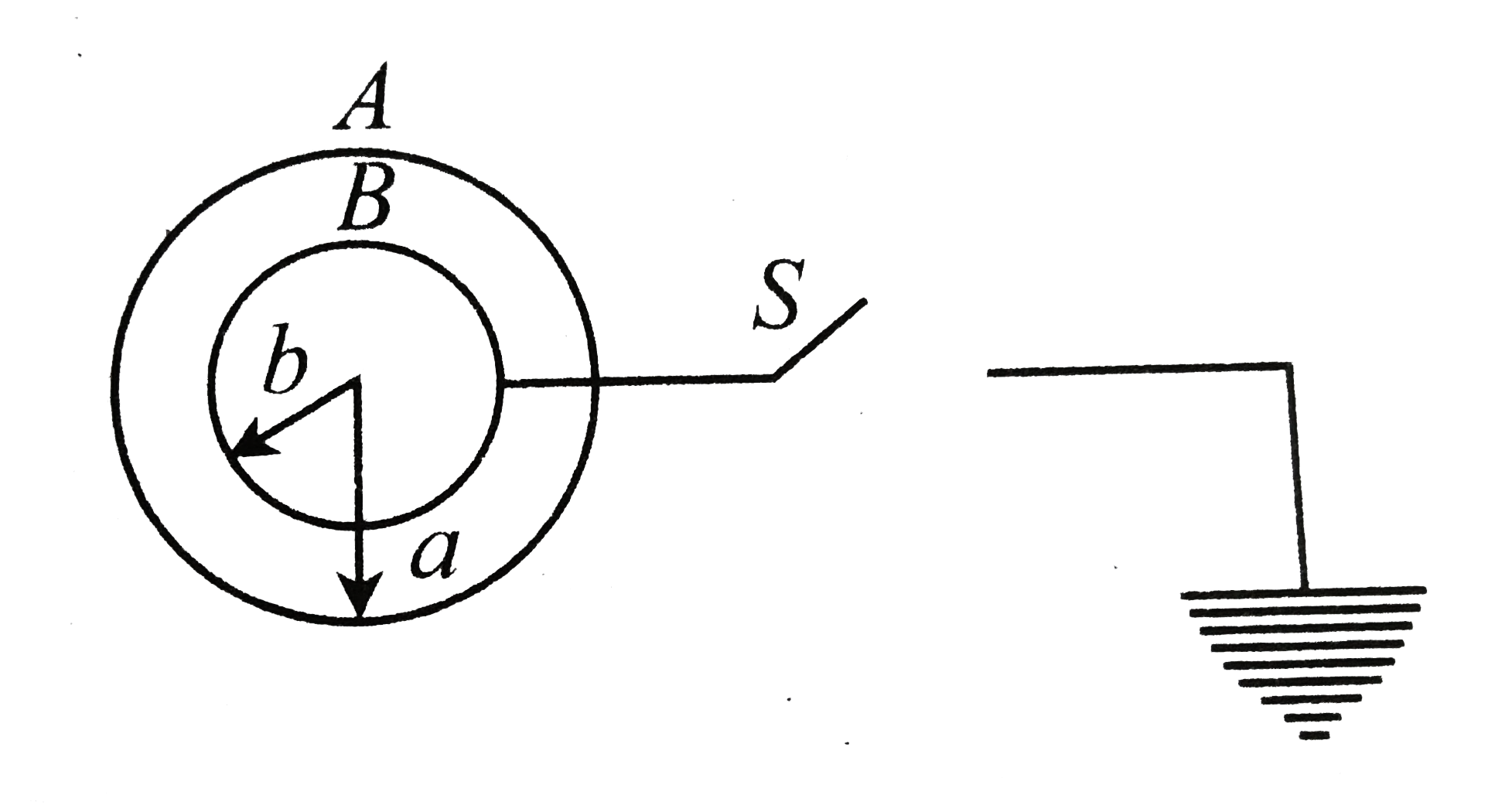 ,
,
View SolutionTwo identical conducting spheres are charged by induction and then separated by a large distance, sphere 1 has charge +Q and sphere 2 has charge -Q. A third sphere is initially uncharged. If sphere 3 is touched to sphere 1 and separated and then touched to sphere 2 and separated what is the final charge on each of the three spheres?
View Solution
Knowledge Check
The ratio of the surface area of sphere A to the surface area of sphere B is 729 : 1. What is ratio of the volume of sphere A to sphere B ?
A27:1B81:1C19,683:1D26,224:1
Two spheres A and B are charged with the charges of +10 and +20 coulomb respectively and separated by a distance of 80 cm. The electric field at point on the line joining the centres of the two spheres will be zero at a distance from the sphere A :
View SolutionTwo metal spheres A and B of radii a & b (a < b) respectively are at a large distance apart. Each sphere carries a charge of 100μO. the sphere are connected by a conducting wire, then
View SolutionA sphere carries a uniformly distributed electric charge. Prove that the field inside the sphere is zero
View SolutionThree spheres of radius 'r' are kept inside a cuylinder such that two of the speres are at bottom touching each other and sides of the cylinder while the third sphere is touching the two bottom spheres and the top of the cylinder .Find the volume of the cylinder .
View SolutionThere are two uncharged identicasl metallic spheres 1 and 2 of radius r separated by a distance d(d>>r). A charged metalllic sphere of same radius having charge q is touched with one of the sphere. After some time it is moved away fom the system. Now, the uncharged sphere is earthed. Charge on earthed sphere is
View SolutionThe diameter of a hollow metallic sphere is 60 cm and the sphere carries a charge of 500μC. The potential at a distance of 100cm from the centre of the sphere will be
View SolutionThere are three concentric conducting spherical shells. All of them are charged. The innermost sphere is connected by a conducting wire to the outermost sphere. Prove that the final charge on the innermost sphere is independent of the initial charge of outermost sphere.
View Solution
