A particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
A-R, B-Q, C-P, D-S
A-Q, B-R, C-S, D-P
A-R, B-P, C-Q, D-S
A-P, B-S, C-R, D-Q
The correct Answer is:A
Step by step video & image solution for A particle is suspended from a string of length R. It is given a velocity u = 3 sqrt(gR) at the bottom Match the following {:(,"Table-1",,"Table-2"),("(A)","Velocity at B","(P)","7 mg"),("(B)","Velocity at C","(Q)",sqrt(5gR)),("(C)","Tension in string at B","(R)",sqrt(7gR)),("(D)","Tension in string at C","(S)","5 mg"),(,,"(T)","None"):} by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLENCHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Match the following.
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Table-1Table-2(A)Coefficient of viscosity(P)[M2L−1T−2](B)Surface tension(Q)[ML0T−2](C)Modulus of elasticity(R)[ML−1T−2](D)Energy per unit volume(S)Noneof a fiuid∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionA pendulum is released from point A as shown in figure. At some instant net force one the bob is making an angle θ with the string. Then Match the following

Table-1Table-2(A)For θ=30∘(P)Particle may be moving along BA(B)For θ=120∘(Q)Particle may be moving along CB(C)For θ=90∘(R)Particle may be at A(D)For θ=0∘(S)Particle is at B(T)None
View SolutionMatch the following
Table-1Table-2(A)L(P)[M0L0T−2](B)Magnetic Flux(Q)[ML2T−2A−1](C)LC(R)[ML2T−2A−2](D)CR2(S)None
View SolutionIn the figure shown, velocity of liquid which comes out is v, time of liquid to fall to ground is t and range on ground is R. If the vessel is taken to a mountain, match the following.

∣∣ ∣ ∣ ∣∣Table-1Table-2(A)V(P)will increase(B)t(Q)will decrease(C)R(R)will remain same∣∣ ∣ ∣ ∣∣
View SolutionA particle of mass m is attached to a string of length L and given velocity √10gL in the horizontal direction at the lowest point. Find tension in the string when the particle is at (i) lowest position (ii) highest position,
View SolutionA heavy particle hangs from a point O, by a string of length a. It is projected horizontally with a velocity u=√(2+√3)ag . The angle with the downward vertical, string makes where string becomes slack is :
View SolutionIn th efigure shown in fig. 10.33 , a bob attached with a light string of radius R is given as initial velocity u=√4gR at the bottommost point.
(a) At what height string will slack.
(b) What is velocity of the bob just befare slacking of string.
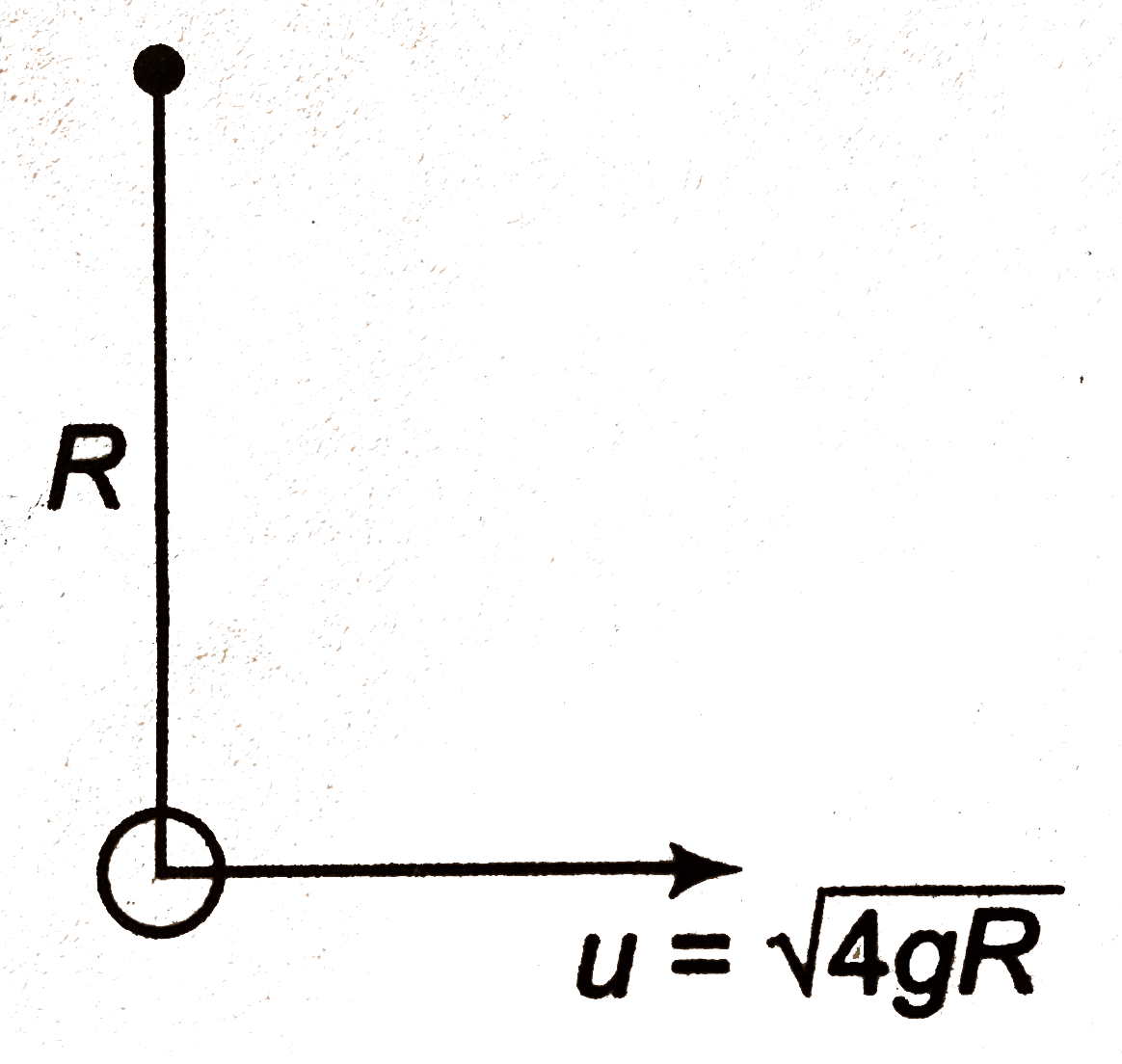
View SolutionA particle of mass 1kg has velocity →v1=(2t)ˆi and another particle of mass 2kg has velocity →v2=(t2)ˆj
Column IColumn II(A)Netforce on centre of mass at 2 s(p)209unit(B)Velocity of centre of mass at 2 s(q)√68 unit(C)Displacement of centre of mass in 2s(r)√803unit(s)None
View SolutionA particle is projected from ground with initial velocity u=20(√2)m/s at θ=45∘. Find
(a) R,H and T,
(b) velocity of particle after 1 s
(c) velocity of particle at the time of collision with the ground (x-axis).

View SolutionA particle suspended by a light inextensible thread of length l is projected horizontally from its lowest position with velocity √7gl/2. The string will slack after swinging through an angle equal to
View Solution
