A sphere of ice at 0∘C having initial radius R is placed in an environment having ambient temperature >0∘C. The ice melts uniformly, such that shape remains spherical. After a time 't' the radius of the sphere has reduced to r. which graph best depicts r(t)
_E01_119_O01.png)
_E01_119_O02.png)
_E01_119_O03.png)
_E01_119_O04.png)
The correct Answer is:B
Heat lost
=σA(T4−T40)=−σ4πr2(T40−T4)
= d(mL)dt=ρLdvdt=ρL4πr2drdt
⇒−σ4πr2(T40−T4)=σL4πr2drdt
⇒ radius decreases with time
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -03
10 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - ASSERTION-REASON
36 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -01
65 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
A solid sphere of radius 'R' density ρ and specific heat 'S' initially at temperature T0 Kelvin is suspended in a surrounding at temperature 0K. Then the time required to decrease the temperature of the sphere from T0 to T02 kelvin is (Assume sphere behaves like a black body)
View SolutionA solid copper sphere of density ρ, specific heat c and radius r is at temperature T1. It is suspended inside a chamber whose walls are at temperature 0K. What is the time required for the temperature of sphere to drop to T2? Take the emmissivity of the sphere to be equal to e.
View Solution
Knowledge Check
A block of ice, initially at −20∘C, is heated a steady rate until the temperature of the sample reaches 120∘C. Which of the following graphs best illustrates the temperature of the sample as a function of time?
A B
B C
C D
D
A sphere of density d, specific heat s and radius r is hung by a thermally insulating thread in an enclosure which is kept at a lower temperature than the sphere. The temperature of the sphere starts to drop at a rate which depends upon the temperature difference between the sphere and the enclosure. If the temperature difference is ΔT and surrounding temperature is T0 then rate of fall in temperature will be
[Given that ΔT<<T0]
View SolutionA solid sphere of radius r is melted and cast into the shape of a solid cone of height r , the radius of the base of the cone is
View SolutionA spherical conductor having charge q and radius r is placed at the centre of a spherical shell of radius R and having charge Q (R > r). The potential difference between the two is
View SolutionA sphere P (emissivity=1) of radius 2R and and another sphere Q(emissivity=1/2) of radius R are placed in vacuum at some distance threre are no other objects. The temperature of the sphere Q is maintained at 200 K by the means of a heater. A fraction 1/32 of the power emitted by the sphere Q falls on the sphere P. if the equilibrium temperature of the sphere P is 10 T kelvin find the value of T.
View SolutionA non conducting sphere of radius a has a net charge +q uniformly distributed throughout its volume. A spherical conducting shell having inner and outer radii b and c and net charge -q is concentric with the sphere (see the figure).
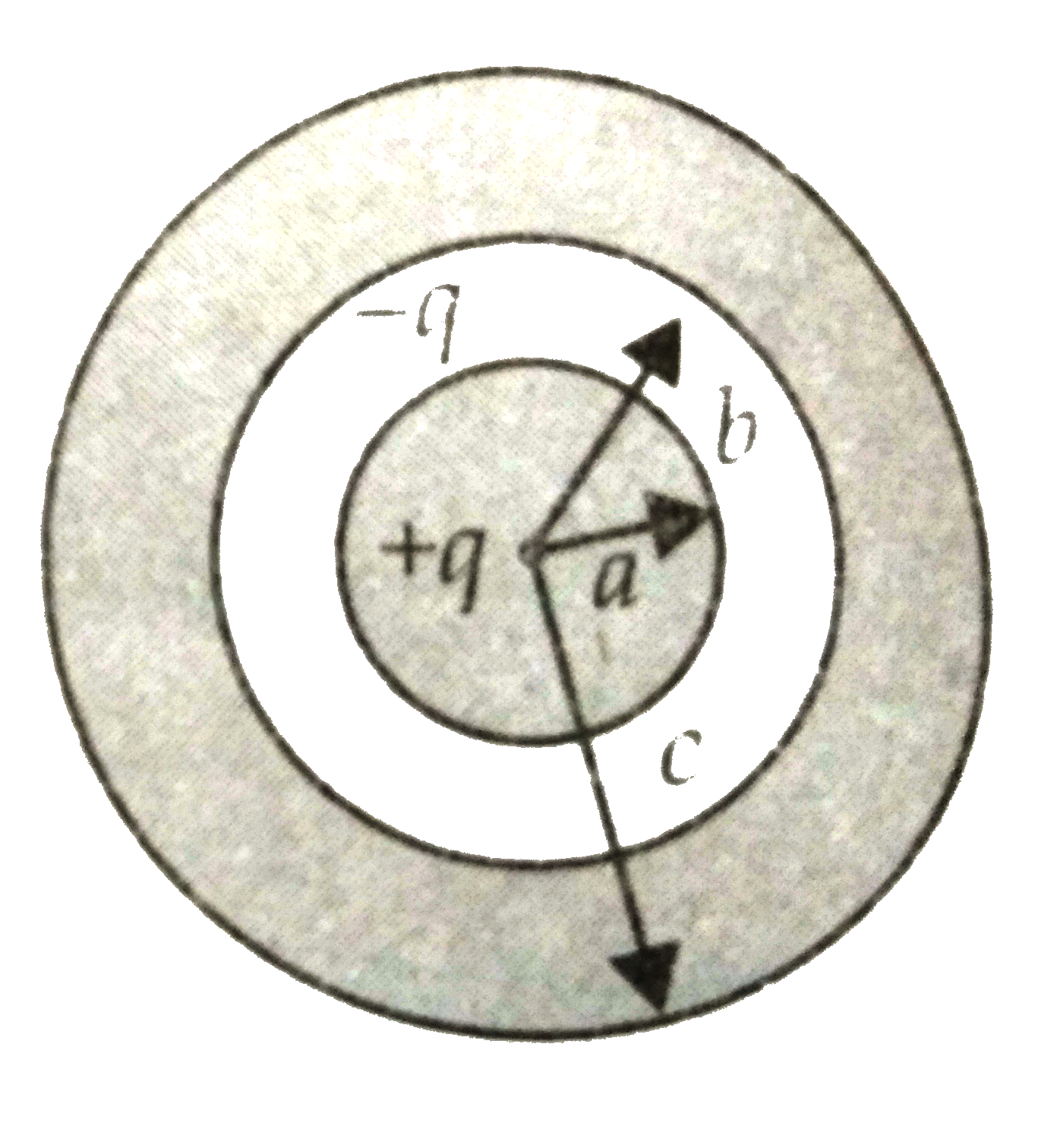
Read the following statements
(i) The electric field at a distance r from the centre of the sphere for r lt a is 14πε0qra3
(ii) The electric field at distance r for a lt r lt b is 0
(iii) The electric field at distance r for b lt r lt c is 0
(iv) The charge on the inner surface of the spherical shell is - q
(v) The charge on the outer surface of the spherical shell is + q
Which of the above statements are true?
View SolutionA sphere of density ρ and radius a has a concentric of radius b as shown in the
(a) Sketch the gravitational force exerted by the spehre on the particle of mass m located at a distance r from the centre of the sphere as a function of r in the range 0≤r≤∞
(b) Sketch the corresponding curve for the potential energy u (r) of the system
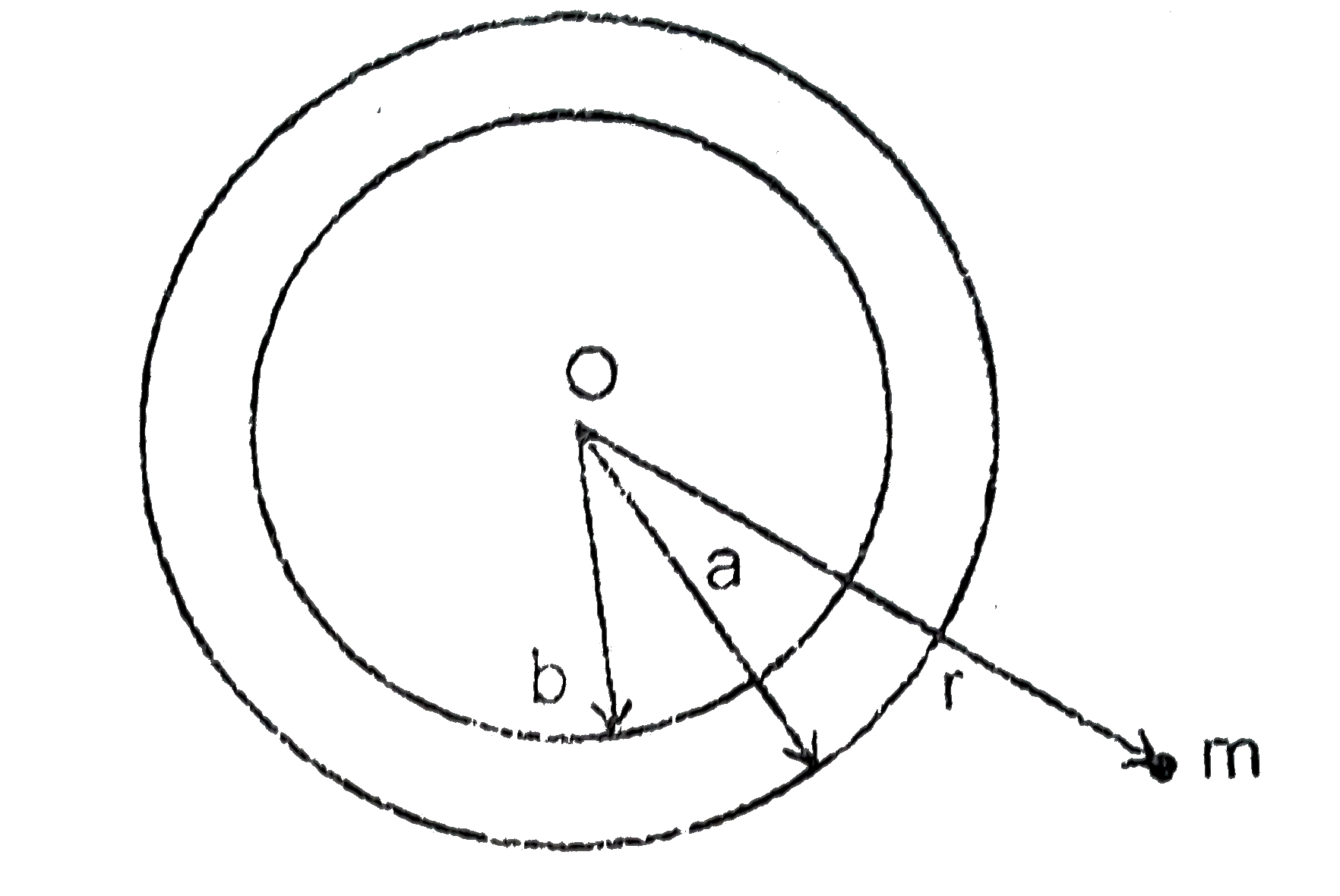 ,
,
View SolutionA sphere is placed inside a right circular cylinder so as to touch the top, base and lateral surface of the cylinder. If the radius of the sphere is r, then the volume of the cylinder is 4π r3 (b) 83π r3 (c) 2π r3 (d) 8π r3
View Solution
