STATEMENT−1 A convex lens may be diverging
STATEMENT−2 The nature of a lens depends upon the refractive incides fo the material of lens and surrounding medium besides geometry.
Statement-I is true , Statement -II is true, Statement-II is correct explanation for Statement-I.
Statement - I is true, Statement -II is true: Statement -II is NOT a correct explanation for statement -I .
Statement-I is true, Statement-II is false.
statement-I is false, statement- II is true.
The correct Answer is:D
To solve the question regarding the nature of a convex lens and its dependence on the refractive indices, we can break down the statements and analyze them step by step.
1. Understanding Statement 1:
- Statement 1 claims that "A convex lens may be diverging."
- A convex lens is typically known as a converging lens. However, this statement suggests that under certain conditions, a convex lens can behave as a diverging lens.
Hint: Consider the conditions under which a convex lens might diverge light instead of converging it.
2. Understanding Statement 2:
- Statement 2 states that "The nature of a lens depends upon the refractive indices of the material of the lens and surrounding medium besides geometry."
- This statement is true because the behavior of a lens (whether it converges or diverges light) is influenced by the refractive index of both the lens material and the medium in which it is placed.
Hint: Think about how the refractive index affects light behavior at the interface of different materials.
3. Analyzing the Conditions:
- A convex lens will converge light when it is in a medium with a lower refractive index than the lens itself (e.g., air, where the refractive index is approximately 1).
- If the lens is placed in a medium with a higher refractive index (e.g., water or another liquid), the lens can behave as a diverging lens because the light rays will bend away from the normal upon entering the lens.
Hint: Visualize how light rays behave when they pass from one medium to another with different refractive indices.
4. Conclusion:
- Since Statement 1 is correct under the condition that the lens is in a medium with a higher refractive index than the lens material, it can indeed act as a diverging lens.
- Statement 2 accurately explains why Statement 1 is true, as it highlights the importance of refractive indices in determining the nature of the lens.
Final Answer: Therefore, both statements are true, and Statement 2 is a correct explanation of Statement 1.
Summary:
- Statement 1: True (A convex lens can diverge under certain conditions).
- Statement 2: True (The nature of a lens depends on refractive indices and geometry).
- Statement 1 claims that "A convex lens may be diverging."
- A convex lens is typically known as a converging lens. However, this statement suggests that under certain conditions, a convex lens can behave as a diverging lens.
- Statement 2 states that "The nature of a lens depends upon the refractive indices of the material of the lens and surrounding medium besides geometry."
- This statement is true because the behavior of a lens (whether it converges or diverges light) is influenced by the refractive index of both the lens material and the medium in which it is placed.
- A convex lens will converge light when it is in a medium with a lower refractive index than the lens itself (e.g., air, where the refractive index is approximately 1).
- If the lens is placed in a medium with a higher refractive index (e.g., water or another liquid), the lens can behave as a diverging lens because the light rays will bend away from the normal upon entering the lens.
- Since Statement 1 is correct under the condition that the lens is in a medium with a higher refractive index than the lens material, it can indeed act as a diverging lens.
- Statement 2 accurately explains why Statement 1 is true, as it highlights the importance of refractive indices in determining the nature of the lens.
- Statement 1: True (A convex lens can diverge under certain conditions).
- Statement 2: True (The nature of a lens depends on refractive indices and geometry).
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - Comprehension Based Questions
66 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (A)
47 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -03
10 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
A bicovex lens has radii of cuvature 20 cm each. If the refractive index of the material of the lens is 1.5, what is its focal length?
View SolutionA lens behaves as a converging lens in air and a diverging lens in water. The refractive index of the material is (refractive index of water =1.33)
View Solution
Knowledge Check
A biconvex lens has focal length 23 times the radius of curvature of either surface. Calculate refraction index f material of the lens.
A1.75B1.33C1.5D1.0
A plano-convex lens is made of refractive index of 1.6. The focal length of the lens is
View SolutionA plano convex lens has radius of curvature 10 cm. Its focal length is 80 cm under water. Calculate the refractive index of the material of the lens, given refractive index of water is 4/3.
View SolutionA biconvex lens has focal length 23 times the radius of curvature of either surface. Calculate refraction index f material of the lens.
View SolutionFor a plano convex lens, the radius of curvature of convex surface is 10 cm and the focal length is 30 cm. The refractive index of the material of the lens is
View SolutionSTATEMENT -1 : A convex lens suffers from chromatic aberration.
and
STATEMENT - 2 : All parallel rays passing through a convex lens do not come to a focus at the same point.
View SolutionA convex lens of refractive index 3/2 has a power of 2.5∘. If it is placed in a liqud of refractive index 2,the new power of the lens is
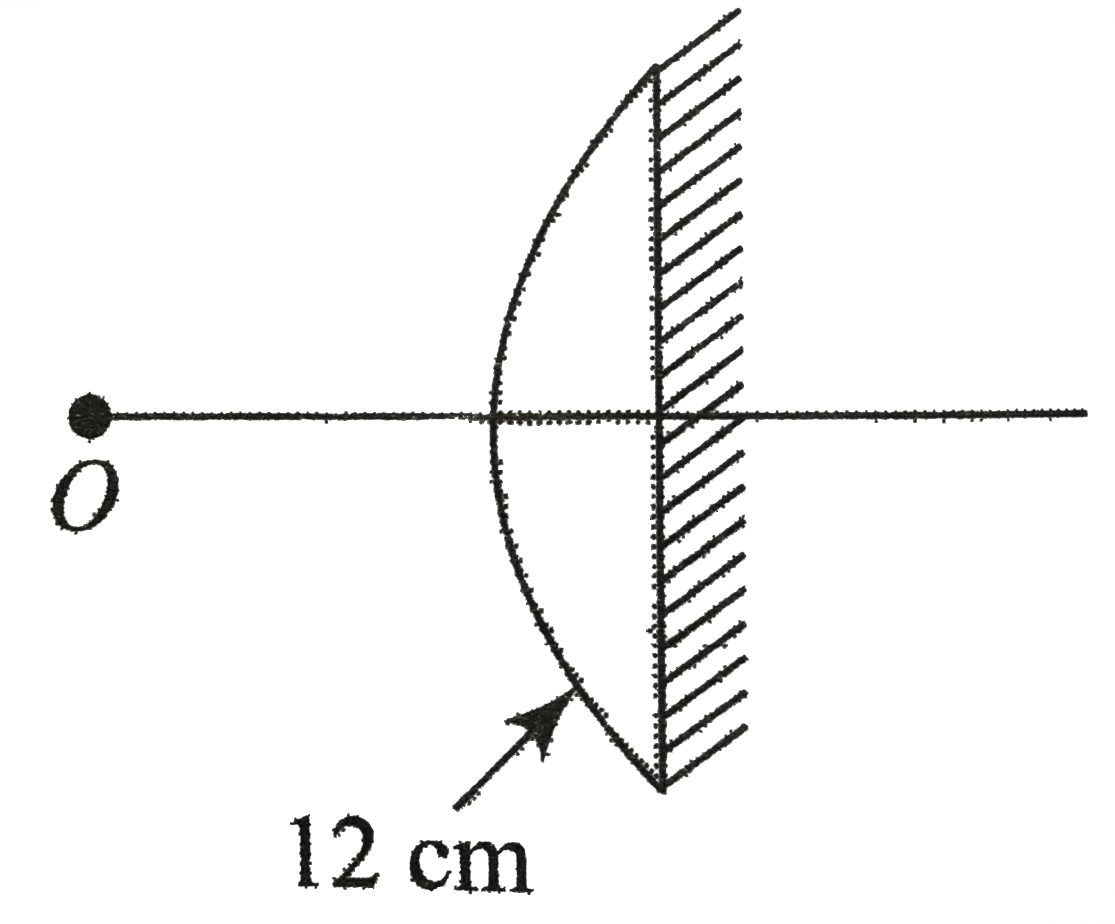
View SolutionA plano-convex lens is silvered on its plane side. The radius of curvature of the other face is 12cm and the refractive index of the material of the lens is 1.5. An object is placed 24 cm in front of the silvered lens. Where will the image be formed?
View Solution
