For the decay of nucleus, the possible reason which is not true
Neutron proton ratio too high
Neutron proton ratio too low
Size of nucleus ratio too low
Binding energy of nucleus is more than that of its products
The correct Answer is:D
To solve the question regarding the possible reasons for the decay of a nucleus and identifying which statement is not true, we can follow these steps:
Step 1: Understand the Concept of Nuclear Stability
The stability of a nucleus is primarily determined by the ratio of neutrons to protons. An ideal ratio for stability is approximately 1:1. If this ratio is too low (too few neutrons) or too high (too many neutrons), the nucleus becomes unstable and is likely to undergo decay.
Hint: Consider how the neutron-to-proton ratio affects nuclear stability.
Step 2: Analyze the Statements
We need to evaluate the given statements regarding nuclear decay and identify which one is not true. The statements typically involve:
- Neutron-to-proton ratios
- Size of the nucleus
- Binding energy
Hint: Look for statements that contradict the established principles of nuclear physics.
Step 3: Evaluate Each Statement
1. Neutron-to-proton ratio too low or too high: This statement is true. An unstable nucleus will decay if the neutron-to-proton ratio is not optimal.
2. Size of the nucleus: A statement about the size of the nucleus affecting stability is also true. If the nucleons are too close or too far apart, it can lead to instability.
3. Binding energy of the nucleus: The binding energy represents how tightly the nucleons are held together. A higher binding energy indicates a more stable nucleus. Thus, if the binding energy is more than its product (which could imply a comparison to the total mass-energy), this would indicate that the nucleus is stable, not unstable.
Hint: Consider how binding energy relates to the stability of the nucleus.
Step 4: Identify the Not True Statement
From the evaluation, the statement regarding binding energy being more than its product is not true in the context of nuclear decay. A higher binding energy means greater stability, not instability.
Conclusion: The statement that is not true regarding the decay of a nucleus is related to the binding energy.
Final Answer: The not true option is related to the binding energy of the nucleus being more than its product.
The stability of a nucleus is primarily determined by the ratio of neutrons to protons. An ideal ratio for stability is approximately 1:1. If this ratio is too low (too few neutrons) or too high (too many neutrons), the nucleus becomes unstable and is likely to undergo decay.
We need to evaluate the given statements regarding nuclear decay and identify which one is not true. The statements typically involve:
- Neutron-to-proton ratios
- Size of the nucleus
- Binding energy
1. Neutron-to-proton ratio too low or too high: This statement is true. An unstable nucleus will decay if the neutron-to-proton ratio is not optimal.
2. Size of the nucleus: A statement about the size of the nucleus affecting stability is also true. If the nucleons are too close or too far apart, it can lead to instability.
From the evaluation, the statement regarding binding energy being more than its product is not true in the context of nuclear decay. A higher binding energy means greater stability, not instability.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-02
19 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column
1 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES
29 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)
24 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
Similar Questions
Knowledge Check
Assertion: For the scattering of α-particles at a large angles, only the nucleus of the atom is responsible.
Reason: Nucleus is very heavy in comparison to electrons.Aif both assertion and reason are true and reason is the correct explanation of assertion.Bif both assertion and reason are true but reason is not the correct explanation of assertion.Cif assertion is true but reason is false.Dif both assertion and reason are false.
A radioactive nucleus A with a half life T, decays into nucleus B. At t=0, there is no nucleus B. At some time t, the ratio of the number of B to that of A is 0.3 . Then, t is given by
View SolutionIn a radioactive decay, a nucleus is transformed into another with the emission of a positron. In this process the neutron-proton ratio
View SolutionMatch the column in radioactive decay process of nucleus with atomic number Z and mass number A.
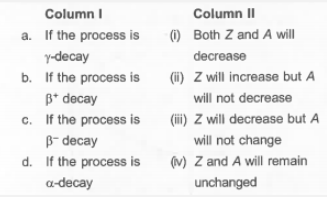
View SolutionHeavy radioactive nucleus decay through α−decay also. Consider a radioactive nucleus x. It spontaneously undergoes decay at rest resulting in the formation of a daughter nucleus y and the emission of an α− particle. The radioactive reaction can be given by
x→y+α
However , the nucleus y and the α− particle will be in motion. Then a natural question arises that what provides kinetic energy to the radioactive products. In fact, the difference of masses of the decaying nucleus and the decay products provides for the energy that is shared by the daughter nucleus and the α− particle as kinetic energy . We know that Einstein's mass-energy equivalence relation E=mc2. Let mx,my and mα be the masses of the parent nucleus x, the daughter nucleus y and α− particle respectively. Also the kinetic energy of α− particle just after the decay is E0. Assuming all motion of to be non-relativistic.
Which of the following is correct ?
View SolutionIn the β+ decay process , the following changes take place inside the nucleus
View SolutionIf .92U235 assumed to decay only by emitting two α-and one β-particles, the possible product of decays is
View SolutionA radioactive nucleus X decays to a nucleus Y with a decay constant λX=0.1s−1, Y further decays to a stable nucleus Z with a decay constant
λY=1/30s−1. Initially, there are only X nuclei and their number is N0=1020. Set up the rate equations for the populations of X, Y and Z. The population of Y nucleus as a function of time is given by NY(t)={N0λX/(λX−λY)}[exp(−λYt)−exp(−λXt)].
Find the time at which NY is maximum and determine the population X and Z at that instant.
View Solution
