Two projectiles are thrown simultaneously in the same plane from the same point. If their velocities are v1 and v2 at angles θ1 and θ2 respectively from the horizontal, then ansewer the following questions
The trajectory of particle 1 with respect to particle 2 wil be
a parabola
a straight linc
a vertical straight line
a horizontal straight line
The correct Answer is:B
To solve the problem of determining the trajectory of particle 1 with respect to particle 2, we will analyze the motion of both projectiles and their relative motion. Here’s a step-by-step solution:
Step 1: Resolve the velocities of both projectiles
For projectile 1, with initial velocity v1 at angle θ1:
- Horizontal component of velocity: v1x=v1cosθ1
- Vertical component of velocity: v1y=v1sinθ1
For projectile 2, with initial velocity v2 at angle θ2:
- Horizontal component of velocity: v2x=v2cosθ2
- Vertical component of velocity: v2y=v2sinθ2
Step 2: Write the equations of motion for both projectiles
The position of projectile 1 as a function of time t can be expressed as:
- x1(t)=v1xt=v1cosθ1⋅t
- y1(t)=v1yt−12gt2=v1sinθ1⋅t−12gt2
The position of projectile 2 can be expressed as:
- x2(t)=v2xt=v2cosθ2⋅t
- y2(t)=v2yt−12gt2=v2sinθ2⋅t−12gt2
Step 3: Determine the relative position of particle 1 with respect to particle 2
The relative position can be defined as:
- xrel(t)=x1(t)−x2(t)=(v1cosθ1−v2cosθ2)t
- yrel(t)=y1(t)−y2(t)=(v1sinθ1−v2sinθ2)t
Step 4: Analyze the relative motion
The relative motion can be expressed as:
- xrel(t)=(v1cosθ1−v2cosθ2)t
- yrel(t)=(v1sinθ1−v2sinθ2)t−12gt2
Step 5: Determine the nature of the trajectory
Since the acceleration due to gravity affects both projectiles equally (both have the same vertical acceleration of −g), the relative acceleration between the two projectiles is zero. This means that the relative velocity is constant over time.
Conclusion
Since the relative velocity is constant and the motion is linear, the trajectory of particle 1 with respect to particle 2 will be a straight line.
Final Answer
The trajectory of particle 1 with respect to particle 2 will be a straight line.
---
For projectile 1, with initial velocity v1 at angle θ1:
- Horizontal component of velocity: v1x=v1cosθ1
- Vertical component of velocity: v1y=v1sinθ1
- Horizontal component of velocity: v2x=v2cosθ2
- Vertical component of velocity: v2y=v2sinθ2
The position of projectile 1 as a function of time t can be expressed as:
- x1(t)=v1xt=v1cosθ1⋅t
- y1(t)=v1yt−12gt2=v1sinθ1⋅t−12gt2
- x2(t)=v2xt=v2cosθ2⋅t
- y2(t)=v2yt−12gt2=v2sinθ2⋅t−12gt2
The relative position can be defined as:
- xrel(t)=x1(t)−x2(t)=(v1cosθ1−v2cosθ2)t
- yrel(t)=y1(t)−y2(t)=(v1sinθ1−v2sinθ2)t
The relative motion can be expressed as:
- xrel(t)=(v1cosθ1−v2cosθ2)t
- yrel(t)=(v1sinθ1−v2sinθ2)t−12gt2
Since the acceleration due to gravity affects both projectiles equally (both have the same vertical acceleration of −g), the relative acceleration between the two projectiles is zero. This means that the relative velocity is constant over time.
Since the relative velocity is constant and the motion is linear, the trajectory of particle 1 with respect to particle 2 will be a straight line.
The trajectory of particle 1 with respect to particle 2 will be a straight line.
Topper's Solved these Questions
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (S-2)
15 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
- MOTION IN A PALNEBOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE
28 Videos
Similar Questions
Two particles are simultaneously projected in the same vertical plane from the same point with velocities u and v at angles αandβ with horizontal. Find the time that elapses when their velocities are parallel.
View SolutionTwo particles are projected simultaneously in the same vertical plane, with speed u1 and u2 at angle of projection θ1 and θ2 respectively with the horizontal. The path followed by one, as seen by other (as long as both are in flight), is
View Solution
Knowledge Check
Two particles are projected simultaneously in the same vertical plane from the same point, with different speeds u1 and u2, making angles θ1 and θ2 respectively with the horizontal, such that u1cosθ1=u2cosθ2. The path followed by one, as seen by the other (as long as both are in flight), is
Aa vertical lineBa parabolaCa hyperbolaDa straight line making a constant angle (≠90∘) with horizontal
Two particles of equal mass 'm' are projected from the ground with speed v1 and v2 at angles θ1 and θ2 at the same times as shown in figure. The centre of mass of the two particles.

View SolutionTwo bodies are thrown with the same initial velocity at angles θ and (90∘−θ) respectively with the horizontal, then their maximum height are in the ratio
View SolutionTwo particles are projected at the same instant with speeds v1 and v2 making angles α and β with the horizontal as shown in the figure. Nelecting air resistance, the trajectory of the mass center the two particles
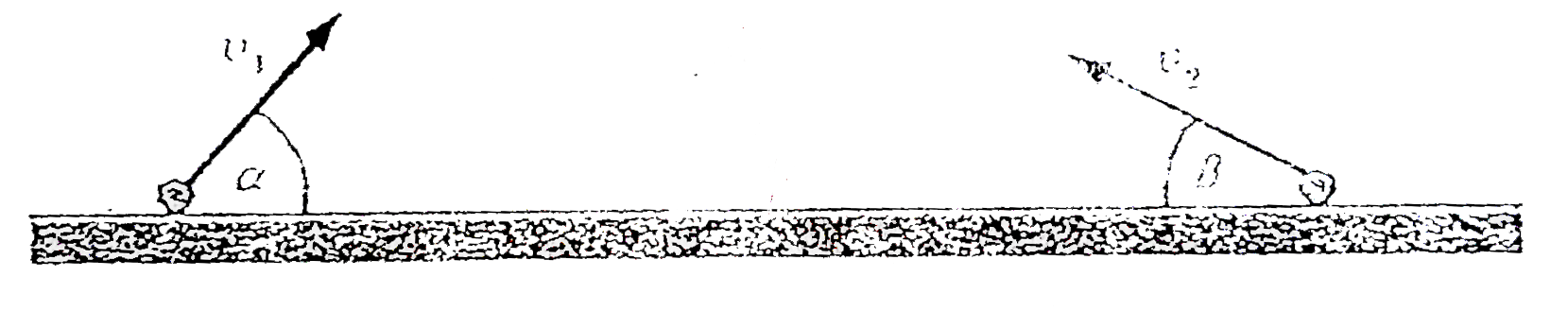
View SolutionTwo particles P & Q are projected simultaneously from a point O on a level ground in the same vertical plane with the same speed in directions making angle of 30∘ and 60∘ respectively with the horizontal.
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
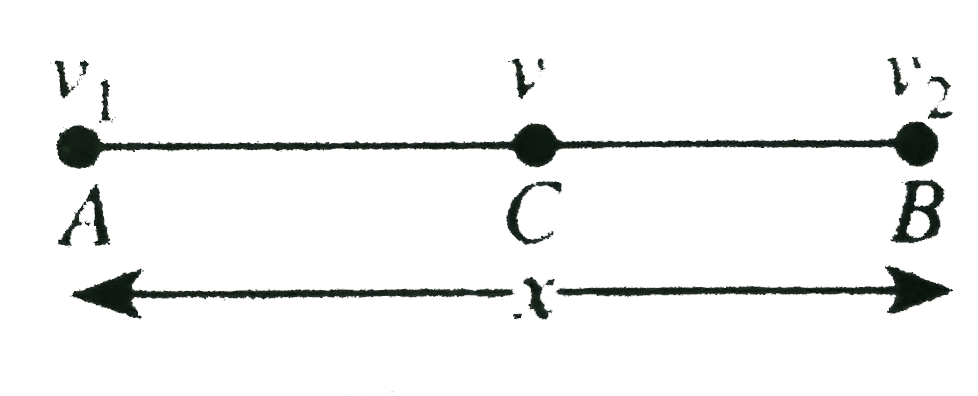 .
.
View SolutionTwo particles are projected from the same point with the same speed at different angles θ1 & θ2 to the horizontal. They have the same range. Their times of flight are t1 & t2 respectily
View SolutionTwo particles are projected from the same point with the same speed at different angles θ1 & θ2 to the horizontal. They have the same range. Their times of flight are t1 & t2 respectfully
View Solution