The speed of a particle moving in a circle slows down at a rate of 3m/sec2. At some instant the magnitude of the total acceleration is 5msec2 and the particle's speed is 12 m/sec. The radius of circle will be :
12 m
24 m
36 m
48 m
The correct Answer is:C
To find the radius of the circle in which a particle is moving, we can follow these steps:
Step 1: Identify the given values
- The tangential acceleration, at=−3m/s2 (negative because the speed is decreasing).
- The total acceleration, a=5m/s2.
- The speed of the particle, v=12m/s.
Step 2: Use the relationship between total acceleration, tangential acceleration, and centripetal acceleration
The total acceleration a can be expressed as:
a=√a2t+a2c
where ac is the centripetal acceleration.
Step 3: Substitute the known values into the equation
We know:
at=−3m/s2anda=5m/s2
Thus, substituting these values gives:
5=√(−3)2+a2c
This simplifies to:
5=√9+a2c
Step 4: Square both sides to eliminate the square root
Squaring both sides results in:
25=9+a2c
Step 5: Solve for centripetal acceleration ac
Rearranging the equation gives:
a2c=25−9=16
Taking the square root:
ac=4m/s2
Step 6: Relate centripetal acceleration to radius
Centripetal acceleration is given by the formula:
ac=v2r
Substituting the known values:
4=122r
This simplifies to:
4=144r
Step 7: Solve for the radius r
Rearranging gives:
r=1444=36m
Final Answer
The radius of the circle is r=36m.
---
- The tangential acceleration, at=−3m/s2 (negative because the speed is decreasing).
- The total acceleration, a=5m/s2.
- The speed of the particle, v=12m/s.
The total acceleration a can be expressed as:
a=√a2t+a2c
where ac is the centripetal acceleration.
We know:
at=−3m/s2anda=5m/s2
Thus, substituting these values gives:
5=√(−3)2+a2c
This simplifies to:
5=√9+a2c
Squaring both sides results in:
25=9+a2c
Rearranging the equation gives:
a2c=25−9=16
Taking the square root:
ac=4m/s2
Centripetal acceleration is given by the formula:
ac=v2r
Substituting the known values:
4=122r
This simplifies to:
4=144r
Rearranging gives:
r=1444=36m
The radius of the circle is r=36m.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (COLLISION AND CENTRE OF MASS )
12 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (ROTATIONAL MOTION)
72 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (WORK POWER & ENERGY)
34 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Video
Similar Questions
Change a speed of 12 m/sec into km/hr.
View SolutionChange a speed of 12 m/sec into km/hr.
View SolutionCentripetal acceleration of a particle of mass m moving in a circle of radius r is 4/r2. What is the angular momentum of the particle ?
View SolutionA particle is moving on a circle of radius A .At an instant its speed and tangential acceleration are B and C respectively.Total acceleration of the particle at that instant is
View SolutionThe speed of a particle moving in a circle of radius r=2m varies with time t as v=t2, where t is in second and v in m/s. Find the radial, tangential and net acceleration at t=2s.
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
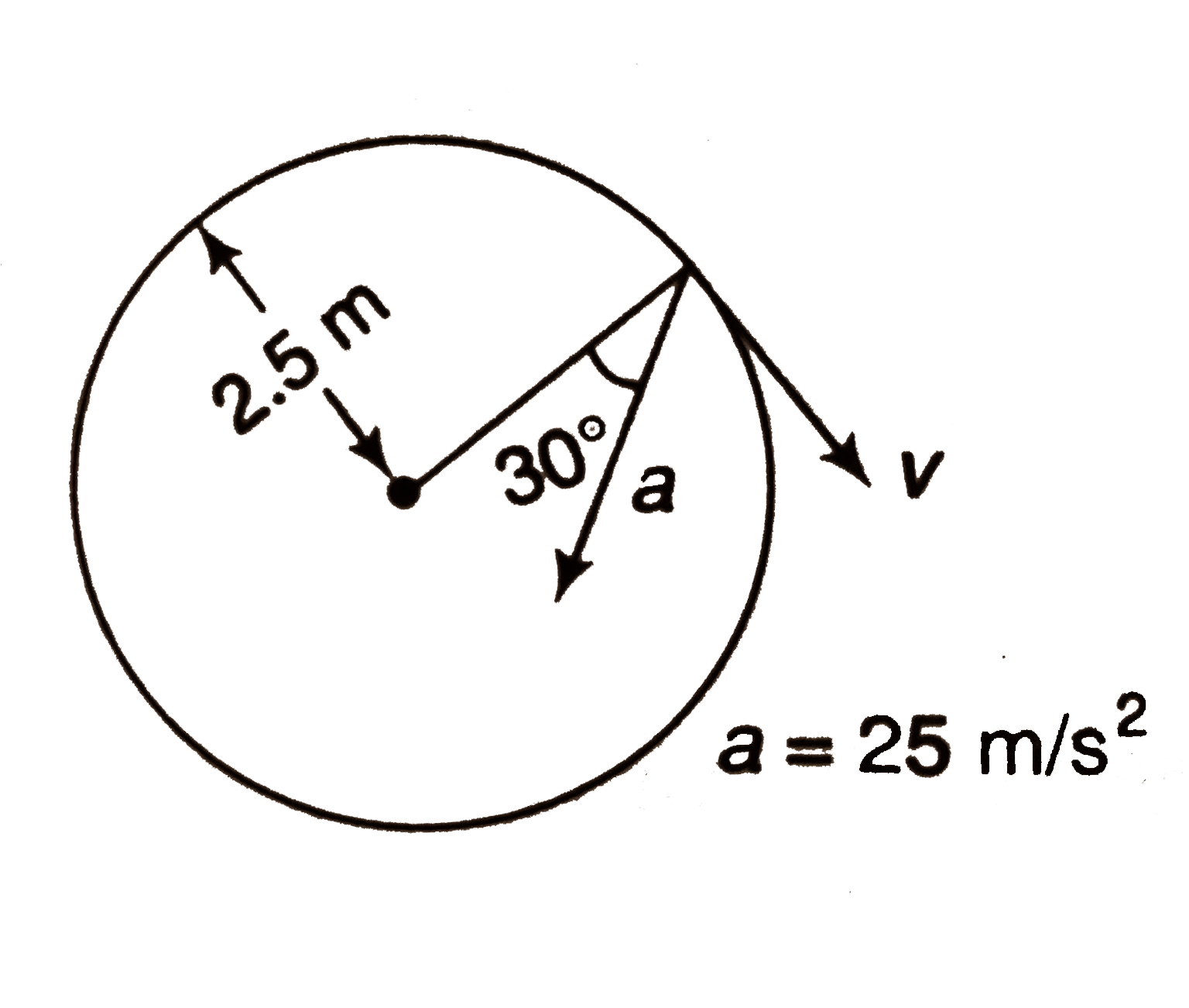
View SolutionA particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
View SolutionFind the total acceleration of a particle, moving in a circular track of radius 2 m, with constant angular acceleration of 1 rad/sec2 at time t = 2 seconds from the center
View SolutionA 2 mg sand particle is blown with a speed of 50m/sec what is the its de Broglie's wavelength ?
View SolutionIf a particle moves in a circle of radius of 4m at speed given by v=2t where v is in m/s and t in sec.At t=4s ,net accleration of particle will be:
View Solution
