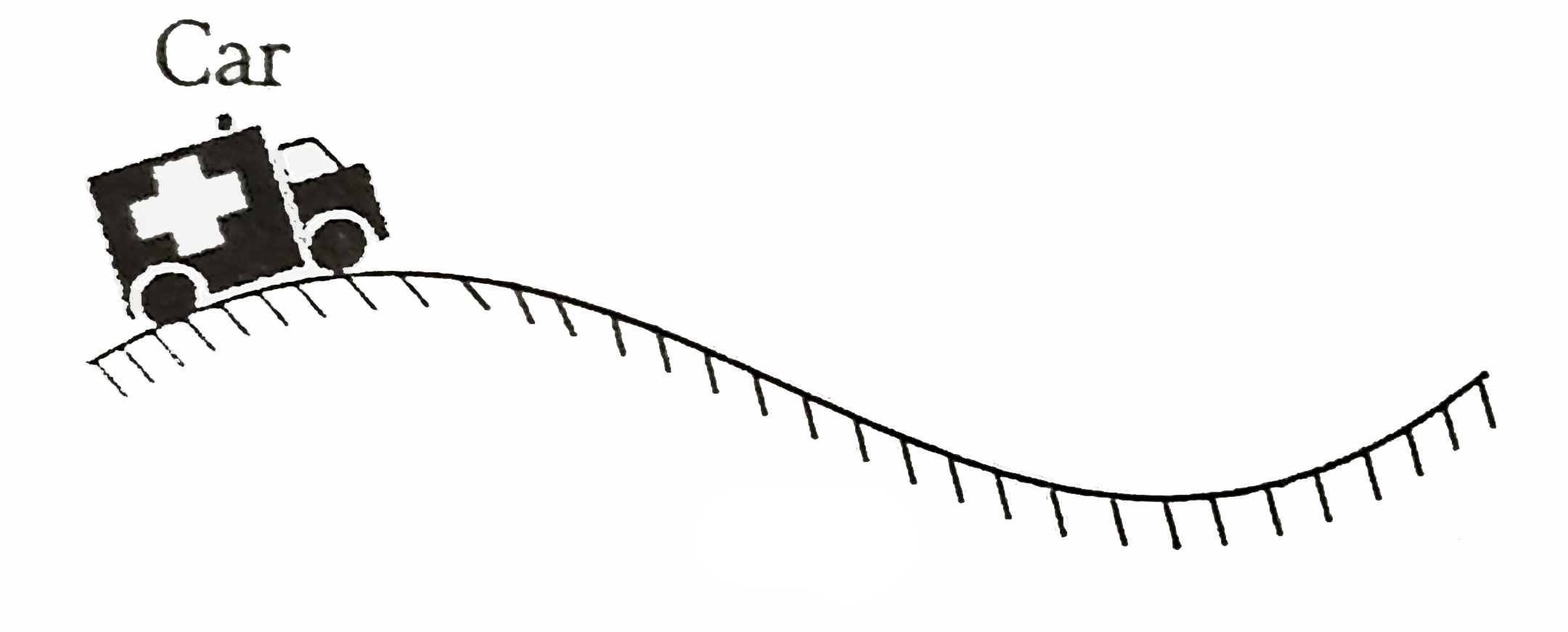
A vehicle is moving on a track as shown in Fig. The normal reaction between tyre's and road is :
maximum at A
maximum at B
maximum at C
same at A, B and C
The correct Answer is:B
To solve the problem of determining the normal reaction between the tires and the road as the vehicle moves along the track, we can follow these steps:
Step 1: Analyze the Vehicle's Motion
The vehicle is moving along a track that has inclines and declines. We need to consider the forces acting on the vehicle at different points on the track, particularly at points B and C where the incline is more pronounced.
Hint: Identify the points of interest on the track where the incline changes significantly.
Step 2: Identify Forces Acting on the Vehicle
At any point on the track, the forces acting on the vehicle include:
- The weight of the vehicle (acting downwards, W=mg)
- The normal force (acting perpendicular to the road surface)
Hint: Remember that the normal force adjusts based on the incline of the road and the weight of the vehicle.
Step 3: Analyze the Normal Force at Point B
At point B, where the incline is steep, the normal force will be affected by both the weight of the vehicle and the centripetal force required for the vehicle to navigate the curve. The vehicle experiences maximum stress here due to the steep incline.
Hint: Consider how the angle of incline affects the distribution of forces acting on the vehicle.
Step 4: Calculate the Normal Force
The normal force can be calculated using the equation:
N=mgcos(θ)+mv2r
where:
- N is the normal force,
- m is the mass of the vehicle,
- g is the acceleration due to gravity,
- θ is the angle of incline at point B,
- v is the velocity of the vehicle,
- r is the radius of the curve.
At point B, the vehicle experiences the maximum normal force due to the combination of gravitational force and the centripetal force.
Hint: Make sure to account for both gravitational and centripetal forces when calculating the normal force.
Step 5: Conclusion
After analyzing the forces and calculating the normal force at point B, we conclude that the normal reaction between the tires and the road is maximum at this point due to the steep incline and the vehicle's motion.
Final Answer: The normal reaction between the tires and the road is maximum at point B.
The vehicle is moving along a track that has inclines and declines. We need to consider the forces acting on the vehicle at different points on the track, particularly at points B and C where the incline is more pronounced.
At any point on the track, the forces acting on the vehicle include:
- The weight of the vehicle (acting downwards, W=mg)
- The normal force (acting perpendicular to the road surface)
At point B, where the incline is steep, the normal force will be affected by both the weight of the vehicle and the centripetal force required for the vehicle to navigate the curve. The vehicle experiences maximum stress here due to the steep incline.
The normal force can be calculated using the equation:
N=mgcos(θ)+mv2r
where:
- N is the normal force,
- m is the mass of the vehicle,
- g is the acceleration due to gravity,
- θ is the angle of incline at point B,
- v is the velocity of the vehicle,
- r is the radius of the curve.
After analyzing the forces and calculating the normal force at point B, we conclude that the normal reaction between the tires and the road is maximum at this point due to the steep incline and the vehicle's motion.
Topper's Solved these Questions
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (COLLISION AND CENTRE OF MASS )
12 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (ROTATIONAL MOTION)
72 Videos
- RACEBOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (WORK POWER & ENERGY)
34 Videos
- NEWTONS LAWS OF MOTIONBOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III
28 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
Similar Questions
A vehicle is moving on a track with constant speed as shown in figure. The apparent weight of the vehicle is

View SolutionAssertion A car moves along a road with uniform speed. The path of car lies in vertical plane and shown in fighre. The radius of curvature (R) of the path is same everywhere. If the car does not loose contact with road at the highest point, it can travel the shown path without loosing contact with road anywhere else.
Reason For car to loose contact with road, the normal reaction between car and road should be zero.
View Solution
Knowledge Check
A small roller coaster starts at point A with a speed u on a curved track as shown in the figure
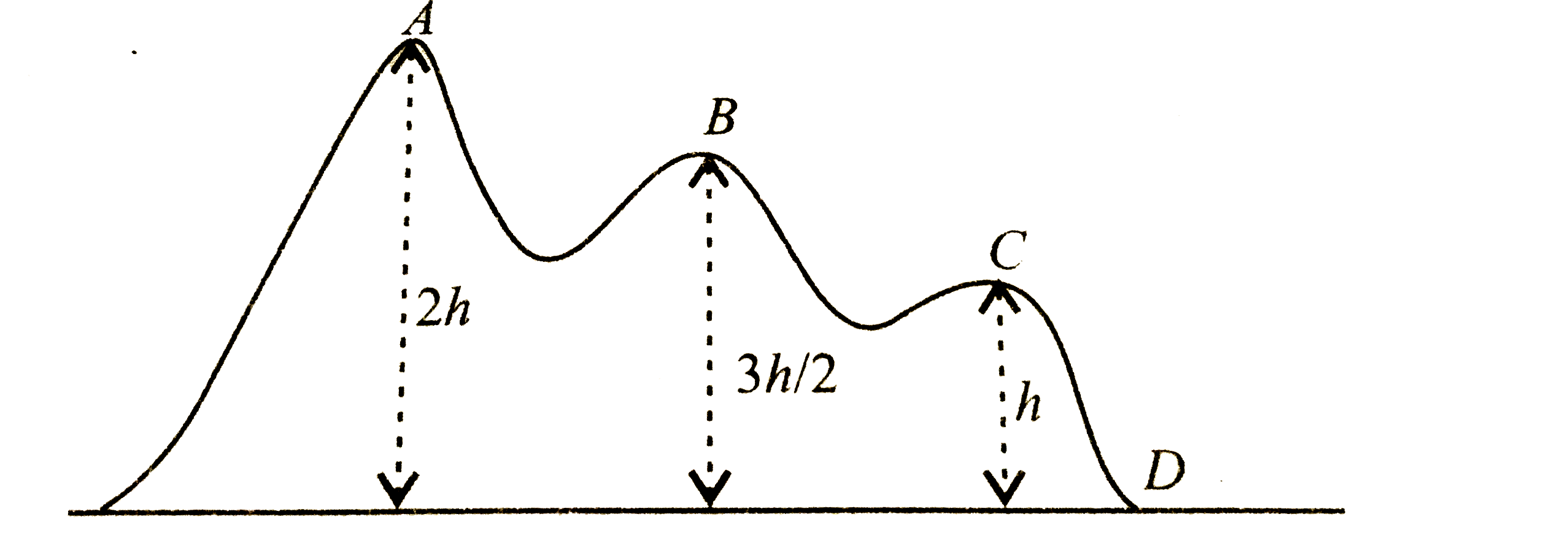
The friction between the roller coaster and the track is negligible and it always remains in contact with the track. The speed of roller coaster at point D on the track will beA(u2+gh)1/2B(u2+2gh)1/2C(u2+4gh)1/2Du
A vehicle is moving on a rough road in a straight line with uniform velocity
View SolutionA vehicle of mass 500 kg is moving with a velocity of 15ms−1. It is brought to rest by a retarding force. Find the distance moved by the vehicle before coming to rest, if the sliding friction between the tyres and the road is 3000N.
View SolutionFor the circuit shown in fig. the equivalent resistance between A and C is

View SolutionA vehicle is travelling along unbanked curved path. If the friction between the road and tyres suddenly disappears then the vehicle
View SolutionA car moves at a constant speed on a road as shown in figure. The normal force by the road on the car is NAandNB when it is at the points A and B respectively
 .
.
View SolutionWhat is the minimum stopping distance for a vehicle of mass m moving with speed v along a level road. If the coefficient of friction between the tyres and the road is μ.
View SolutionA car is taking turn on a circular path of radius R. If the coefficient of friction between the tyres and road is μ, the maximum velocity for no slipping is
View Solution
