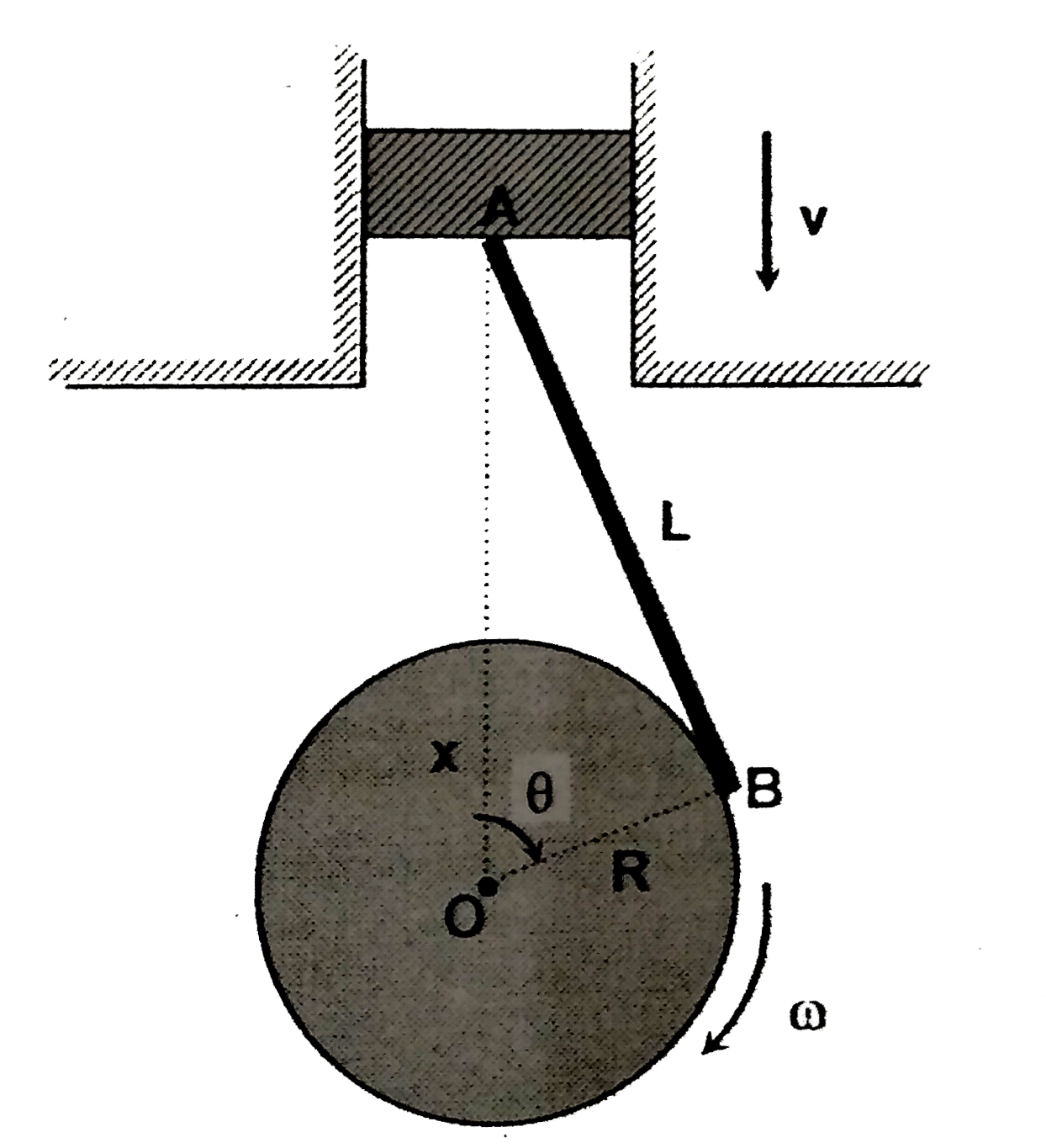
Find velocity of piston A in the given situation if angular velocity of wheel of radius R is $ω$ (constant) in the clockwise sense (O is fixed point)

$\frac{x R ω sin θ}{R cos θ + x}$

$\frac{x^{2} ω sin θ}{R cos θ - x}$

$\frac{x R ω sin θ}{R cos θ - x}$

$\frac{R^{2} ω sin θ}{R cos θ - x}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

$cos θ = \frac{x^{2} + R^{2} - L^{2}}{2 x R} \Rightarrow 2 x R cos θ = x^{2} + R^{2} + L^{2}$
$2 R {- x sin θ (\frac{d θ}{d t}) + cos θ (\frac{d x}{d t})} = 2 x \frac{d x}{d t}$
$x v = R v cos θ - R x ω sin θ$
$\Rightarrow v = (\frac{x R ω sin θ}{R cos θ - x})$

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Similar Questions

A rod is rotating with angular velocity $ω$ about axis AB. Find $cos θ$ .

View Solution
two cylindrical hollow drums of radii R and 2 R, and of a common height h , are rotating with angular velocities $ω$ (anti- clockwise ) and $ω$ (clockwise ) , respectively , their axes , fixed are parallel and in a horizontal plane separated by by 3R + $δ$ , they are now brought in contact $(δ \to 0)$
(A) show frictional forces just after contact .
(B) identify forces and torque external to the system just after contact ,
(c) what would be the ratio of final of final angular velocities when friction ceases ?
View Solution
A rod of mass $m$ and length $l$ is rotating about a fixed point in the ceiling with an angular velocity $ω$ as shown in the figure. The rod maintains a constant angle $θ$ with the vertical.
What is the rate of change of angular momentum of the rod ?

View Solution
A rod of mass $m$ and length $l$ is rotating about a fixed point in the ceiling with an angular velocity $ω$ as shown in the figure. The rod maintains a constant angle $θ$ with the vertical.
What will be the horizontal component of angular momentum of the rod about the point of suspension in terms of $m$ , $ω$ , $l$ and $θ$ .

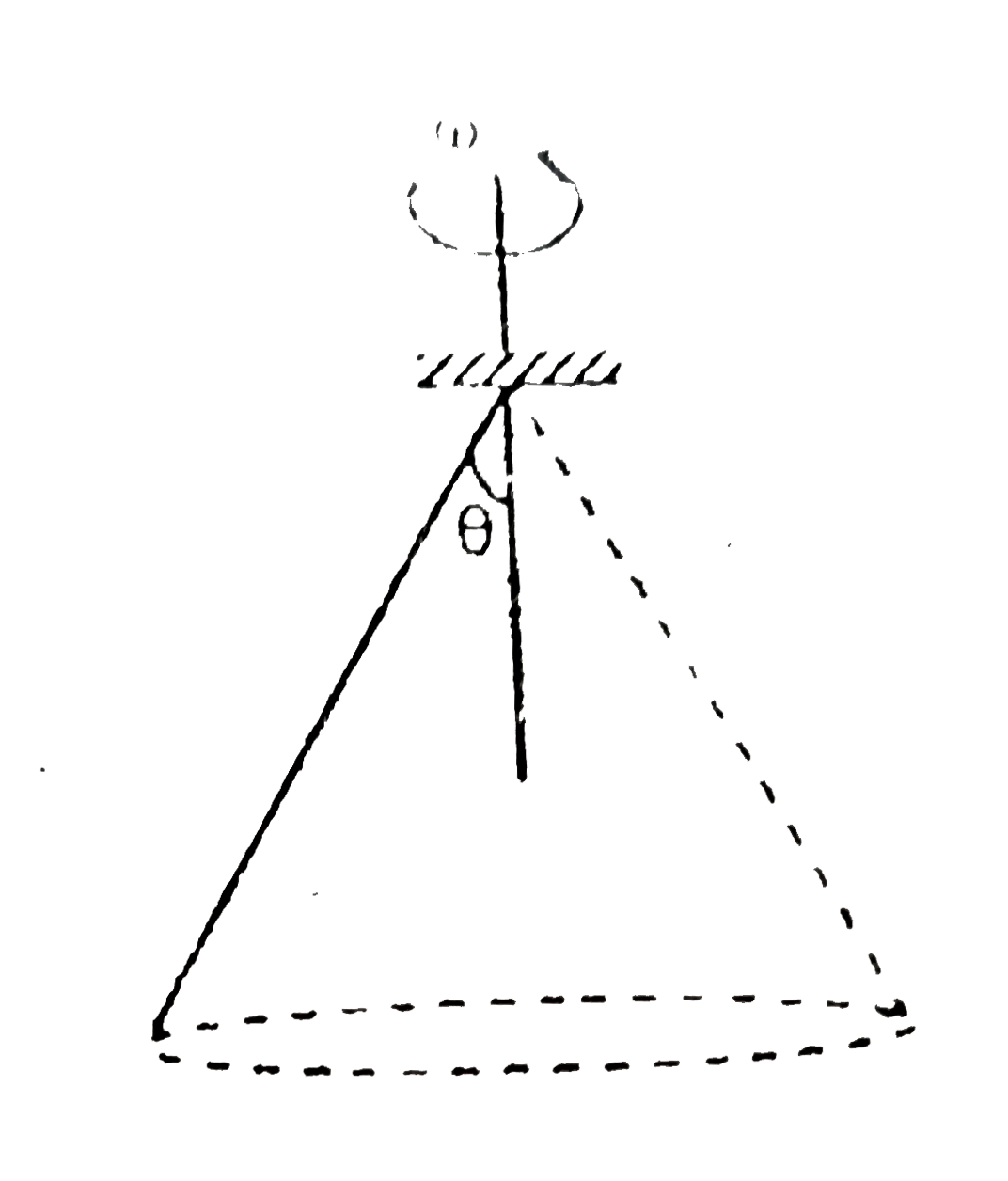
View Solution
A rod of mass $m$ and length $l$ is rotating about a fixed point in the ceiling with an angular velocity $ω$ as shown in the figure. The rod maintains a constant angle $θ$ with the vertical.
What angle will the rod make with the vertical

View Solution
Two cylindrical hollow drums of radii $R and 2 R$ , and of a commom height h, are rotating with angular velocities $ω$ (anti-clockwise) and $ω$ (clockwise), respectively. Their axes, fixed are parallel and in a horizontal plane separated by $(3 R + δ)$ . They are now brought in contact $(δ \to 0)$ .
(a) Show the frictional forces just after contact.
(b) Identify forces and torque external to the system just after contact.
(c ) What would be the ratio of final angular velocities when friction ceases ?
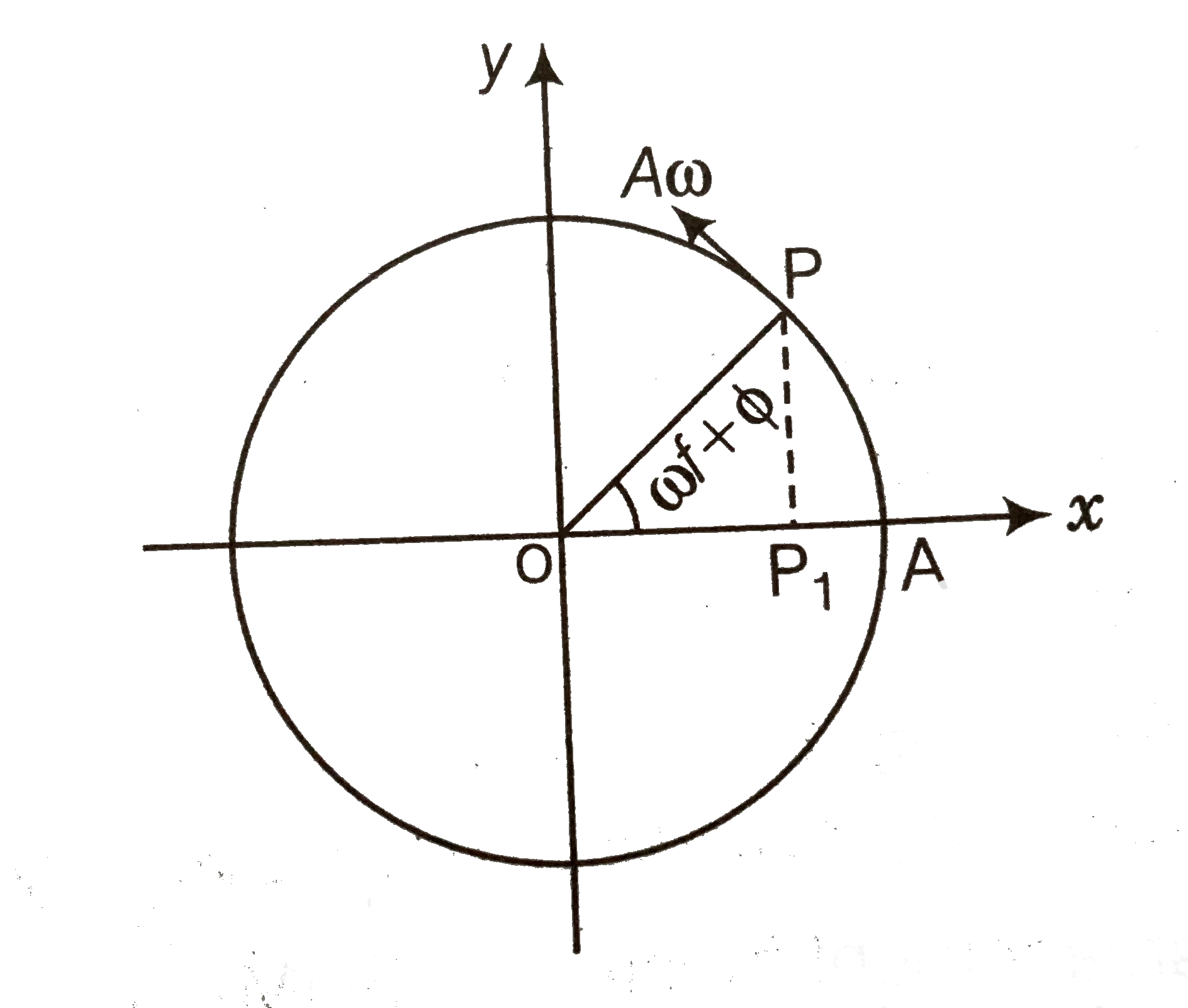
View Solution
In figure, what be the sign of the velocity of the point P', which is the projection of the velocity of the reference particle P.P is moving in a circle of radius R in anti-clockwise direction.

View Solution
The linear velocity of a rotating body is given by $\to v = \to ω \times \to r$ , where $\to ω$ is the angular velocity and $\to r$ is the radius vector. The angular velocity of a body is $\to ω = ˆ i - 2 ˆ j + 2 ˆ k$ and the radius vector $\to r = 4 ˆ j - 3 ˆ k$ , then $| \to v |$ is
View Solution
A sphere is moving at some instant with horizontal velocity $v_{0}$ in right and angular velocity $ω$ in anti clockwise sense. If $| v_{0} | = | ω R |$ , the instantaneous centre of rotation is
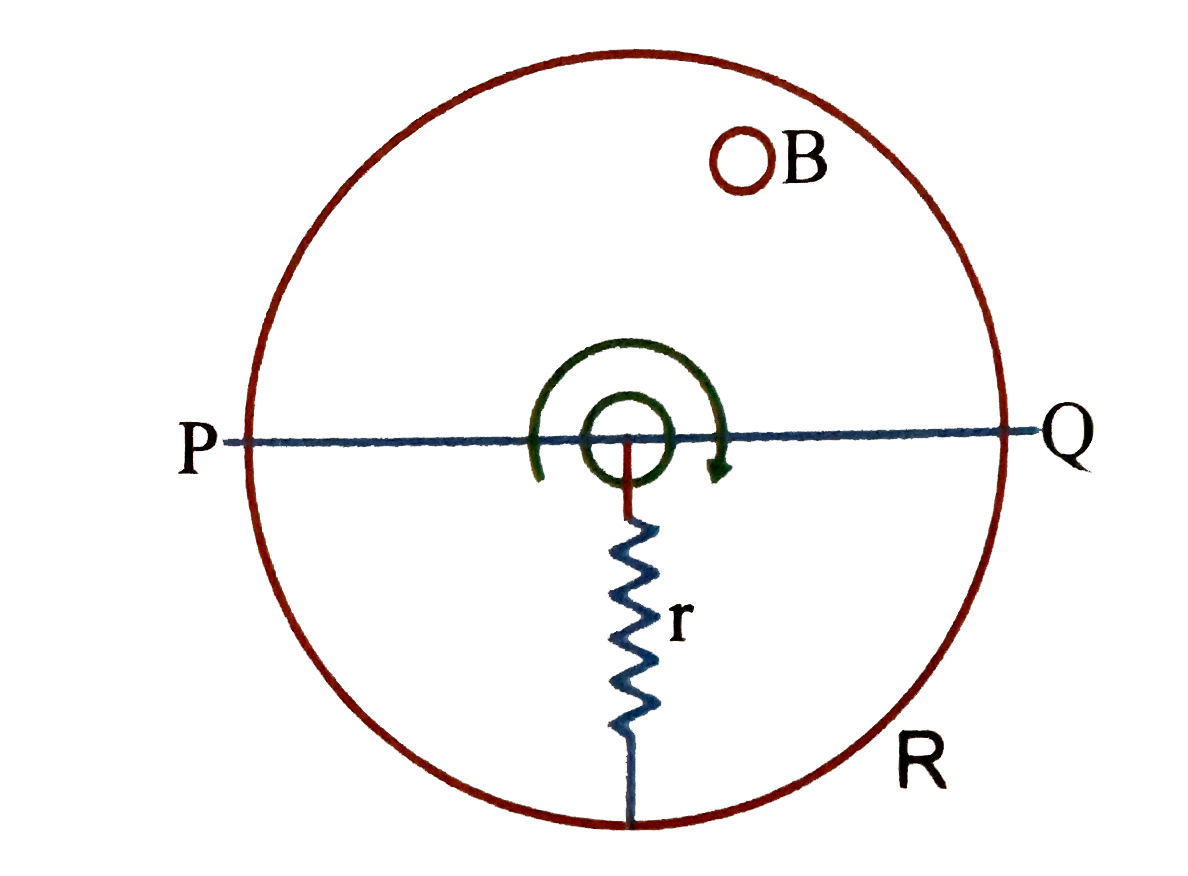
View Solution
In the figure shown $' R'$ is a fixed conducting fixed ring of negligible resistance and radius $' a'$ $P Q$ is a uniform rod of resistance $r$ . It is hinged at the centre of the ring and rotated about this point in clockwise direction with a uniform angular velocity $w$ . These is a uniform magnetic filled of strength $' B'$ ponting inwards. $' r'$ is a stationary resistance

View Solution

Find velocity of piston A in the given situation if angular velocity of wheel of radius R is ω (constant) in the clockwise sense (O is fixed point)

The correct Answer is:C

cosθ=x2+R2−L22xR⇒2xRcosθ=x2+R2+L2 2R{−xsinθ(dθdt)+cosθ(dxdt)}=2xdxdt xv=Rvcosθ−Rxωsinθ ⇒v=(xRωsinθRcosθ−x)

Similar Questions

Recommended Questions

Find velocity of piston A in the given situation if angular velocity of wheel of radius R is $ω$ (constant) in the clockwise sense (O is fixed point)

$cos θ = \frac{x^{2} + R^{2} - L^{2}}{2 x R} \Rightarrow 2 x R cos θ = x^{2} + R^{2} + L^{2}$
$2 R {- x sin θ (\frac{d θ}{d t}) + cos θ (\frac{d x}{d t})} = 2 x \frac{d x}{d t}$
$x v = R v cos θ - R x ω sin θ$
$\Rightarrow v = (\frac{x R ω sin θ}{R cos θ - x})$