Magnets and are geometrically similar but the magnetic moment of is twice that of . If and be the time periods of the oscillation when their like poles and unlike poles are kept togather respectively, then will be
The correct Answer is:C
To solve the problem, we need to find the ratio of the time periods T1 and T2 when magnets A and B are placed with like poles and unlike poles together, respectively.
1. Understanding the Time Periods:
- The time period T1 when like poles are together is given by:
T1=2π√I1+I2M1+M2⋅1BH
- The time period T2 when unlike poles are together is given by:
T2=2π√I1+I2M1−M2⋅1BH
2. Taking the Ratio T1T2:
- We can write the ratio of the time periods as follows:
T1T2=2π√I1+I2M1+M22π√I1+I2M1−M2
- The 2π and √I1+I2 terms cancel out:
T1T2=√M1−M2M1+M2
3. Substituting the Magnetic Moments:
- Given that the magnetic moment of A (M1) is twice that of B (M2), we can express M1 and M2 as:
M1=2MandM2=M
- Now substituting these values into the ratio:
T1T2=√(2M)−M(2M)+M=√M3M=√13=1√3
4. Final Result:
- Therefore, the ratio T1T2 is:
T1T2=1√3
Conclusion:
The final answer is T1T2=1√3.
- The time period T1 when like poles are together is given by:
T1=2π√I1+I2M1+M2⋅1BH
- The time period T2 when unlike poles are together is given by:
T2=2π√I1+I2M1−M2⋅1BH
- We can write the ratio of the time periods as follows:
T1T2=2π√I1+I2M1+M22π√I1+I2M1−M2
- The 2π and √I1+I2 terms cancel out:
T1T2=√M1−M2M1+M2
- Given that the magnetic moment of A (M1) is twice that of B (M2), we can express M1 and M2 as:
M1=2MandM2=M
- Now substituting these values into the ratio:
T1T2=√(2M)−M(2M)+M=√M3M=√13=1√3
- Therefore, the ratio T1T2 is:
T1T2=1√3
The final answer is T1T2=1√3.
Similar Questions
If T1 and T2 are the time-periods of oscillation of a simple pendulum on the surface of earth (of radius R) and at a depth d, the d is equal to
View SolutionIf M is the magnetic moment, B is the magnetic induction and T is the time then MBT2 has the dimensions of
View SolutionTwo similar magnets of magnetic moments M1 and M2 are taken and vibrated in vibration magnetometer with their like pole together and unlike pole together . If ratio of M1M2=53 , then ratio of the period is
View SolutionThree arrangements of spring - mass system are shown in figures (A), (B) and ( C). If T1, T2 and T3 represent the respective periods of oscillation, then correct relation is
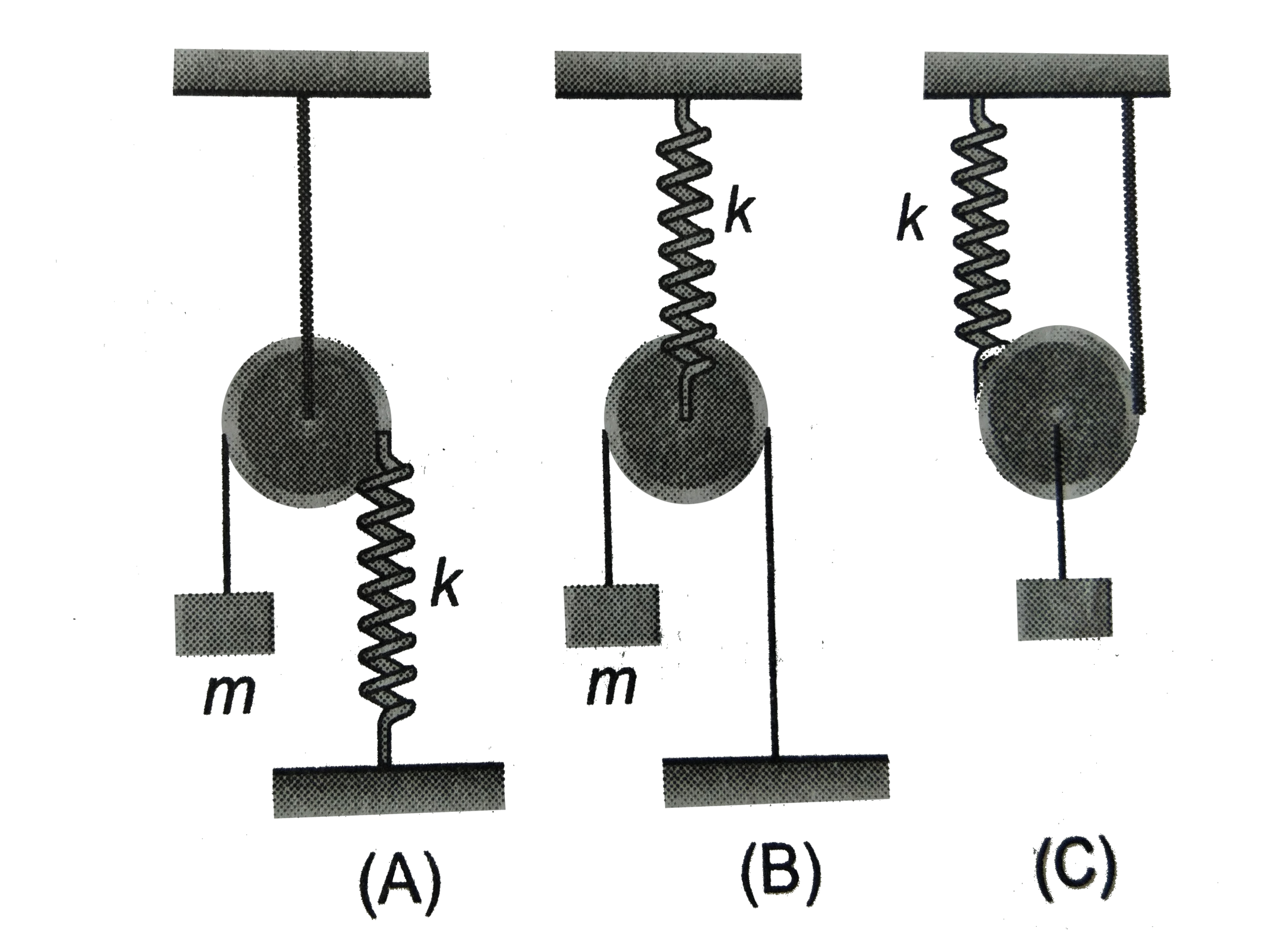
View SolutionTwo stones are projected from level ground. Trajectory of two stones are shown in figure. Both stones have same maximum heights above level ground as shown. Let T1 and T2 be their time of flights and u1 and u2 be their speeds of projection respectively (neglect air resistance). then
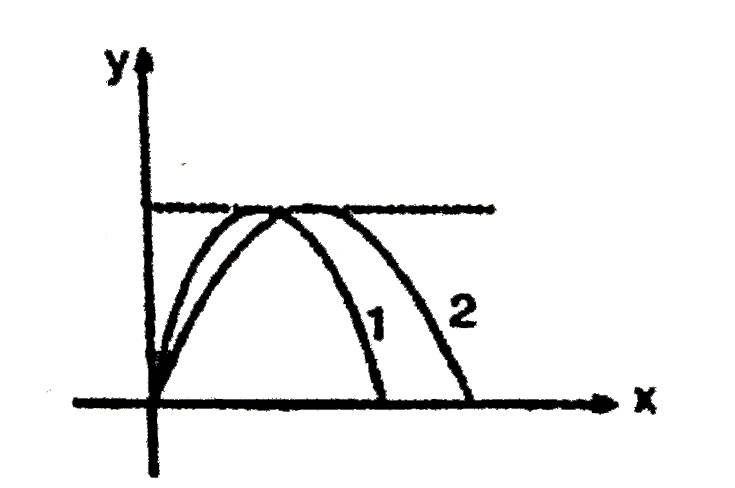
View SolutionThe period of oscillations of a magnet is 2 sec. When it is remagnetised so that the pole strength is 4 times its period will be
View SolutionThe period of oscillations of a magnet is 2 sec. When it is remagnetised so that the pole strength is 4 times its period will be
View SolutionA, B, C are respectively the points (1,2), (4, 2), (4, 5). If T1,T2 are the points of trisection of the line segment BC, the area of the Triangle AT1T2 is
View Solutioncompounds having similar geometry have different magnetic moment(T/F)
View SolutionTwo bar magnets having same geometry with magnetic moments M and 2M, are firstly placed in such a way what their similar poles are same side then its time period of oscillation is T1. Now the polarity of one of the magnet is reversed then time period of oscillation will be:-
View Solution
