Masses m,2m, 3m, 4m,………,12m are kept on the peripheri of a clock having a circular dial. The masses are kept such that m is kept at 1, 2, 3m at 3………12m at 12 . →g is the gravitational field intensity at the centre of dial.
At 9:30 the hour hand is in the direction of →g
At 3:30 the hour hand is in the direction of →g
there will be no component of →g along hour hand at 6:30
There will be no component of →g along hour hand at 12:30
To solve the problem of determining the net gravitational field intensity at the center of a clock with masses placed at each hour mark, we can follow these steps:
Step 1: Identify the Masses and Their Positions
The masses placed on the clock are:
- At 1: m
- At 2: 2m
- At 3: 3m
- At 4: 4m
- At 5: 5m
- At 6: 6m
- At 7: 7m
- At 8: 8m
- At 9: 9m
- At 10: 10m
- At 11: 11m
- At 12: 12m
Step 2: Calculate the Gravitational Field Contribution from Each Mass
The gravitational field intensity →g at the center due to a mass m at a distance r is given by:
g=Gmr2
Since all masses are at the same distance r from the center, we can consider the contributions from each mass in terms of their components along the x-axis and y-axis.
Step 3: Break Down Each Mass's Contribution into Components
Each mass contributes to the gravitational field in both the x and y directions. The angle for each hour mark can be calculated as follows:
- 1 o'clock: 0∘
- 2 o'clock: 30∘
- 3 o'clock: 60∘
- 4 o'clock: 90∘
- 5 o'clock: 120∘
- 6 o'clock: 150∘
- 7 o'clock: 180∘
- 8 o'clock: 210∘
- 9 o'clock: 240∘
- 10 o'clock: 270∘
- 11 o'clock: 300∘
- 12 o'clock: 330∘
The components of the gravitational field from each mass can be calculated using:
- gx=gcos(θ)
- gy=gsin(θ)
Step 4: Sum Up the Components
We need to sum the contributions from all the masses in both the x and y directions:
Gx=∑gx=∑(G⋅massr2cos(θ))
Gy=∑gy=∑(G⋅massr2sin(θ))
Step 5: Calculate the Net Gravitational Field
The net gravitational field intensity G at the center can be found using:
G=√G2x+G2y
The direction can be found using:
tan(ϕ)=GyGx
Step 6: Analyze the Direction of the Gravitational Field
From the calculated components, determine the angle ϕ to understand the direction of the net gravitational field.
Step 7: Answer the Options
Based on the direction of the gravitational field, analyze the given options regarding the position of the hour hand and the direction of →g.
The masses placed on the clock are:
- At 1: m
- At 2: 2m
- At 3: 3m
- At 4: 4m
- At 5: 5m
- At 6: 6m
- At 7: 7m
- At 8: 8m
- At 9: 9m
- At 10: 10m
- At 11: 11m
- At 12: 12m
The gravitational field intensity →g at the center due to a mass m at a distance r is given by:
g=Gmr2
Since all masses are at the same distance r from the center, we can consider the contributions from each mass in terms of their components along the x-axis and y-axis.
Each mass contributes to the gravitational field in both the x and y directions. The angle for each hour mark can be calculated as follows:
- 1 o'clock: 0∘
- 2 o'clock: 30∘
- 3 o'clock: 60∘
- 4 o'clock: 90∘
- 5 o'clock: 120∘
- 6 o'clock: 150∘
- 7 o'clock: 180∘
- 8 o'clock: 210∘
- 9 o'clock: 240∘
- 10 o'clock: 270∘
- 11 o'clock: 300∘
- 12 o'clock: 330∘
- gx=gcos(θ)
- gy=gsin(θ)
We need to sum the contributions from all the masses in both the x and y directions:
Gx=∑gx=∑(G⋅massr2cos(θ))
Gy=∑gy=∑(G⋅massr2sin(θ))
The net gravitational field intensity G at the center can be found using:
G=√G2x+G2y
The direction can be found using:
tan(ϕ)=GyGx
From the calculated components, determine the angle ϕ to understand the direction of the net gravitational field.
Based on the direction of the gravitational field, analyze the given options regarding the position of the hour hand and the direction of →g.
Similar Questions
Masses of 1kg each are placed 1m,2m,4m,8m, ... from a point P. The gravitational field intensity at P due to these masses is
View SolutionThree particles each of mass m are kept at vertices of an equilateral triangle of side L. The gravitational field at centre due to these particle is
View SolutionTwo bodies of mass M and 4M kept at a distance y apart. Where should a small particle of mass m be placed from M so that the net gravitational force on it is zero
View SolutionA particle of mass m is kept on the axis of a fixed circular ring of mass M and radius R at a distance x from the centre of the ring. Find the maximum gravitational force between the ring and the particle.
View SolutionIf four different masses m1,m2,m3 and m4 are placed at the four corners of a square of side a, the resultant gravitational force on a mass m kept at the centre is
View SolutionFour particles of masses m, 2m, 3m & 4m respectively are placed on the periphery of a circle of radius R as shown in the figure then CM of the system contains the particle is at
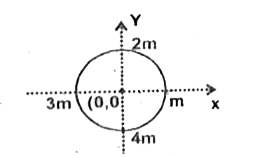 View Solution
View SolutionA solid sphere of radius a and mass m is surrounded by cocentric spherical shell of thickness 2a and mass 2m the gravitational field at a distance 3a from their centres is
View SolutionTwo particles having masses m and 4m are separated by distance l. The distance of the centre of mass from m is x1andx2 is the distance of point at which gravitational field intensity is zero. Find the value of x1x2
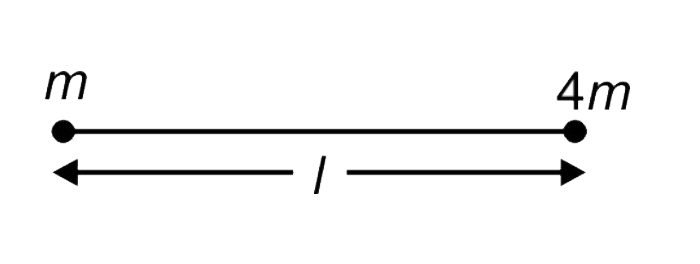 View Solution
View SolutionFour particles having masses, m, 2m, 3m, and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.
View SolutionFour particles having masses, m, 2m, 3m, and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.
View Solution

