In the figure shown a massless spring of stiffness k and natural length l0 is rigidly attached to a block of mass m and is in vertical position. A wooden ball of mass m is released from rest to fall under gravity. Having fallen a height h the ball strikes the spring and gets stuck up in the spring at the top. What should be the minimum value of h so that the lower block will just lose contact with the ground later on ? Assume that l0>>4mgk. Neglect any loss of energy. (Given k=mg/2)

The minimum force needed to lift the lower block is equal to its weight. During upward motion the spring will get elongated. If elongation in the spring for just lifting the block is x0 then
kx0=mg
⇒x0=mgk……(i)
From COE
mg(l0+h)=mg(l0+x0)+12kx20
⇒mgh=mgx0+12kx20⇒mgh=(mg)2k+12m2g2k⇒h=3mg2k
During down ward motion, suppose maximum compression in the spring is x
From COE
mg(l0+h)=mg(l0−x)+12kx2
⇒mgh=−mgx+12kx2⇒mg3mg2k=−mgx+12kx2
⇒3(mg)2=−2mgkx+k2x2
⇒k2x2−2mgkx−3(mg)2=0
⇒x=2mgk±√4(mgk)2+12k2(mg)22k2=2mgk±4mgk2k2⇒x=3mgk
Similar Questions
A block of mass m is relesed from rest from a height h onto a smooth surface of mass M fitted with an ideal spring of stiffness k. find the maximum compression in the spring.

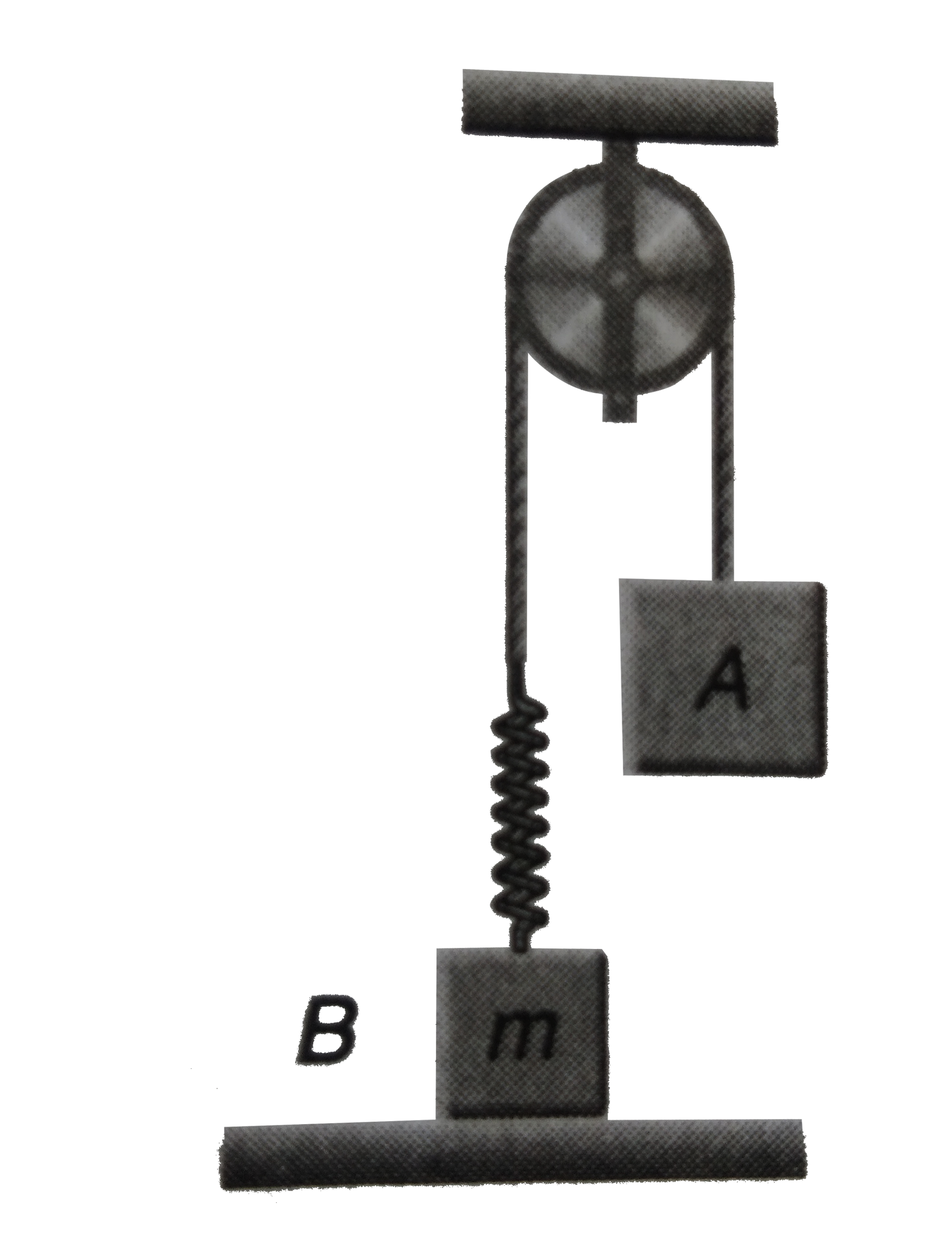
View SolutionIn the figure, block A is released from rest when the spring is its natural length for the block B of mass m to leave contact with the ground at some stage what should be the minimum mass of block A?
 .
.
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

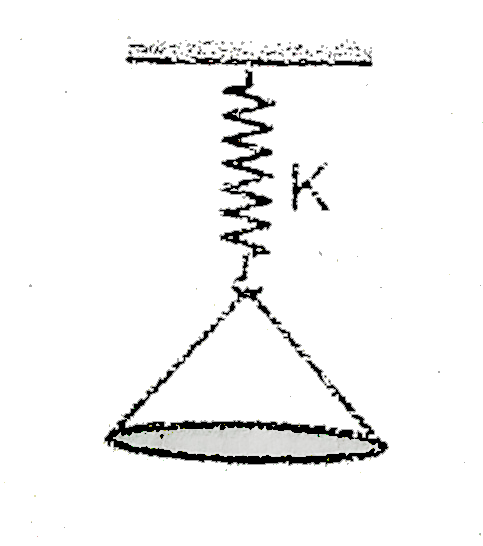
View SolutionA solid ball of mass m is made to fail from a height H on a pan suspended through a spring of spring constant K as shown in figure. If the does not rebound and the pan is massless, then amplitude of oscillation is
View SolutionA block of mass m is released from rest onto a spring. A having stiffness kA=mg/2h as shown in figure. If the block compresses spring B through a distance h, find the:
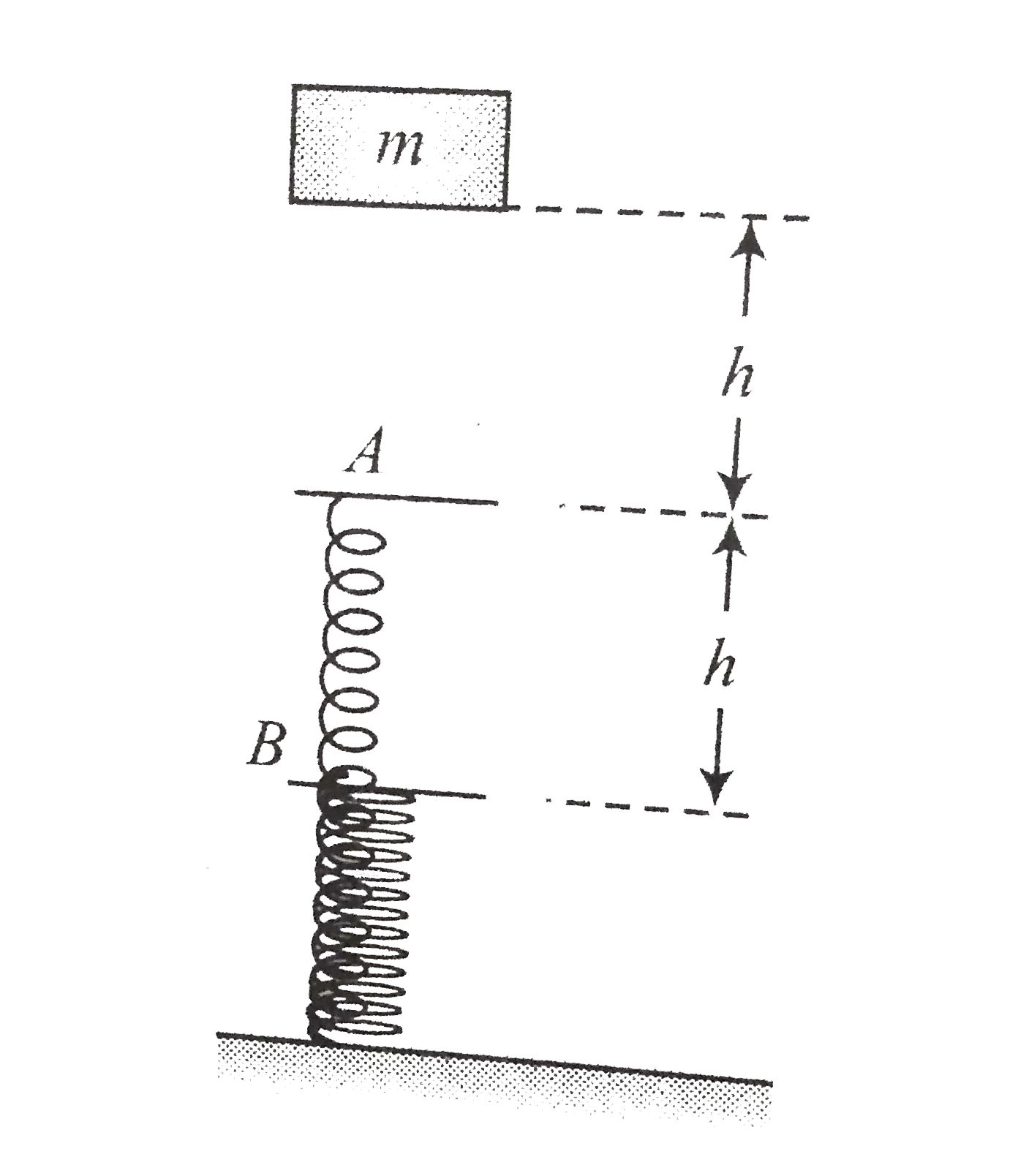
a. stiffness of the spring B
b. equilibrium position of the block
c. maximum velocity of the block
d. maximum acceleration of the block
View SolutionA block of mass m is released from rest onto a spring. A having stiffness kA=mg/2h as shown in figure. If the block compresses spring B through a distance h, find the:

a. stiffness of the spring B
b. equilibrium position of the block
c. maximum velocity of the block
d. maximum acceleration of the block
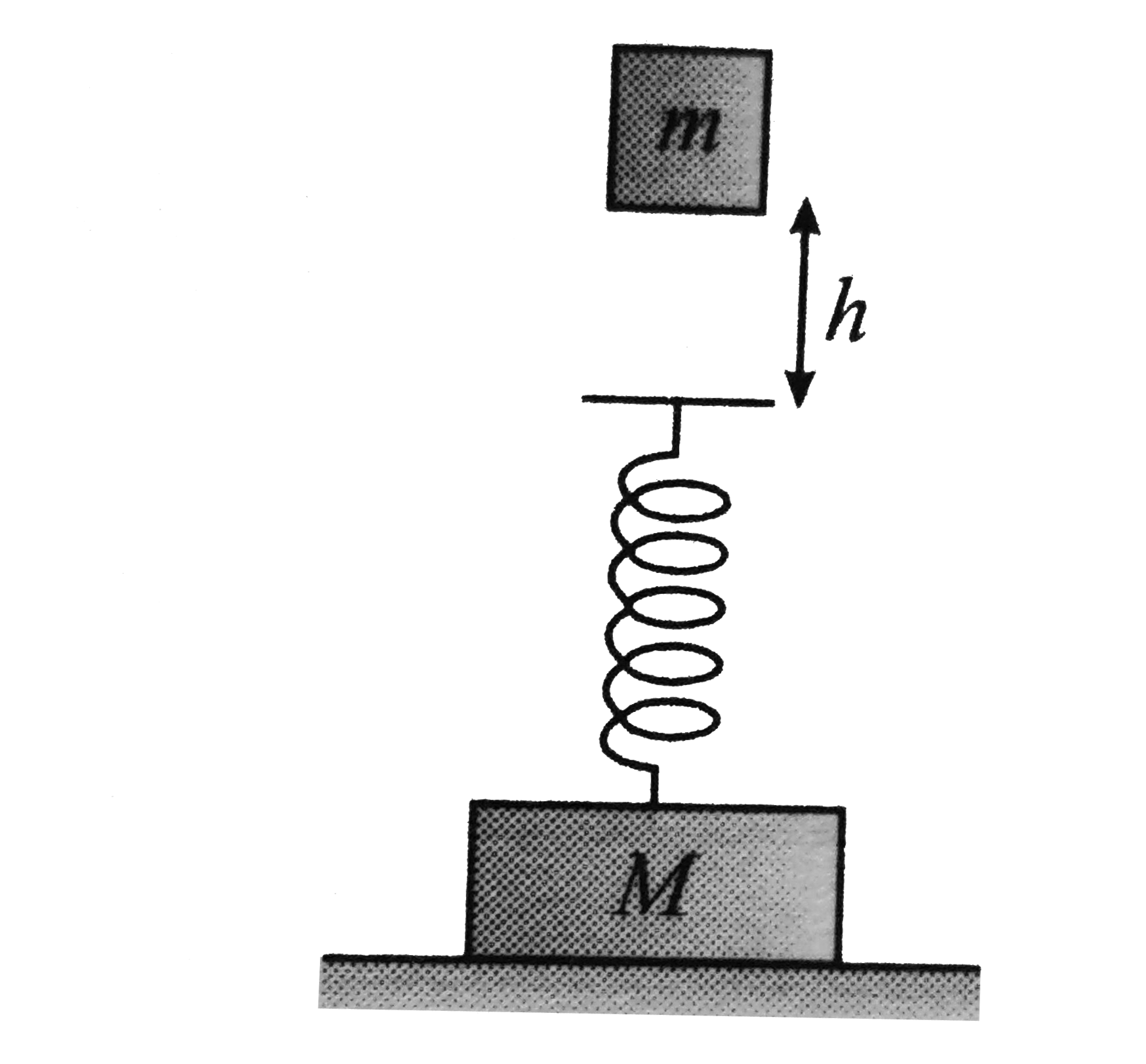
View SolutionA block of mass m is dropped onto a spring of constant k from a height h. The second end of the spring is attached to a second block of mass M as shown in figure. Find the minimum value of h so that the block M bounces off the ground. If the block of mass m sticks to the spring immediately after it comes into contact with it.
View SolutionA block of mass m is attached with a spring in vertical plane as shown in the figure. If initially spring is in its natural length and the block is released from rest, then maximum extension in the spring will be
View SolutionA ball of mass m is droppped from a height h on a platform fixed at the top of a vertical spring. The platform is depressed by a distance x. What is the spring constant K?
View SolutionIn an ideal pulley particle system, mass m2 is connected with a vertical spring of stiffness k. If mass m2 is released from rest, when the spring is underformed, find the maximum compression of the spring.
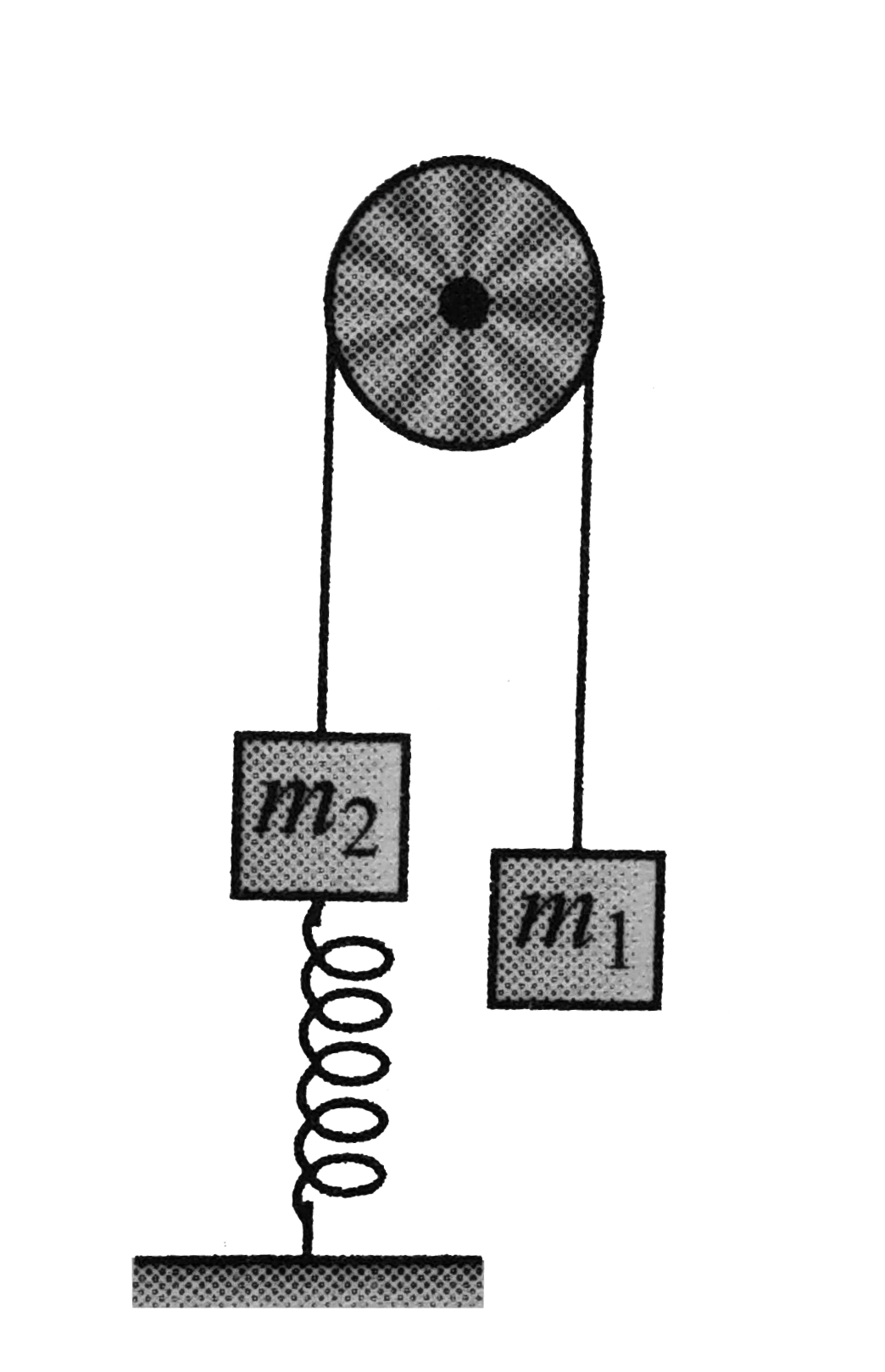
View Solution
