A ball is projected from a point in a horizontal plane so as to strike a vertical wall at right angle A.after rebounding from the wall it strikes the horizontal plane once and returns to the point of projection just before second collision at horizontal surface. Find co-efficient of restitution for the two collisions, assuming it to be same for both the collision.
To solve the problem of finding the coefficient of restitution for the two collisions of a ball projected towards a vertical wall and then rebounding, we can follow these steps:
1. Understanding the Problem:
- A ball is projected horizontally towards a vertical wall at point A.
- After hitting the wall, it rebounds and strikes the horizontal plane.
- The ball then returns to the original point of projection just before the second collision with the horizontal surface.
2. Defining Variables:
- Let the initial horizontal velocity of the ball be u.
- Let the angle of projection be θ.
- The coefficient of restitution (e) is the same for both collisions.
3. Finding the Time to Reach Point A:
- The time taken to reach the maximum height (point A) is given by:
t=usinθg
- This is the time taken to reach the height before hitting the wall.
4. Velocity at Point A:
- At point A, the horizontal component of the velocity is:
vx=ucosθ
5. Applying the Coefficient of Restitution:
- After rebounding from the wall, the velocity of separation (downward) can be expressed as:
v′=e⋅vx=e⋅(ucosθ)
6. Finding the Horizontal Distance (BN):
- The horizontal distance BN that the ball travels after rebounding before hitting the horizontal plane is given by:
BN=v′⋅t=e⋅(ucosθ)⋅(usinθg)
- This simplifies to:
BN=eu2sinθcosθg
7. Using the Range Formula:
- The horizontal range (A) for the initial projection is:
A=u2sin2θ2g
8. Setting Up the Equation:
- The distance OB can be expressed as:
OB=ON−BN=A−BN
- Substituting the values we have:
OB=u2sin2θ2g−eu2sinθcosθg
9. Equating the Ranges:
- The range for the rebound must equal the distance OB:
e2⋅u2sin2θg=u2(1−e)sin2θ2g
- Simplifying gives:
2e2=1−e
10. Forming the Quadratic Equation:
- Rearranging leads to:
2e2+e−1=0
11. Solving the Quadratic Equation:
- Using the quadratic formula:
e=−b±√b2−4ac2a=−1±√1+84=−1±34
- This gives:
e=12(since e cannot be negative)
Final Answer:
The coefficient of restitution e for the two collisions is 12.
- A ball is projected horizontally towards a vertical wall at point A.
- After hitting the wall, it rebounds and strikes the horizontal plane.
- The ball then returns to the original point of projection just before the second collision with the horizontal surface.
- Let the initial horizontal velocity of the ball be u.
- Let the angle of projection be θ.
- The coefficient of restitution (e) is the same for both collisions.
- The time taken to reach the maximum height (point A) is given by:
t=usinθg
- This is the time taken to reach the height before hitting the wall.
- At point A, the horizontal component of the velocity is:
vx=ucosθ
- After rebounding from the wall, the velocity of separation (downward) can be expressed as:
v′=e⋅vx=e⋅(ucosθ)
- The horizontal distance BN that the ball travels after rebounding before hitting the horizontal plane is given by:
BN=v′⋅t=e⋅(ucosθ)⋅(usinθg)
- This simplifies to:
BN=eu2sinθcosθg
- The horizontal range (A) for the initial projection is:
A=u2sin2θ2g
- The distance OB can be expressed as:
OB=ON−BN=A−BN
- Substituting the values we have:
OB=u2sin2θ2g−eu2sinθcosθg
- The range for the rebound must equal the distance OB:
e2⋅u2sin2θg=u2(1−e)sin2θ2g
- Simplifying gives:
2e2=1−e
- Rearranging leads to:
2e2+e−1=0
- Using the quadratic formula:
e=−b±√b2−4ac2a=−1±√1+84=−1±34
- This gives:
e=12(since e cannot be negative)
The coefficient of restitution e for the two collisions is 12.
Topper's Solved these Questions
Similar Questions
A ball is projected from a point in a horizontal plane with a velocity of 20 m/s at 30∘ with the horizontal. Coefficient of restitution is 0.8, then
View SolutionA ball is projected from ground with a speed 70m/s at an angle 45∘ with the vertical so that it strikes a vertical wall at horizontal distance 4903m from the point of projection. If the ball returns back to the poin tof projection without any collision with ground then find the coefficient of restitution between the ball and wall.
View SolutionA projectile is thrown from a point in a horizontal plane such that the horizontal and vertical velocities are 9.8ms−1 and 19.6ms−1. It will strike the plane after covering distance of
View SolutionA projectile is thrown from a point in a horizontal plane such that the horizontal and vertical velocities are 9.8ms−1 and 19.6ms−1. It will strike the plane after covering distance of
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at a distance a from the point of projection and returns to its original position. Find the coefficient of restitution between the ball and the wall.
View SolutionA ball falls from height h over an inclined plane of inclination 30°C as shown. After the collision, the ball moves horizontally, the coefficient of restitution is
_PHY_E05_227_Q001.png) View Solution
View SolutionA ball falls from height h over an inclined plane of inclination 30∘ as shown. After the collision, the ball moves horizontally, the coefficient of restitution is
_PHY_224_Q01.png) View Solution
View SolutionA ball of mass 1kg is dropped from a height of 3.2m on smooth inclined plane. The coefficient of restitution for the collision is e=1/2. The ball's velocity become horizontal after the collision.
 View Solution
View SolutionA ball moving with speed v collides with a horizontal smooth surface at an angle θ with normal to surface as shown in figure. If coefficient of restitution of collision is e, then find v'
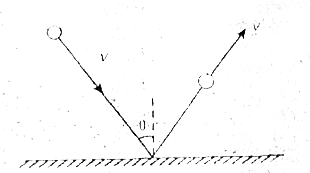 View Solution
View SolutionA small ball is projected from point P towards a vertical wall as shown in Fig. It hits the wall when its velocity is horizontal. Ball reaches point P after one bounce on the floor. The coefficient of restitution assuming it to be same for two collisions is n/2. All surfaces are smooth. Find the value of n.
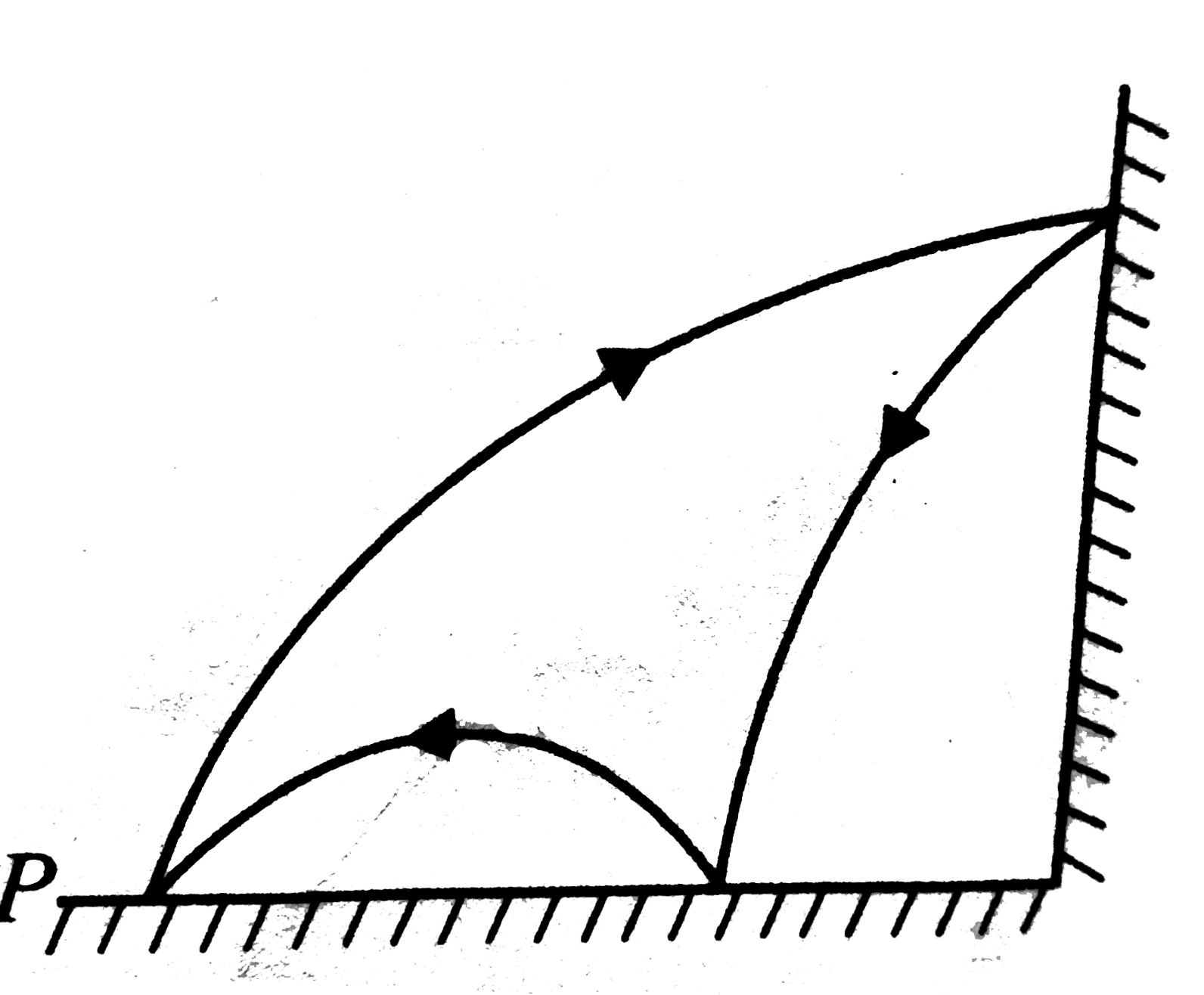 View Solution
View Solution

