A planet of mass M has uniform density in a spherical volume of radius R. Calculate the work done by the external agent to deassemble the planet in eight identical spherical part against gravitational pull amongst its constitute particle.
The correct Answer is:920Gm2R
To solve the problem of calculating the work done by an external agent to disassemble a planet of mass M and radius R into eight identical spherical parts against gravitational pull, we can follow these steps:
Step 1: Determine the mass of each spherical part
Since the planet is divided into 8 identical parts, the mass of each part m can be calculated as:
m=M8
Step 2: Determine the radius of each spherical part
The volume of the original planet is given by:
V=43πR3
The volume of each smaller sphere must also equal the total volume divided by 8:
Volume of each part=V8=18⋅43πR3=16πR3
Let r be the radius of each smaller sphere. Then:
43πr3=16πR3
Cancelling π and solving for r:
r3=R38⟹r=R2
Step 3: Calculate the gravitational potential energy of the original planet
The gravitational potential energy U of a solid sphere is given by:
U=−35GM2R
Step 4: Calculate the gravitational potential energy of the eight smaller spheres
The gravitational potential energy of the eight smaller spheres can be calculated as follows:
Uf=8(−35Gm2r)
Substituting m=M8 and r=R2:
Uf=8⎛⎜
⎜⎝−35G(M8)2R2⎞⎟
⎟⎠
Calculating this gives:
Uf=8⎛⎜⎝−35GM264R2⎞⎟⎠=8(−3GM2320R)=−24GM2320R=−3GM240R
Step 5: Calculate the work done by the external agent
The work done W by the external agent is the change in gravitational potential energy:
W=Uf−Ui
Substituting Ui and Uf:
W=(−3GM240R)−(−3GM25R)
Finding a common denominator (40R):
W=−3GM240R+24GM240R=21GM240R
Final Answer
Thus, the work done by the external agent to disassemble the planet into eight identical spherical parts is:
W=21GM240R
Since the planet is divided into 8 identical parts, the mass of each part m can be calculated as:
m=M8
The volume of the original planet is given by:
V=43πR3
The volume of each smaller sphere must also equal the total volume divided by 8:
Volume of each part=V8=18⋅43πR3=16πR3
Let r be the radius of each smaller sphere. Then:
43πr3=16πR3
Cancelling π and solving for r:
r3=R38⟹r=R2
The gravitational potential energy U of a solid sphere is given by:
U=−35GM2R
The gravitational potential energy of the eight smaller spheres can be calculated as follows:
Uf=8(−35Gm2r)
Substituting m=M8 and r=R2:
Uf=8⎛⎜
⎜⎝−35G(M8)2R2⎞⎟
⎟⎠
Calculating this gives:
Uf=8⎛⎜⎝−35GM264R2⎞⎟⎠=8(−3GM2320R)=−24GM2320R=−3GM240R
The work done W by the external agent is the change in gravitational potential energy:
W=Uf−Ui
Substituting Ui and Uf:
W=(−3GM240R)−(−3GM25R)
Finding a common denominator (40R):
W=−3GM240R+24GM240R=21GM240R
Thus, the work done by the external agent to disassemble the planet into eight identical spherical parts is:
W=21GM240R
Similar Questions
Mass M, of a planet earth is uniformly distributed over a spherical volume of radius R. Calculate the energy needed to deassemble the planet against the gravitational pull amongst its consituent particles. Given
mR=2.5×1031kgm and g=10ms−2.
View SolutionA sphere of radius R has a uniform volume density ρ. A spherical cavity of radius b, whose center lies at →a, is removed from the sphere.
i. Find the electric field at any point inside the spherical cavity.
ii. Find the electric field outside the cavity
(a) at points inside the large sphere but outside the cavity and
(b) at points outside the large sphere.

View SolutionAn interesting analogy based upon energy density. Find the work done by external agent to filll the mass m in a sherical volume of Radius R. (You may takethe example of earth)
View SolutionA particle of mass 'm' is raised from the surface of earth to a height h = 2R. Find work done by some external agent in the process. Here, R is the radius of earth and g the acceleration due to gravity on earth's surface.
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distanace R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA particle of mass m oscillates inside a smooth spherical shell of radius R and starts its motion from point B. At given instant the kinetic energy of the particle is K. Then the force applied by particle on the shell at this instant is :−
View Solution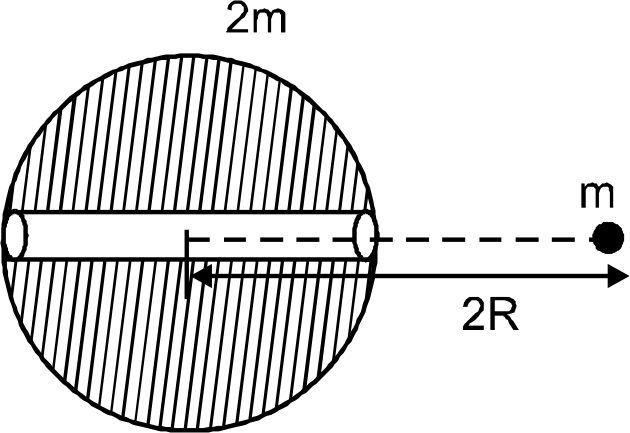
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View SolutionA thin spherical shell of mass M and radius R has a small hole. A particle of mass m released at its mouth. Then
View SolutionThe figure represents a solid uniform sphere of mass M and radius R. A spherical cavity of radius r is at a distance a from the centre of the sphere. The gravitational field inside the cavity is

View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m is
View Solution
