A block of mass 9 kg is lying on a rough horizontal surface. A particle of mass 1kg strikes the block with a speed of 50m/s at an angle of 37∘ with the vertical and sticks to it. The frictional coefficient between the ground and the block is μ=0.4. Find the velocity of the combined system just after the collision assuming limiting friction coefficient between the block and the ground after the collision ?
Less than √2m/s
Equal to √2m/s
Greater than √2m/s
None of the above
The correct Answer is:B
To solve the problem step by step, we will follow the principles of conservation of momentum and the effects of friction.
Step 1: Analyze the collision
The mass of the block (m1) is 9 kg, and the mass of the particle (m2) is 1 kg. The particle strikes the block with a speed of 50 m/s at an angle of 37 degrees with the vertical. We need to resolve the velocity of the particle into horizontal and vertical components.
Horizontal component (u_x):
ux=u⋅sin(θ)=50⋅sin(37∘)
Using sin(37∘)≈0.6:
ux=50⋅0.6=30m/s
Vertical component (u_y):
uy=u⋅cos(θ)=50⋅cos(37∘)
Using cos(37∘)≈0.8:
uy=50⋅0.8=40m/s
Step 2: Apply conservation of momentum in the horizontal direction
Before the collision, only the particle is moving. After the collision, both the block and the particle move together. Let V be the velocity of the combined system after the collision.
Using conservation of momentum in the horizontal direction:
m2⋅ux=(m1+m2)⋅V
Substituting the values:
1⋅30=(9+1)⋅V
30=10V
V=3m/s
Step 3: Calculate the frictional force
The frictional force can be calculated using the coefficient of friction (μ=0.4) and the normal force. The normal force (N) acting on the block after the collision is equal to the weight of the combined system:
N=(m1+m2)⋅g=(9+1)⋅10=100N
The maximum frictional force (F_f) is:
Ff=μ⋅N=0.4⋅100=40N
Step 4: Analyze the vertical momentum
Using conservation of momentum in the vertical direction:
m2⋅uy=(m1+m2)⋅Vy
Here, Vy is the vertical velocity of the combined system after the collision. Since the block does not move vertically, we have:
1⋅40=(9+1)⋅0
This shows that the vertical momentum is not transferred to the block, and thus the vertical velocity of the block remains 0.
Step 5: Check if the block moves
To check if the block will move, we need to compare the horizontal momentum with the frictional force. The horizontal momentum change is:
Δpx=m2⋅ux−Ff⋅Δt
Assuming a very small time interval (Δt), the frictional force will act against the motion. If Ff is greater than the momentum change, the block will not move.
Final Step: Conclusion
Since the horizontal velocity V=3m/s is less than the frictional force can overcome, the block will move with a velocity of:
V′=119m/s≈1.21m/s
Thus, the final velocity of the combined system just after the collision is approximately:
Velocity≈1.21m/s
The mass of the block (m1) is 9 kg, and the mass of the particle (m2) is 1 kg. The particle strikes the block with a speed of 50 m/s at an angle of 37 degrees with the vertical. We need to resolve the velocity of the particle into horizontal and vertical components.
ux=u⋅sin(θ)=50⋅sin(37∘)
Using sin(37∘)≈0.6:
ux=50⋅0.6=30m/s
uy=u⋅cos(θ)=50⋅cos(37∘)
Using cos(37∘)≈0.8:
uy=50⋅0.8=40m/s
Before the collision, only the particle is moving. After the collision, both the block and the particle move together. Let V be the velocity of the combined system after the collision.
m2⋅ux=(m1+m2)⋅V
Substituting the values:
1⋅30=(9+1)⋅V
30=10V
V=3m/s
The frictional force can be calculated using the coefficient of friction (μ=0.4) and the normal force. The normal force (N) acting on the block after the collision is equal to the weight of the combined system:
N=(m1+m2)⋅g=(9+1)⋅10=100N
The maximum frictional force (F_f) is:
Ff=μ⋅N=0.4⋅100=40N
Using conservation of momentum in the vertical direction:
m2⋅uy=(m1+m2)⋅Vy
Here, Vy is the vertical velocity of the combined system after the collision. Since the block does not move vertically, we have:
1⋅40=(9+1)⋅0
This shows that the vertical momentum is not transferred to the block, and thus the vertical velocity of the block remains 0.
To check if the block will move, we need to compare the horizontal momentum with the frictional force. The horizontal momentum change is:
Δpx=m2⋅ux−Ff⋅Δt
Assuming a very small time interval (Δt), the frictional force will act against the motion. If Ff is greater than the momentum change, the block will not move.
Since the horizontal velocity V=3m/s is less than the frictional force can overcome, the block will move with a velocity of:
V′=119m/s≈1.21m/s
Velocity≈1.21m/s
Similar Questions
A block of mass 4kg rests on a rough horizontal plane. The plane is gradually inclined until at an angle θ=60∘ , with the vertical, the mass just begins to side. What is the coefficient of static friction between the block and the plane?
View SolutionA block of mass 5kg is lying on a rough horizontal surface. The coefficient of static and kinetic friction are 0.3 and 0.1 and g=10ms−2 The frictional force on the block is
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A10.3NB23.8NC11.9ND6.3N
A 30kg block rests on a rough horizontal surface A force of 200N is applied on the block The block acquires a speed of 4m/s starting from rest in 2s What is the value of coefficient of friction ? .
View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block of mass m lying on a rough horizontal plance is acted upon by a horizontal force P and another force Q inclined at an angle θ to the vertical. The block will remain in equilibrium, if the coefficient of friction between it and the surface is
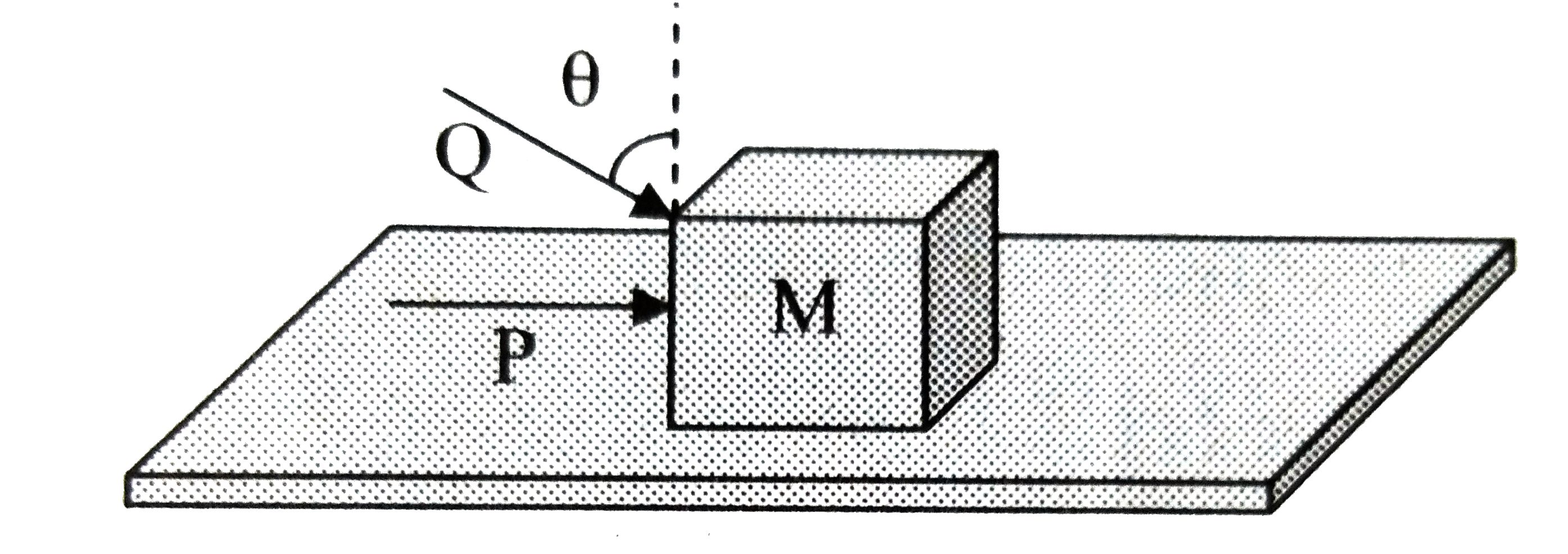 View Solution
View SolutionA block of mass'm' is resting on the floor of a lift. The coefficient of friction between the block and the floor is μ. When the lift is falling freely, the limiting frictional force between block and surface is
View SolutionA block of mass 50 kg can slide on a rough horizontal surface. The coefficient of friction between the block and the surface is 0.6. The least force of pull acting at an
angle of 30∘ to the upwawrd drawn ve3rtical which causes the block to just slide isView SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a forace of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View Solution
