A particle of mass m was transferred from the centre of the base of a uniform hemisphere of mass M and radius R into infinity.
What work was performed in the process by the gravitational force exerted on the particle by the hemisphere?
a) GMmR
b) −12GMmR
c) −32GMmR
d) −34GMmR
The correct Answer is:C
To find the work performed by the gravitational force exerted on a particle of mass m when it is transferred from the center of the base of a uniform hemisphere of mass M and radius R to infinity, we can follow these steps:
Step 1: Determine the Gravitational Potential at the Center of the Hemisphere
The gravitational potential V at a point due to a mass M is given by the formula:
V=−GMr
However, since we are dealing with a hemisphere, we need to consider the symmetry and the contribution of the mass distribution. For a uniform hemisphere, the potential at the center can be derived from the potential of the entire sphere and then halved.
The potential at the center of a full sphere of mass M and radius R is:
Vsphere=−3GM2R
Thus, for a hemisphere, the potential at the center is:
Vhemisphere=−3GM4R
Step 2: Calculate the Change in Potential Energy
The change in potential energy ΔU when moving the mass m from the center of the hemisphere to infinity (where the potential is zero) is given by:
ΔU=Ufinal−Uinitial
At infinity, the potential energy Ufinal=0. The initial potential energy Uinitial at the center of the hemisphere is:
Uinitial=m⋅Vhemisphere=m(−3GM4R)=−3mGM4R
Thus, the change in potential energy is:
ΔU=0−(−3mGM4R)=3mGM4R
Step 3: Calculate the Work Done by the Gravitational Force
The work done W by the gravitational force is equal to the negative of the change in potential energy:
W=−ΔU=−(3mGM4R)=−3mGM4R
Final Answer
The work performed by the gravitational force exerted on the particle by the hemisphere when it is moved to infinity is:
W=3mGM4R
---
V=−GMr
However, since we are dealing with a hemisphere, we need to consider the symmetry and the contribution of the mass distribution. For a uniform hemisphere, the potential at the center can be derived from the potential of the entire sphere and then halved.
Vsphere=−3GM2R
Thus, for a hemisphere, the potential at the center is:
Vhemisphere=−3GM4R
ΔU=Ufinal−Uinitial
At infinity, the potential energy Ufinal=0. The initial potential energy Uinitial at the center of the hemisphere is:
Uinitial=m⋅Vhemisphere=m(−3GM4R)=−3mGM4R
Thus, the change in potential energy is:
ΔU=0−(−3mGM4R)=3mGM4R
W=−ΔU=−(3mGM4R)=−3mGM4R
W=3mGM4R
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 3 (Miscellaneous Type Questions)
20 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 4 A (Conceptual Subjective Exercise)
14 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 1 (Check your Grasp)
28 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
Knowledge Check
A particle of mass m is placed at the centre of a unifrom spherical shell of mass 3 m and radius R The gravitational potential on the surface of the shell is .
AGMRB−GMRC−4GMRD−2GMRA uniform ring of mass m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at distance √3a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
AGMm8r2BGMm4r2C√3GMm8r2DGMm8r3√3
A particle of mass 1kg is kept on the surface of a uniform sphere of mass 20kg and radius 1.0m. Find the work to be done against the gravitational force between them to take the particle away from the sphere.
View SolutionA particle of mass 100 g is kept on the surface of a uniform sphere of mass 10 kg and radius 10 cm. Find the work to be done against the gravitational force between them to take the particle away from the sphere.
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionA uniform ring of mas m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at a distance √3 a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
View SolutionA uniform ring of mas m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The center of the ring is at a distance √3a from the center of the sphere. Find the gravitational force exerted by the sphere on the ring.
View SolutionCalculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
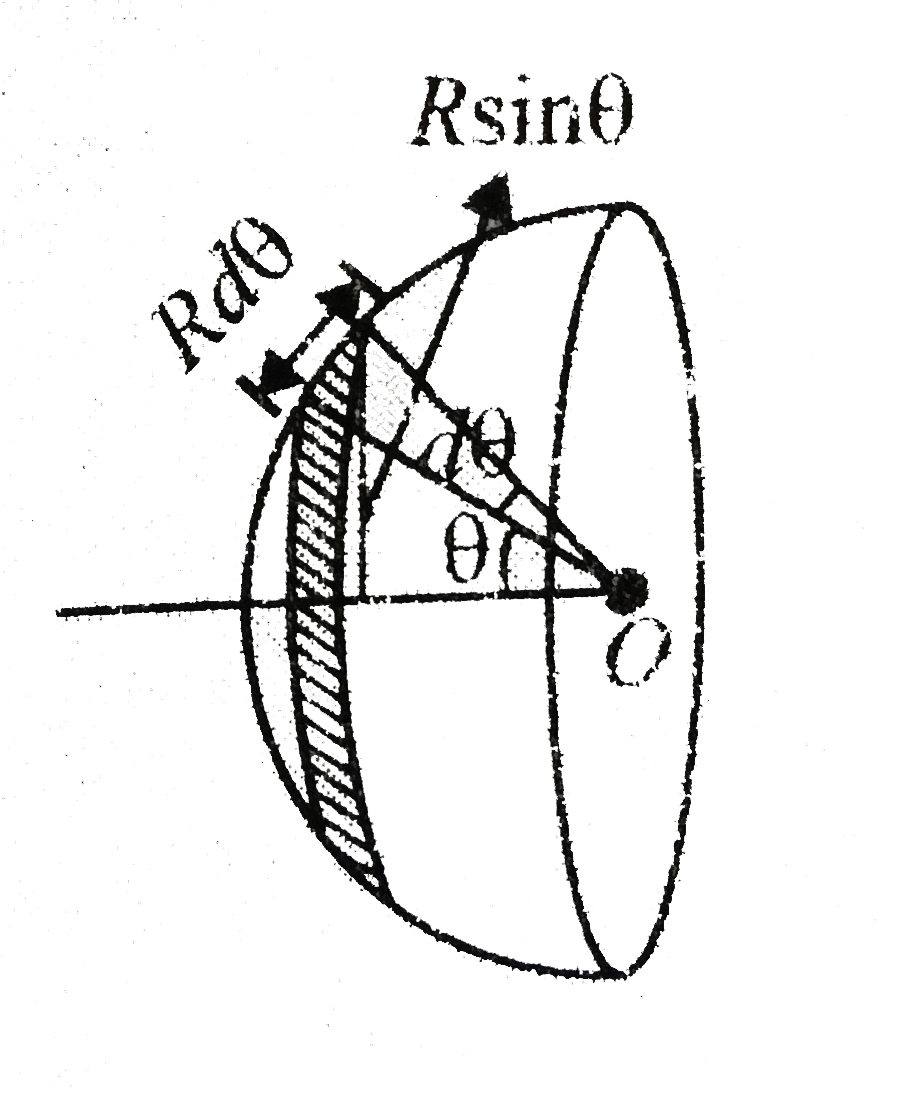
View Solution
