A steel wire of length 'l' has a magnetic moment 'M'. It is bent in 'L' shape having equal size of arm. The new magnetic moment is
The correct Answer is:M/√2
To find the new magnetic moment of a steel wire bent into an 'L' shape, we can follow these steps:
1. Understand the Initial Condition:
- The steel wire has a length l and a magnetic moment M. The magnetic moment is defined as the product of the pole strength and the length of the wire.
2. Bending the Wire:
- When the wire is bent into an 'L' shape, each arm of the 'L' will have equal lengths. Since the total length of the wire is l, each arm will have a length of l2.
3. Magnetic Moment of Each Arm:
- The magnetic moment for each arm can be calculated using the formula:
M′=m⋅l2
- Here, m is the pole strength. Since the total magnetic moment M is given by M=m⋅l, we can express the magnetic moment for each arm as:
M1=M2=M2
4. Direction of Magnetic Moments:
- Assume the first arm (vertical) has a magnetic moment M1 directed upwards, and the second arm (horizontal) has a magnetic moment M2 directed to the right.
5. Resultant Magnetic Moment:
- The resultant magnetic moment MR can be found using the Pythagorean theorem since the two arms are perpendicular to each other:
MR=√M21+M22
6. Substituting the Values:
- Substitute M1 and M2:
MR=√(M2)2+(M2)2
- This simplifies to:
MR=√2⋅(M2)2=√2⋅M2
7. Final Expression:
- Thus, the new magnetic moment MR can be expressed as:
MR=M√2
Conclusion:
The new magnetic moment of the steel wire bent into an 'L' shape is:
New Magnetic Moment=M√2
- The steel wire has a length l and a magnetic moment M. The magnetic moment is defined as the product of the pole strength and the length of the wire.
- When the wire is bent into an 'L' shape, each arm of the 'L' will have equal lengths. Since the total length of the wire is l, each arm will have a length of l2.
- The magnetic moment for each arm can be calculated using the formula:
M′=m⋅l2
- Here, m is the pole strength. Since the total magnetic moment M is given by M=m⋅l, we can express the magnetic moment for each arm as:
M1=M2=M2
- Assume the first arm (vertical) has a magnetic moment M1 directed upwards, and the second arm (horizontal) has a magnetic moment M2 directed to the right.
- The resultant magnetic moment MR can be found using the Pythagorean theorem since the two arms are perpendicular to each other:
MR=√M21+M22
- Substitute M1 and M2:
MR=√(M2)2+(M2)2
- This simplifies to:
MR=√2⋅(M2)2=√2⋅M2
- Thus, the new magnetic moment MR can be expressed as:
MR=M√2
The new magnetic moment of the steel wire bent into an 'L' shape is:
New Magnetic Moment=M√2
Similar Questions
A steel wire of length l has a magnetic moment M. It is bent into a semicircular arc. What is the new magnetic moment?
View SolutionA steel wire of length l has a magnetic moment M. It is bent into a semicircular arc. What is the new magnetic moment?
View SolutionA bar magnet of magnetic moment M is bent in the form of quadrant of circular arc . The new magnetic moment is
View SolutionA bar magnet of lenth l and magnetic dipole moment 'M' is bent in the form of an arc as shown in figure. The new magnetic dipole moment will be
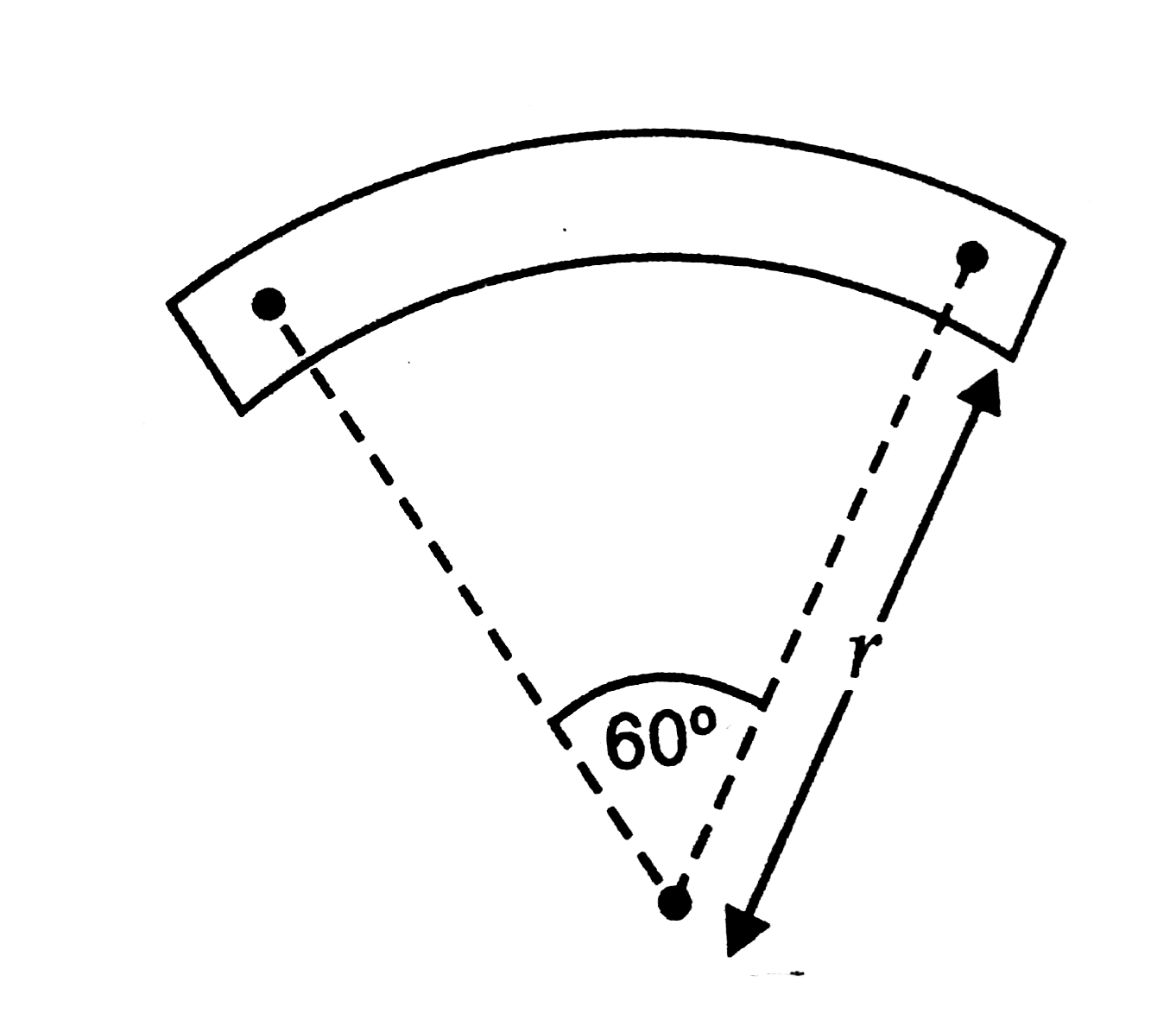
View SolutionA magnetic wire of dipole moment 4πAm2 is bent in the form of semicircle. The new magnetic moment is
View SolutionA long thin magnet of moment M is bent into a semi circle. The decrease in the magnetic moment is
View SolutionThe magnetic moment has dimensions of
View SolutionThe pair having similar magnetic moment is
View SolutionWhich of the following have same magnetic moment
View SolutionAn iron rod of length L and magnetic moment M is bent in the form of a semicircle. Now its magnetic moment will be
View Solution
