Derive an expression for magnetic field at a point on axial line of the bar magnet.
Magnetic field intensity : It is defined as the force experienced by a unit positive charge moving with unit velocity in a direction perpendicular to the magnetic field. Magnetic field intensity at a point on the axial line Consider a bar magnet of length 2l.
Let P be the point on the axial line at a distance r from the centre of the magnet. Let m be the pole strength of each pole. Magnetic field at point P due to north pole,
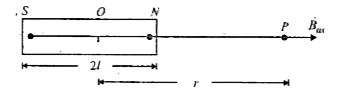
BN=μ04π⋅m(r−l)2 .....(i)
Magnetic field at point P due to south pole,
BS=μ04π⋅m(r+l)2 ....(ii)
∴ Net magnetic field intensity at point P due to bar magnet,
Bax=μ04π⋅m(r−l)2−μ04π⋅m(r+l)2=μ04πm[1(r−l)2−1(r+l)2]
=μ04π[(r+l)2−(r−l)2(r2−l2)2]=μ04πm[2r×2l(r2−l2)2]
Bax=μ04π⋅2Mr(r2−l2)2[∴m×2l=M]
If the magnet have small length, then l2<<r2
∴ Bax=μ04π2Mr3
Similar Questions
Using Biot-Savart’s law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
View SolutionUsing Biot-Savart’s law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
View Solution
Knowledge Check
Assertion: Magnetic field strength at a point on axial line of a bar magnet is along South to North pole of magnet.
Reason: The magnetic field strength can never be along North to South pole of magnet.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true and reason is not the correct explanation of assertion.CIf assertion is ture but reason is false.DIf both assertion and reason are false.Statement-1: Magnetic field strength at a point on axial line of a bar magnet is along South to North pole of magnet.
Statement-2: The magnetic field strength can never be along North to South pole of magnet.AStatement-1 is true, Statement-2 is true, Statement-2 is a correct explaination of Statement-1.BStatement-1 is true, Statement-2 is true, Statement-2 is not a correct explanation of Statement-1.CStatement-1 is true, Statement-2 is false.DStatement-1 is false, Statement-2 is true.
Using Biot - Savart's law, derive an expression for magnetic field at any point on axial line of a current carrying circular loop. Hence, find magnitude of magnetic field intensity at the centre of circular coil.
View SolutionWrite an expression for magnetic field intensity at any point an axial line of a bar magnet.
View SolutionDefine axial line of a bar magnet.
View SolutionDefine axial line of a bar magnet.
View SolutionDerive an expression for the magnetic field due to a solenoid of length 2l, radius a, having n number of turns per unit length and carrying a steady current I, at a point on the axial line, distant r from the centre of the solenoid. How does this expression compare with the axial magnetic field due to a bar magnet of magnetic moment M?
View SolutionOrientation of two identical small bar magnet of magnetic moment 'M' is as shown in figure. The magnitude of magnetic field at the point which lies on equatorial line of one magnet and axial line of another magnet is (r >> size of magnet)
View Solution
