The tangents drawn at point A and B of a circle with centre O, meet at P. If ∠AOB=120∘ and AP = 6 cm, then what is the area of triangle APB (in cm2) ?
6√3
8√3
9
9√3
The correct Answer is:D
To find the area of triangle APB given the conditions in the problem, we can follow these steps:
Step 1: Understand the Geometry
We have a circle with center O, and tangents AP and BP meeting at point P. The angle AOB is given as 120 degrees. Since AP and BP are tangents to the circle, we know that OA and OB are radii of the circle.
Step 2: Identify Angles
Since OA and OB are radii, the angles OAP and OBP are both right angles (90 degrees). Therefore, we can find angle APB using the fact that the angles in triangle AOB sum up to 180 degrees:
∠APB=180∘−∠AOB−∠OAP−∠OBP=180∘−120∘−90∘−90∘=60∘
Step 3: Use Properties of Isosceles Triangle
Since AP = PB (both are tangents from the same external point P), triangle APB is isosceles with AP = PB = 6 cm. We already found that angle APB = 60 degrees.
Step 4: Calculate Area of Triangle APB
The area A of triangle APB can be calculated using the formula for the area of a triangle:
A=12×base×height
In triangle APB, we can use the formula for the area of an isosceles triangle:
A=12×AP×PB×sin(∠APB)
Substituting the known values:
A=12×6×6×sin(60∘)
Since sin(60∘)=√32:
A=12×6×6×√32=36√34=9√3 cm2
Final Answer
The area of triangle APB is 9√3 cm2.
---
We have a circle with center O, and tangents AP and BP meeting at point P. The angle AOB is given as 120 degrees. Since AP and BP are tangents to the circle, we know that OA and OB are radii of the circle.
Since OA and OB are radii, the angles OAP and OBP are both right angles (90 degrees). Therefore, we can find angle APB using the fact that the angles in triangle AOB sum up to 180 degrees:
∠APB=180∘−∠AOB−∠OAP−∠OBP=180∘−120∘−90∘−90∘=60∘
Since AP = PB (both are tangents from the same external point P), triangle APB is isosceles with AP = PB = 6 cm. We already found that angle APB = 60 degrees.
The area A of triangle APB can be calculated using the formula for the area of a triangle:
A=12×base×height
In triangle APB, we can use the formula for the area of an isosceles triangle:
A=12×AP×PB×sin(∠APB)
Substituting the known values:
A=12×6×6×sin(60∘)
Since sin(60∘)=√32:
A=12×6×6×√32=36√34=9√3 cm2
The area of triangle APB is 9√3 cm2.
Topper's Solved these Questions
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - III
21 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - IV
169 Videos
- MENSURATIONBOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Test Yourself
28 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
- MISCELLANEOUSBOOK - KIRAN PUBLICATIONCHAPTER - MISCELLANEOUSEXERCISE - TYPE-VI
15 Videos
Similar Questions
Knowledge Check
The tangents drawn at points A and B of a circle with centre O, meet at P. If ∠AOB=120∘ and AP=6cm then what is the area of quad APB (in cm2) ?
A6√3B8√3C9D12√3Two tangents drawn at the points A and B of a circle centred at O meet at P. If ∠AOB=120∘ then ∠APB:∠APO is
A2:5B3:2C4:1D2:1The tangents drawn at the point P and Q of a circle centered at O meet at A. If ∠POQ=120∘, then what is the ratio of ∠PAQ:∠PAO?
A2:3B4:1C2:1D5:2
Two tangents drawn at the points A and B of a circle centred at O meet at P. If ∠AOB=120∘ then ∠APB:∠APO is
View SolutionThe tangents at two points A and B on the circle with centre O intersect at P. If in quadrilateral PAOB, ∠AOB:∠APB=5:1, then measure of ∠APB is :
View SolutionThe tangents at two points A and B on the circle with centre O Intersects at P, If in quadrilateral AOBP,∠AOB:∠APB=5:1, then measure of ∠APBis:
View SolutionIn figure , APB is a tangent to a circle with centre O at point, If ∠QPB=50∘ , then the measure of ∠POQ is
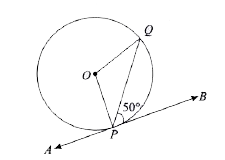
View SolutionTwo tangents PA and PB are drawn to a circle of centre O in such a way that ∠AOB=120∘. Calculate the value of ∠APB.
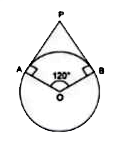
View Solution
