The median BD of the triangle ABC meets AC at D. If BD=12AC , then which one of the following is correct ?
∠ACB=1 right angle
∠BAC=1 right angle
∠ABC=1 right angle
None of the above
The correct Answer is:C
Step by step video, text & image solution for The median BD of the triangle ABC meets AC at D. If BD = 1/2AC , then which one of the following is correct ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
- BOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B8videos
CENTRE OF TRIANGLE
- BOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B8videos
CENTRE OF TRIANGLE
- BOOK - LUCENT PUBLICATIONCHAPTER - ALGEBRAIC IDENTITIES EXERCISE - Exercise - 1B28videos
ALGEBRAIC IDENTITIES
- BOOK - LUCENT PUBLICATIONCHAPTER - CIRCLE AND ITS TANGENT LINESEXERCISE - EXERCISE 8B27videos
CIRCLE AND ITS TANGENT LINES
Similar Questions
Knowledge Check
- Question 1 - Select One
The median AD of the triangle ABC is bisected at E and BE meets AC at F. Find AF:FC.
A3/4B1/3C1/2D1/4 - Question 2 - Select One
ABCD is a quadrilateral. AM and CN are perpendiculars to BD, AM= CN and diagonals AC and BD intersect at O, then which one of the following is correct?
AAO=OCBBO=ODCAO=BODCO=DO - Question 3 - Select One
ABC is a triangle right- angled at B and D is a point on BC produced (BD>BC), such that BD=2DC. Which one of the following is correct?
AAC2=AD2−3CD2BAC2=AD2−2CD2CAC2=AD2−4CD2DAC2=AD2−5CD2
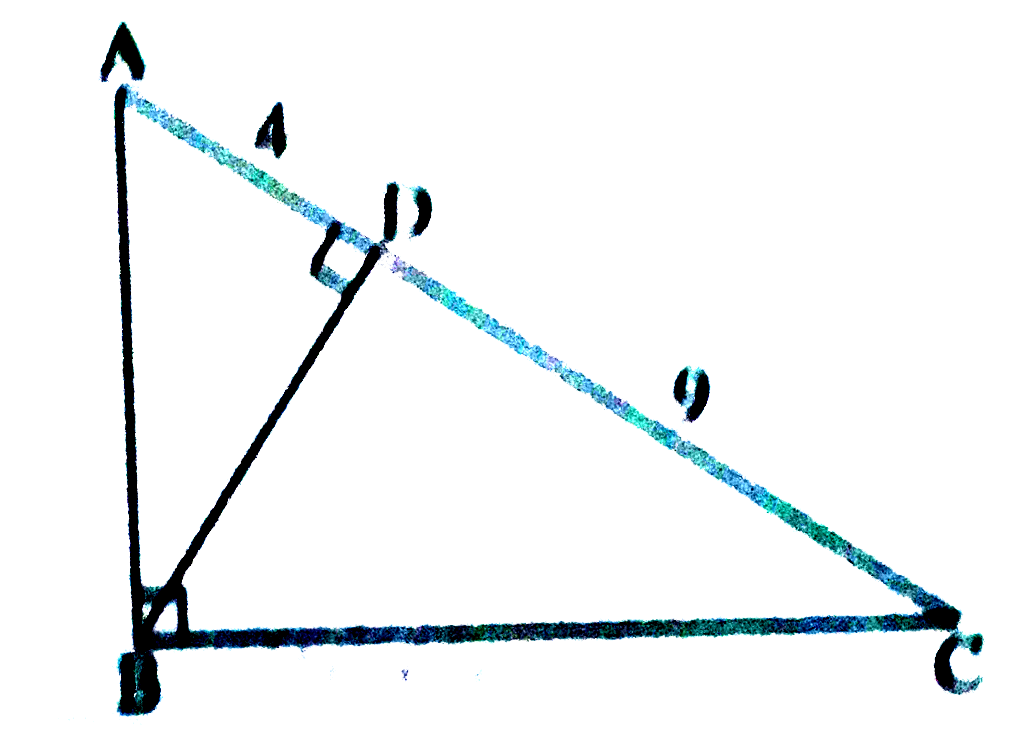
In right -angled △ABC,BD⊥AC.
If AD =4 DC =9 then find BD.View SolutionABCD is a quadrilateral whose diagonals are AC and BD. Which one of the following is correct?
View SolutionABCD is a quadrilateral whose diagonals are AC and BD. Which one of the following is correct?
View SolutionIn the right angle triangle ∠C=90∘. AE and BD are two medians of a triangle ABC meeting at F. The ratio of the area of △ABF and the quadrilateral FDCE is :
View SolutionIn the triangle ABC, the internal bisector of angle A meets the side BC at D. If BD=7.5 cm and BC=9 cm. Then calculate the value of AB:AC.
View Solution

