Deduce an expression for electrostatic potential due to a point charge.
Electric Potential due to a Point Charge
Let P be the point at a distance r from the origin O at which the electric potential due to a charge +q is required as shown in the figure given below:
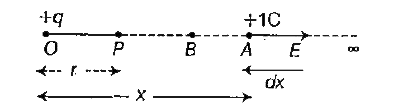
The electric potential" at a point P is the amount of work done in carrying a unit positive charge from infinity (∞) to point P.
As work done is independent of the path, we choose a convenient path along the radial direction from infinity to the point P without an acceleration.
Let A be an intermediate point on this path, where OA = x.
The electrostatic force on unit positive charge A is.
F=14πε0.qx2, along OA
Small work done in moving the charge through a distance ox from point A to B is:
dW=F.dx=Fdxcos180∘=−Fdx(cos180∘=−1)
dW=−Fdx
Total work done in moving a unit positive charge from ∞ to the point P is:
W=∫r∞−Fdx=∫t∞−14πε0qx2dx
=q4πε0[1r−1∞](∫x−2dx=−12)
W=q4πε0r
from the definition of an electric potential, this work done is equal to the potential at point P.
V=q4πε0r
Similar Questions
Obtain an expression for electric potential .V due to a point charge .Q at a distance r.
View SolutionDeduce an expression for the electric potential due to an electric dipole at any point on its axis. Mention one contrasting feature of electric potential at a point due to dipole as compared to that due to a single charge.
View SolutionDeduce an expression for the electric potential due to an electric dipole at any point on its axis. Mention one contrasting feature of electric potential of a dipole at a point as compared to that due to a single charge.
View SolutionDefine electrostatic potential energy. Obtain an expression for electrostatic potential at a point on the equatorial line of an electric dipole.
View SolutionFind an expression for the potential at a point due to a point charge Q.
View SolutionTwo point charges q1, and q2 are kept at a distance of r12 in air. Deduce the expression for the electrostatic potential energy of this system.
View Solution(a) Obtain the expression for the potential due to a point charge.
(b) Use the above expression to show that the potential, due to an electric dipole (length 2a), varies as the inverse square' of the distance r of the 'field point' from the centre of the dipole for r>a.
View Solution(a) Obtain the expression for the potential due to a point charge.
(b) Use the above expression to show that the potential, due to an electric dipole (length 2a), varies as the inverse square' of the distance r of the 'field point' from the centre of the dipole for r>a.
View SolutionWhat do you understand by electrostatic potential energy ? Find an expression for electrostatic potential energy of a system of two point charges.
View SolutionDeduce an expression for the electrostatic energy stored in a capacitance 'C' and having charge Q
How will the energy stored
View Solution
