Apply Ampere's circuital law, find the magnetic flux density at the centre of a long solenoid carrying current.
Let us consider a solenoid having current I, number of turns per unit length n. To calculate the magnetic field B, we consider a loop PQRS having length L Hence, total current through the loop is (nL)I.
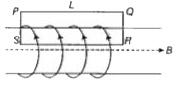
According to Ampere.s circuital law,
∫PQRSB⋅dl=μ0(NLl)
∮B⋅dl=∮QPB⋅dl+∫RQB⋅dl+∫SRB⋅dl+∫PSB⋅dl
Now ∫RQB⋅dl=∫PS⋅dl=0
(Because along QR and PS the field B is at right angles to d l, so that
B⋅dl=B⋅dlcos90∘=0)
∮B⋅dl=∫QP0dl+∫RQBdlcos90∘+∫SRBdlcos0∘+∫PSBdlcos90∘
=0+0+B∫SRdl+0=BL
Hence, from Ampere.s law,
∮B⋅dl=μ0× current enclosed by PQRSP
The current in each turns is I, so that current enclosed by the loop is nLl.
∴Inet=nLI
∮B⋅dl=μ0×nLI
∴BL=μ0nLI⇒B=μ0nI
Similar Questions
Can you use Ampere's law of find the magnetic field at the centre of a ring of radius R and carrying current l?
View SolutionCan you use Ampere's law of find the magnetic field at the centre of a ring of radius R and carrying current l?
View SolutionApply Ampere's circuital law to determine the magnetic induction inside a thick wire of radius R and carrying I amperes of current.
View Solution(i) State Biot-Savart.s law.
(ii) Find magnetic flux density at a point on the axis of a long solenoid having 5000 turns m when it carrying a current of 2 A.
View Solution(a)Using Ampere's circuital law, obtain the expression for the magnetic field due to a long solenoid at a point inside the solenoid on its axis.
(b )How is the magnetic field inside a given solenoid made strong?
View Solution(a) Using Ampere's circuital law, obtain the expression for the magnetic field due to a long solenoid at a point inside the solenoid on its axis.
(b) In what respect is a toroid different from a solenoid ? Draw and copare the patern of the magnetic field lines in the two cases.
(c) How is the magnetic field inside a given solenoid made strong?
View SolutionUsing Ampere.s circuital law, obtain an expression for the magnetic flux density .B. at a point .X. at a perpendicular distance .r. from a long current carrying conductor. (Statement of the law is not required).
View SolutionUsing Ampere's circuital law, obtain an expression for the magnetic field along the axis of a current carrying solenoid of length l and having N number of turns.
View SolutionUsing Ampere's circuital law, obtain an expression for the magnetic field along the aixs of a current carrying solenoid of length l and having N number of turns.
View Solution(a)Using Ampere's circuital law,obtain the expression for the magnetic field due to a long solenoid at a point inside the solenoid on its axis.
(b)In what respect is a toroid different from a solenoid?Draw and compare the pattern of the magnetic field lines in the two cases.
(c)How is the magnetic field inside a given solenoid made strong?
View Solution
