Question

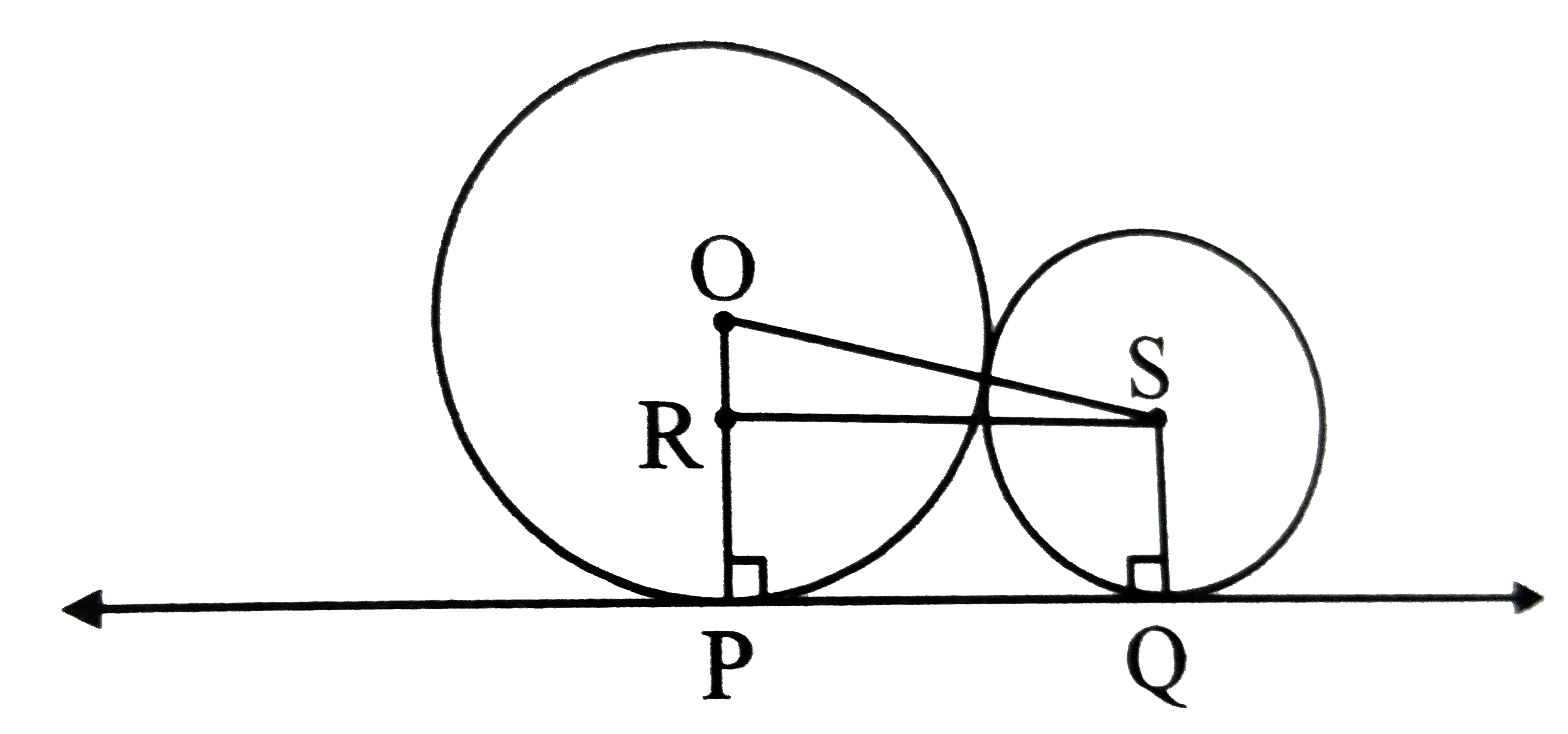
Radii of two circles are respectively 25 cm and 9 cm and their centres are 34 cm apart common tangent to the two.

Answer

30 cm

Explanation

To find the length of the common tangent to two circles with given radii and distance between their centers, we can follow these steps:

1. Identify the Given Values:
- Radius of the first circle (R) = 25 cm
- Radius of the second circle (r) = 9 cm
- Distance between the centers of the circles (d) = 34 cm

2. Use the Formula for the Length of the Direct Common Tangent:
The formula for the length of the direct common tangent (PQ) between two circles is given by:
\( PQ = \sqrt{d^2 - (R - r)^2} \)

3. Substitute the Values into the Formula:
- First, calculate \(R - r\):
\( R - r = 25 - 9 = 16 \text{ cm} \)
- Now substitute the values into the formula:
\( PQ = \sqrt{34^2 - (16)^2} \)

4. Calculate \(34^2\) and \(16^2\):
- \(34^2 = 1156\)
- \(16^2 = 256\)

5. Subtract \(16^2\) from \(34^2\):
\( 1156 - 256 = 900 \)

6. Take the Square Root:
\( PQ = \sqrt{900} = 30 \text{ cm} \)

Final Answer:
The length of the common tangent (PQ) is 30 cm.

---