ABCD is a quadrilateral such that BC=BA and CD>AD. Which one of the following is correct?
∠BAD=∠BCD
∠BAD<∠BCD
∠BAD>∠BCD
2∠BAD=∠BCD
To solve the problem step by step, we will analyze the given conditions and derive the relationship between the angles in the quadrilateral ABCD.
1. Understanding the Quadrilateral:
We have a quadrilateral ABCD where it is given that BC=BA and CD>AD. This means that triangle ABC is isosceles with AB=BC.
Hint: Visualize the quadrilateral and label the sides according to the given information.
2. Identifying Angles:
Since AB=BC, we can denote the angles at points A and C as follows:
- Let ∠BAD=α
- Let ∠BCD=β
In triangle ABC, since AB=BC, we have:
∠ABC=∠ACB=α
Hint: Remember that in an isosceles triangle, the angles opposite the equal sides are also equal.
3. Using the Condition CD>AD:
The condition CD>AD implies that the angle opposite to side CD (which is ∠CAD) is greater than the angle opposite to side AD (which is ∠DCA). Therefore, we can denote:
- Let ∠CAD=θ
- Let ∠DCA=ϕ
Since CD>AD, we have:
θ>ϕ
Hint: Relate the lengths of the sides to the angles opposite them.
4. Relating Angles:
From our triangle ACD, we can express the angles:
∠ACD=β(since it is the same angle as in triangle ABC)
Therefore, we can write:
θ+β+ϕ=180∘
Hint: Use the triangle angle sum property to relate the angles.
5. Combining the Relationships:
Since θ>ϕ, we can infer that:
∠BAD=α>β
This means that:
∠BAD>∠BCD
Hint: Use the inequalities derived from the side lengths to compare the angles.
6. Conclusion:
From our analysis, we conclude that:
∠BAD>∠BCD
Thus, the correct option is that ∠BAD is greater than ∠BCD.
Final Answer:
The correct statement is: Angle BAD is greater than angle BCD.
We have a quadrilateral ABCD where it is given that BC=BA and CD>AD. This means that triangle ABC is isosceles with AB=BC.
Since AB=BC, we can denote the angles at points A and C as follows:
- Let ∠BAD=α
- Let ∠BCD=β
∠ABC=∠ACB=α
The condition CD>AD implies that the angle opposite to side CD (which is ∠CAD) is greater than the angle opposite to side AD (which is ∠DCA). Therefore, we can denote:
- Let ∠CAD=θ
- Let ∠DCA=ϕ
θ>ϕ
From our triangle ACD, we can express the angles:
∠ACD=β(since it is the same angle as in triangle ABC)
Therefore, we can write:
θ+β+ϕ=180∘
Since θ>ϕ, we can infer that:
∠BAD=α>β
This means that:
∠BAD>∠BCD
From our analysis, we conclude that:
∠BAD>∠BCD
The correct statement is: Angle BAD is greater than angle BCD.
Similar Questions
Knowledge Check
ABCD is a quadrilateral whose diagonals are AC and BD. Which one of the following is correct?
A−−→BA+−−→CD=−−→AC+−−→DBB−−→BA+−−→CD=−−→BD+−−→CAC−−→BA+−−→CD=−−→AC+−−→BDD−−→BA+−−→CD=−−→BC+−−→ADABCD is a quadrilateral whose diagonals are AC and BD. Which one of the following is correct?
AA. −−→BA+−−→CD=−−→AC+−−→DBBB. −−→BA+−−→CD=−−→BD+−−→CACC. −−→BA+−−→CD=−−→AC+−−→BDDD. −−→BA+−−→CD=−−→BC+−−→ADSides BA and DC of quadrilateral ABCD are extended as per diagram then which one of the follwing is true?
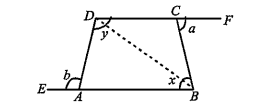 Ax+y=a+bBx+y=a-bCx-y=(a+b)/2D(x+y)/2=a-b
Ax+y=a+bBx+y=a-bCx-y=(a+b)/2D(x+y)/2=a-b
ABCD is a quadrilateral. Is AB+BC+CD+DA>AC+BD?
View SolutionABCD is a quadrilateral in which AB=CD and AD=BC. show that it is a parallelogram.
View SolutionABCD is a quadrilateral in which AB=BC and AD =CD ,Show that BD bisects boht the angle ABC and ADC
View SolutionIn a quadrilateral ABCD, AC bisects ∠C and BC = CD, then which of the following statement is false?
View SolutionLet ABCD be a cyclic quadrilateral such that AB=2,BC=3,∠B=120∘ and area of quadrilateral =4√3. Which of the following is/are correct ?
View Solution
