माना आगंड समतल में किसी बिंदु P(z) का बिंदुपथ arg(z−5iz+5i)=π4है। यदि O मूलबिंदु है, तब अधिकतम (OP) + न्यूनतम (OP)2 का मान है
5√2
5+5√2
5+5√2
10−5√2
The correct Answer is:B
Topper's Solved these Questions
Similar Questions
P1:x+y+2z=3 और
P2:x−2y+z=4 दो समतल है। माना
A(2,4,5) और B(4,3,8) त्रिविम में दो बिंदु है। P1 और P2 की प्रतिच्छेद रेखा से होकर गुजरने वालो समतल P3 का समीकरण, इस प्रकार है कि इस पर रेखाखंड AB के प्रक्षेप की लंबाई न्यूनतम है,हैं04:50View Solutionसमीकरण √x+14−8√x−2+√x+23−10√x−2=3के वास्तविक हलों की संख्या हैं
View SolutionThe locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
Then the length of the arc described by the locus of P(z) is05:04View SolutionThe locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
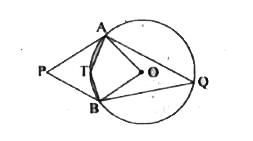
Area of the region bounded by the locus of a complex number Z satisfying arg(z+5iz−5i)=±π401:02View Solutionआकृति में O वृत्त का केंद्र है। यदि ∠APB=70∘ तो ∠AQB का मान है -
 01:58View Solution
01:58View Solutionयदि z = x + iy हो, तो |z| का मान …………. है ।
08:25View Solutionयदि z = 2 - 3i तब z.¯z का मान …………. है ।
03:32View SolutionLet the locus of any point P(z) in the argand plane is arg(z−5iz+5i)=π4. If O is the origin, then the value of max.(OP)+min.(OP)2 is
07:04View Solutionसुसंगत क्षेत्र में बिंदु जो उदेश्य फलन का इष्टतम मान (अधिकतम या न्यूनतम ) एक देता है, वह कहलाता है -
03:52View Solutionव्यवरोधों x+3y≥3,x+y≥2,x≥,y≥0 के अंतर्गत Z=3x+8y का न्यूनतम मान है।
04:48View Solutionरेखा x−42=y−52=z−31 तथा समतल x+y+z=2 का प्रतिच्छेदन बिंदु निम्न में से किस रेखा पर स्थित है ?
02:23View Solutionयदि z−αz+α(α∈R) एक शुद्ध रूप से काल्पनिक संख्या है तथा |z|=2 है तो α का एक मान है
05:17View Solutionकिसी सम्मिश्र संख्या z के लिए |z|+|z-1| का न्यूनतम मान है
02:57View Solutionबिन्दु पथ (लोकस) जिसका समीकरण arg(z+iz−i)=π4 है, का परिमाप (पेरामीटर) है
01:42View SolutionThe locus of z such that arg[(1−2i)z−2+5i]=π4 is a
01:09View SolutionThe locus of any point P(z) on argand plane is arg(z−5iz+5i)=π4.
Then the length of the arc described by the locus of P(z) is02:50View Solution

