A current of 1.5 A is flowing through a triangle of side 9 cm each .The magnetic field at the centroid of the triangle is : (Assume that the current is flowing in the clockwise direction .)
2√3×10−5T, inside the plane of triangle
2√3×10−7T , outside the plane of triangle
3×10−7T ,outside the plane of triangle
3×10−5T, inside the plane of triangle
The correct Answer is:4
To find the magnetic field at the centroid of an equilateral triangle carrying a current, we can follow these steps:
1. Identify the Triangle and Current:
We have an equilateral triangle with each side measuring 9 cm. The current of 1.5 A flows in a clockwise direction around the triangle.
2. Determine the Centroid:
The centroid of an equilateral triangle is located at a distance of h3 from the base, where h is the height of the triangle. The height h can be calculated using the formula:
h=√32×a
where a is the side length. For our triangle:
h=√32×9 cm=9√32 cm
3. Calculate the Distance from Each Side to the Centroid:
The distance from each side of the triangle to the centroid is h3:
d=h3=9√36=3√32 cm
4. Use the Formula for Magnetic Field:
The magnetic field B at the centroid due to a straight current-carrying wire is given by:
B=μ0I4πd
However, since we have three sides contributing to the magnetic field, we multiply this by 3:
B=3×μ0I4πd
5. Substitute Values:
Here, I=1.5A and d=3√32 cm=3√3200 m (since we need to convert cm to m). The permeability of free space μ0=4π×10−7T⋅m/A.
B=3×4π×10−7×1.54π×3√3200
6. Simplify the Equation:
The 4π cancels out:
B=3×10−7×1.53√3200=3×10−7×1.5×2003√3=10−7×300√3
7. Calculate the Final Value:
B=300×10−7√3≈173.21×10−7T=1.732×10−5T
8. Determine the Direction of the Magnetic Field:
Using the right-hand rule, since the current is flowing in a clockwise direction, the magnetic field at the centroid will be directed into the plane of the paper.
Final Answer:
The magnetic field at the centroid of the triangle is approximately 1.732×10−5T directed into the plane of the paper.
We have an equilateral triangle with each side measuring 9 cm. The current of 1.5 A flows in a clockwise direction around the triangle.
The centroid of an equilateral triangle is located at a distance of h3 from the base, where h is the height of the triangle. The height h can be calculated using the formula:
h=√32×a
where a is the side length. For our triangle:
h=√32×9 cm=9√32 cm
The distance from each side of the triangle to the centroid is h3:
d=h3=9√36=3√32 cm
The magnetic field B at the centroid due to a straight current-carrying wire is given by:
B=μ0I4πd
However, since we have three sides contributing to the magnetic field, we multiply this by 3:
B=3×μ0I4πd
Here, I=1.5A and d=3√32 cm=3√3200 m (since we need to convert cm to m). The permeability of free space μ0=4π×10−7T⋅m/A.
B=3×4π×10−7×1.54π×3√3200
The 4π cancels out:
B=3×10−7×1.53√3200=3×10−7×1.5×2003√3=10−7×300√3
B=300×10−7√3≈173.21×10−7T=1.732×10−5T
Using the right-hand rule, since the current is flowing in a clockwise direction, the magnetic field at the centroid will be directed into the plane of the paper.
The magnetic field at the centroid of the triangle is approximately 1.732×10−5T directed into the plane of the paper.
Topper's Solved these Questions
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics Section B
20 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section A)
81 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - PHYSICS
30 Videos
- JEE MAINS 2020BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2020EXERCISE - PHYSICS
246 Videos
- JEE MAINS 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS (SECTION -B)
10 Videos
Similar Questions
A current of 1A is flowing in the sides of equilateral triangle of side 4.5×10−2m. The magnetic induction at centroid of the triangle:
View SolutionA current of 1⋅0A flowing in the sides of an equilateral triangle of side 4⋅5×10−2m. Find the magnetic fied at the centroid of the triangle.
View Solution
Knowledge Check
A current of 2A is flowing in the sides of an equilateral triangle of side 9 cm. The magnetic field at the centroid of the triangle is
A1.66×10−6TB1.22×10−4TC1.33×10−6TD1.44×10−4TA current of 10A is flowing in a equilateral triangle of side length l=1M as shown in figure. The magnetic field at center of triangle is:
_2019_CBT6_E01_040_Q01.png) A8×10−6TB9×10−5TC9×10−6TD10−5T
A8×10−6TB9×10−5TC9×10−6TD10−5TThe current I ampere is flowing in an equilateral triangle of side a the magnetic field induction at the centroid will be
A9μ0I2πaB5√2μ0I3πaC3μ0I2πaDμ0I3√3πa
An equilateral triangle of side 10cm carries a current of 3A. Find the magnetic field at centroid of triangle.
View SolutionFind the magnetic field at the centroid of an equilateral triangle of side0.03 m and a current 2 A flowing through the sides of it.
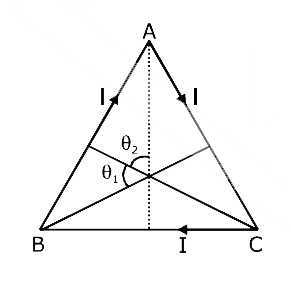
View SolutionA current I is flowing in a hexgonal coil of side a figure. The magnetic field induction at the centre O of the coil will be
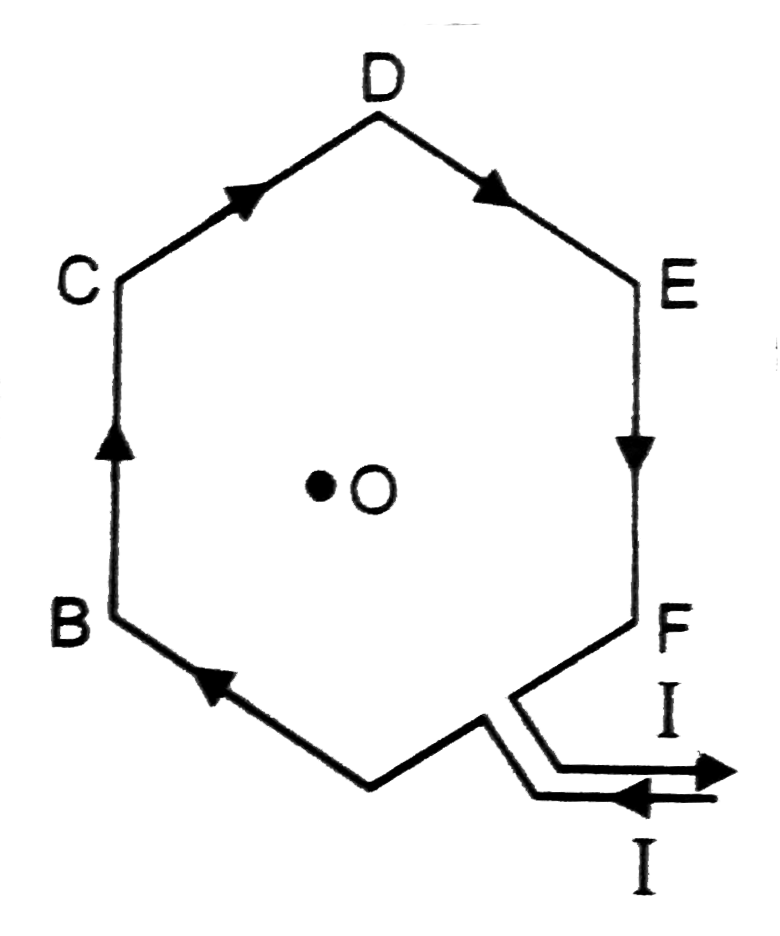
View SolutionA current 1 flows along a triangle loop having sides of equal length a. The strength of magnetic field at the centre of the loop is :
View SolutionA current I is flowing in an octagonal coil of side a figure. The magnetic field induction at the centre O of the coil will be
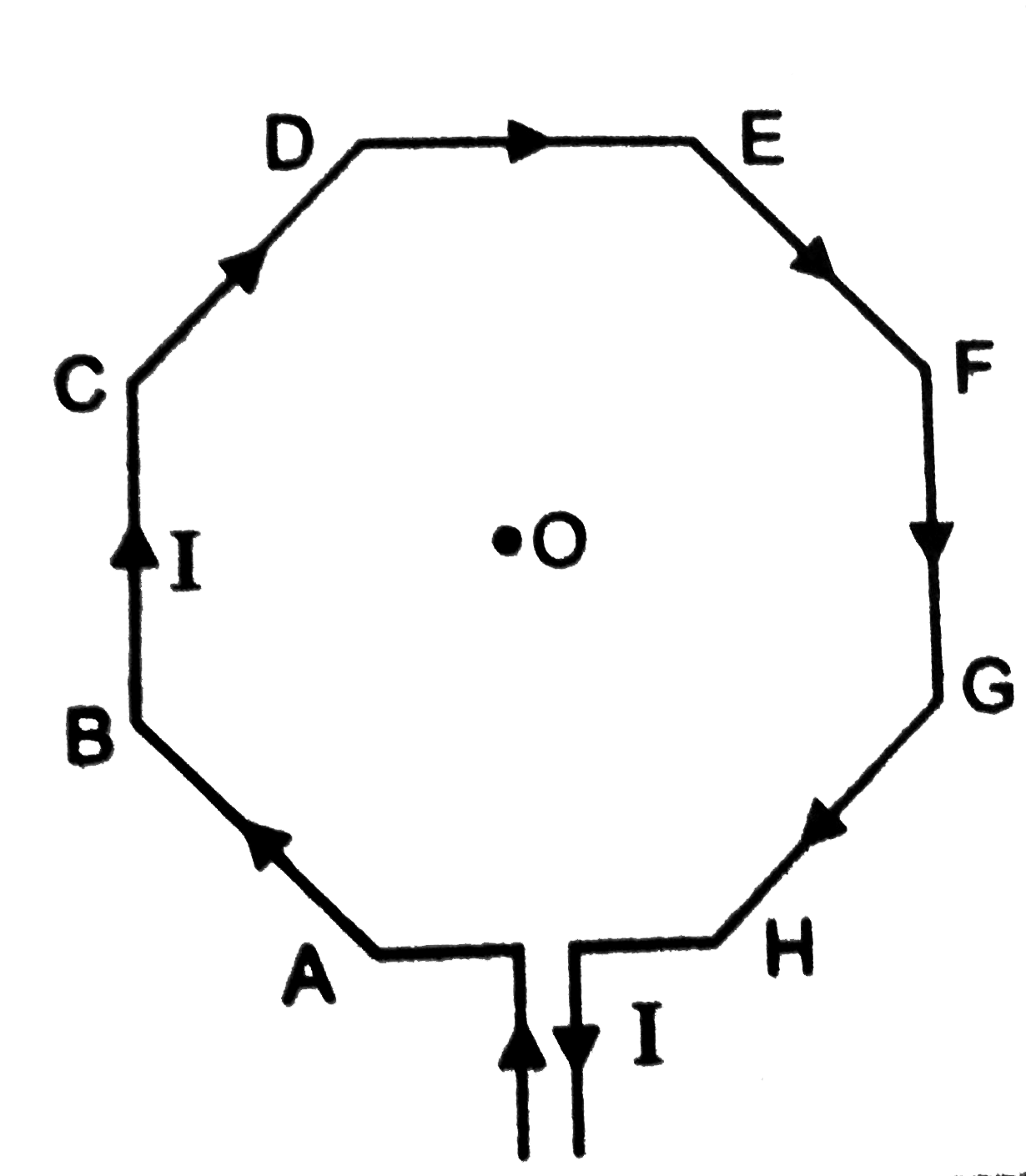
View Solution
