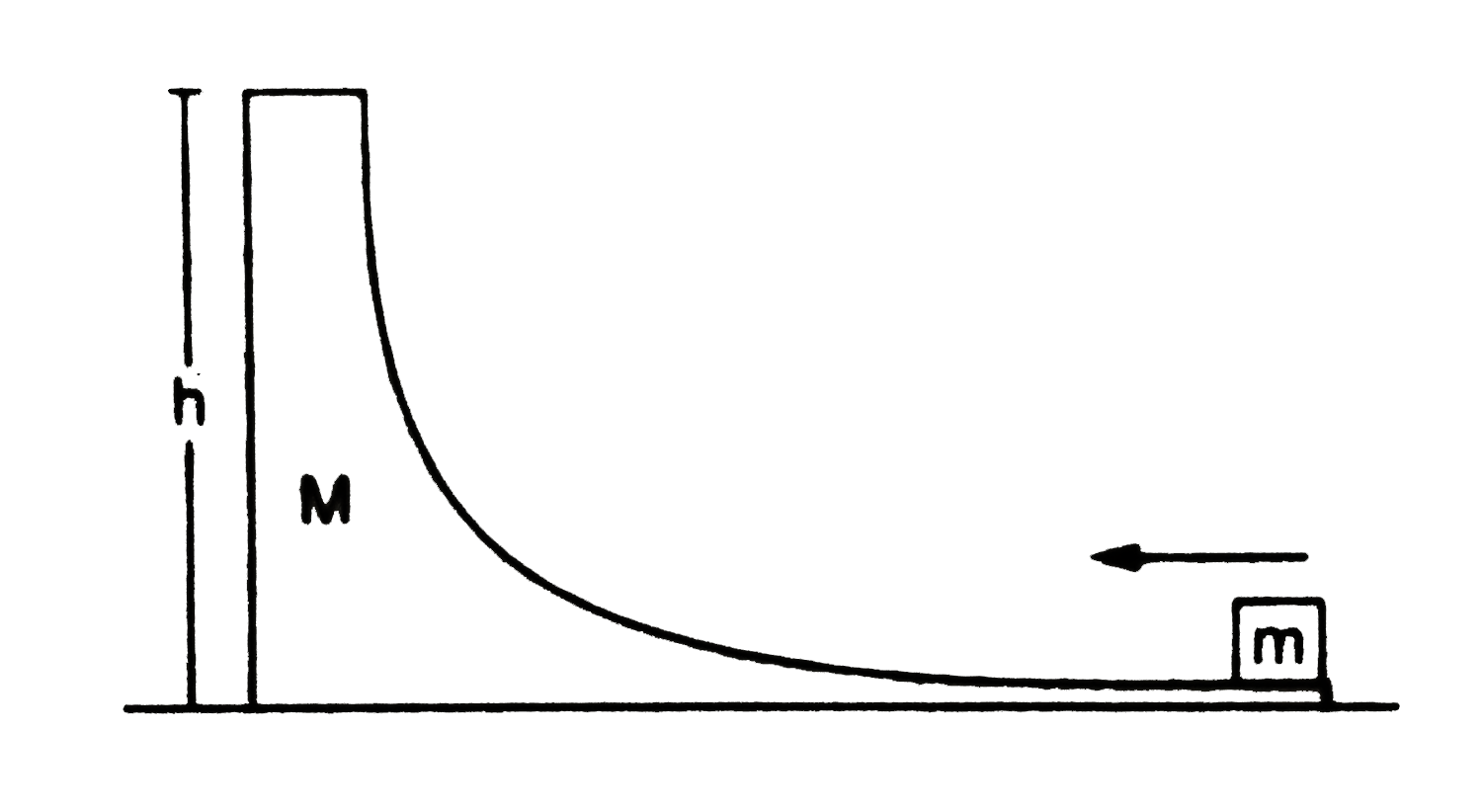
Figure shows a small body of mass m placed over a larger mass M whose surface is horizontal near the smaller mass and gradually curves to become verticle. The smaller mass is pushed on the longer on at a speed v and the system is left to itself. Assume thast all the surfaces are frictionless. a. find teh speed of the larger block when the smaller block is sliding on the vertical part. b. find the speed of the smaller mass when it breaks off the larger mas at height h. c. find the maximum height (from the ground) that the smaller mass seconds. d show that the smaller mass will again land on the bigger one. Find the distane traversed by the bigger block during the time when the smaller block was in its flight under gravity.
 Solution in Tamil
Solution in Tamil
The correct Answer is:A, B, C, D
The mass m is given a velocity v over the larger mass M.
a. when the smaller block is travelling on the vertical part let the velocilty of the bigger block be v1 towards left
From law of conservation of momentum (in the horizontal direction)
mv=(M+m)v1
→v1=mvM+m
b. When the smaller block breaks off let its result velocity is v2
From law a conservation of energy
(12)mv2=(12)Mv21+(12)mv22+mgh
→v22=v2−Mmv21−2gh......i
→v22=v62[1−Mm,m2(M+m)2]−2gh
→v2=[(m2+Mm+m2)(M+m)2]−2gh]120
c. now the vertical component of the velocity v2 of mass m is given by
v_y^2=v_2^I2-v_1^2<br>=((M^2+Mm+m^2)/(M+m)v^2-2gh-(m^2v^2)/((M+m)^2)<br>[:. v_1=(mv)/(M+m)]<br>rarr v_y^2=(M^2+Mm+m^2-m^2)/(M+m) v^2-gh<br>rarr v_y^2=(Mv^2)/((M+m))-2gh.........ii<br>→f∈dthemaxiμmheightomthegroudn,<br>Letusas∑ethebodyrise→aheighthoveraddaboveh<br>now, (1/2)mv_y^2=mgh_1<br>rarr h_1=v_y^2/(2g).........iii<br>so→talheight=h+h_1<br>=h+v_y^2/(2g)<br>=h+(Mv^2)/((M+m)2g)-h.....iii<br>[omequationiiandiii]<br>rarr H=(Mv^2)/(2g(M+m))<br>d.∵thesmal≤rmasshasalsogotahorizontalcompo≠tnofvelocityVatthetimeitbreaksoffomM(whichhasavelocityv_1)theblockmwillaga∈landontheblockM(big≥ro≠)<br>Letusf∈doutthetimeofflightofblockmafteritbreaksoff.<br>Dur∈gtheupwardmotion(BC)<br>0=v_y-gt<br>rarr g_1=v/g<br>=1/g[(Mv^2)/((M+m)-2gh]..........iv<br>[omequationii]<br>Sothetimeforwhichthesmal≤rblockwas∈flightisgivenby<br>T=2t_1<br>=2/g[(Mv^2-2(M+m)gh)/(M+m)]<br>sothedistancetrvel≤dbythebig≥rblockdur∈gthistimeis<br>S=v_1T<br>>=(mv)/(M+m) 2/g xx[(Mv^2-(M+m)gh)]^(1/2)/((M+m)^(1/2)<br>so S=(2gv[Mv^2-2(M+m)gh)]^(1/2)/(g(M+m)^(3/2))
Topper's Solved these Questions
Similar Questions
The centre of mass of a system of particles is at the origin. It follows that:
03:54View SolutionAn aluminium vessel of mass 0.5kg contains 0.2kg of water at 20∘C A block of iron of mass 0.2kgat100∘C is gently put into the water .Find the equilibrium temperature of the mixture, Specific heat capacities of aluminium , iron and water are 910Jkg−1K−1470Jkg−1K−1and4200Jkg−1K−1 respectively
02:25View SolutionFind the accelerationof the moon with respect to the eath from the following data, Distance between the earth and the moon =3.85×105 km and the time taken by the moon to complete one revolution around the earth =27.3days
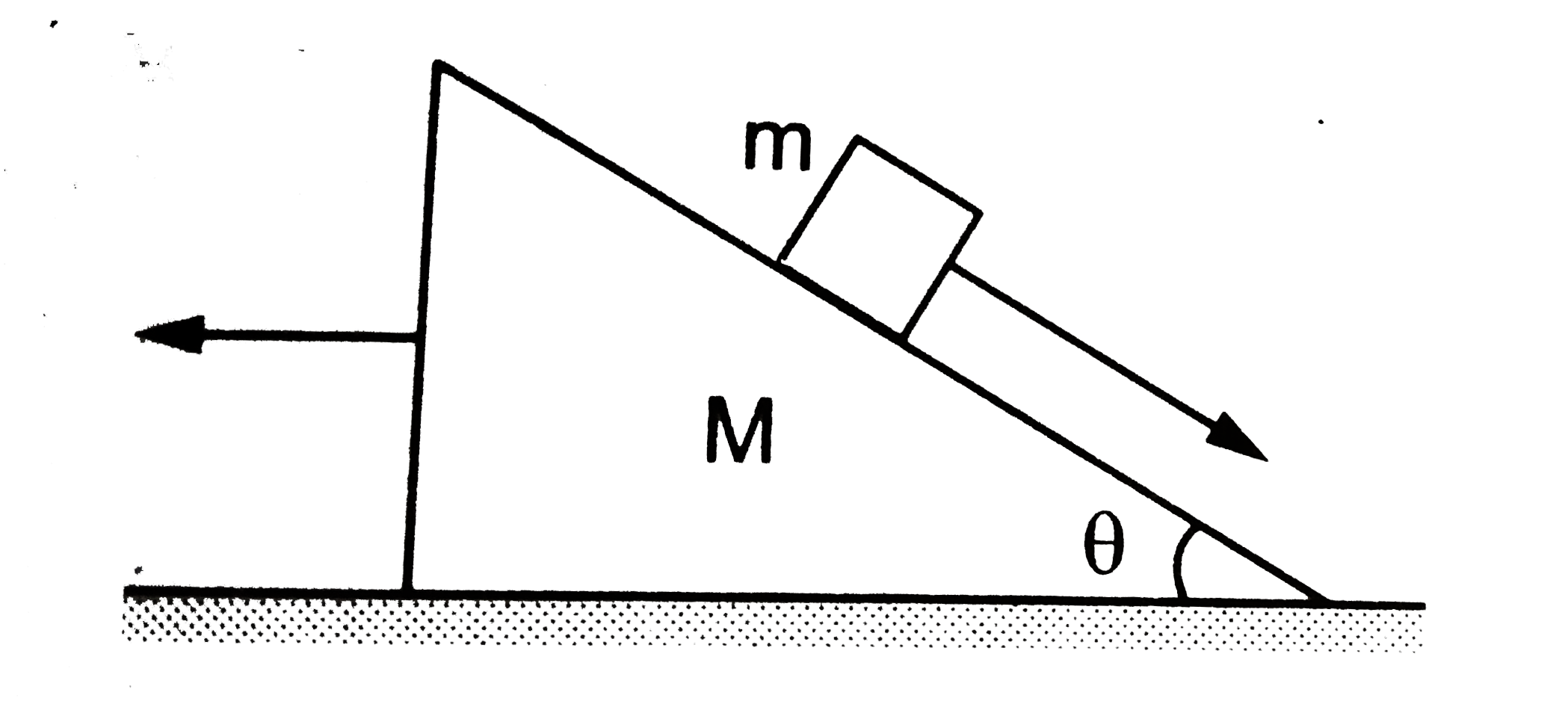
03:03View SolutionAll the surfaces shownin figure are assumed to be frictionless. The block of mass m slides on the prism which in turn slides backwasrd on the horizontal surface. Find the aceleration of the smaller block with respect to the prism.
 04:47View Solution
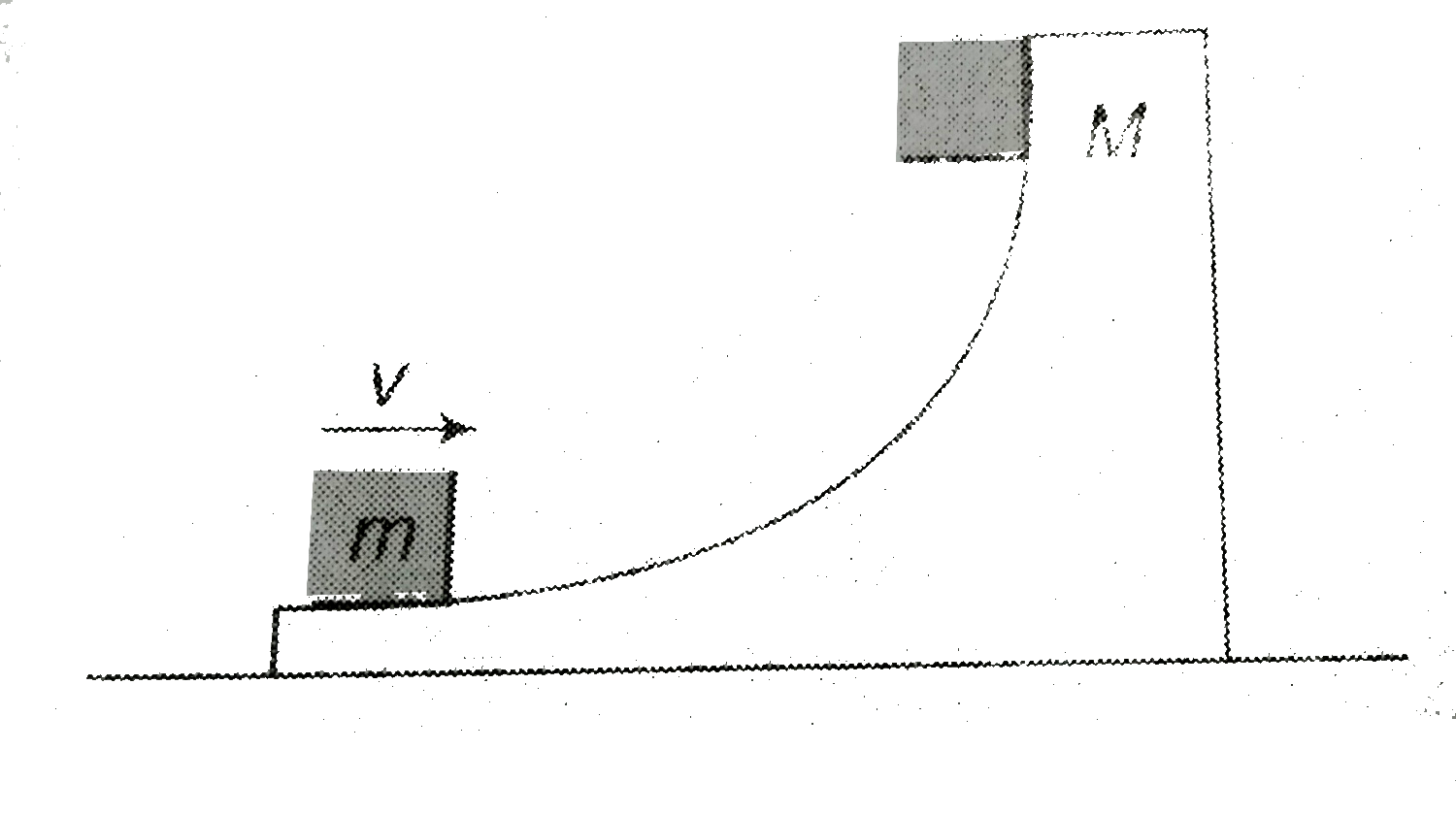
04:47View SolutionFigure shows a small block of mass m which is started with a speed v on the horizontal part of the bigger block of mas m placed on a horizontal floor. The curved part of the surface shown is semicircular. All the surfaces are frictionless. Find the speed of the bigger block when the smaller block reaches the point A of the surface.
 03:50View Solution
03:50View SolutionAs shown , a small body of mass 50g placed over a larger mass 1950g whosw surface is horizontal near the smaller mass and gradually curves to become vertical. The smaller mass is pushed on the longer one at a speed 20m/s and the system is left to itself. All surfaces are smooth. Find the speed of the larger block when the smaller block is moving on the vertical part.
 04:34View Solution
04:34View SolutionAssuming all the surfaces to be smooth, find the acceleration of the triangular block of mass M when the block of mass m slides on it.
 05:04View Solution
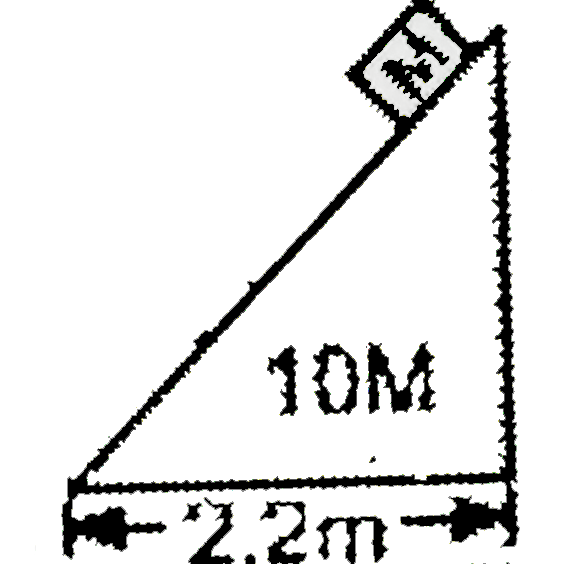
05:04View SolutionA block of mass M is placed on the top of a bigger block of mass 10 M as shown in figure. All the surfaces are frictionless. The system is released from rest, then the distance moved by the bigger block at the instant the smaller block reaches the ground :
 14:43View Solution
14:43View SolutionFind the mass of the hanging block in which will prevent the smaller block of mass m from slipping over the triangular block of mass M. All the surfaces are frictionless and the strings and the pulleys are light
 02:54View Solution
02:54View Solutionचित्र में एक M द्रव्यमान का बड़ा गुटका एक क्षैतिज सतह पर रखा हुआ दिखाया गया है। इसके क्षैतिज भाग पर द्रव्यमान का एक छोटा गुटका चाल से गति करना प्रारंभ करता है। चित्र में प्रदर्शित सतह का वक्राकार भाग अर्द्धवृत्ताकार है। सभी सतहों को घर्षणरहित मानें। जब छोटा गुटका सतह के बिंदु A पर पहुँचता है उस समय बड़े गुटके की चाल ज्ञात करें।
 06:53View Solution
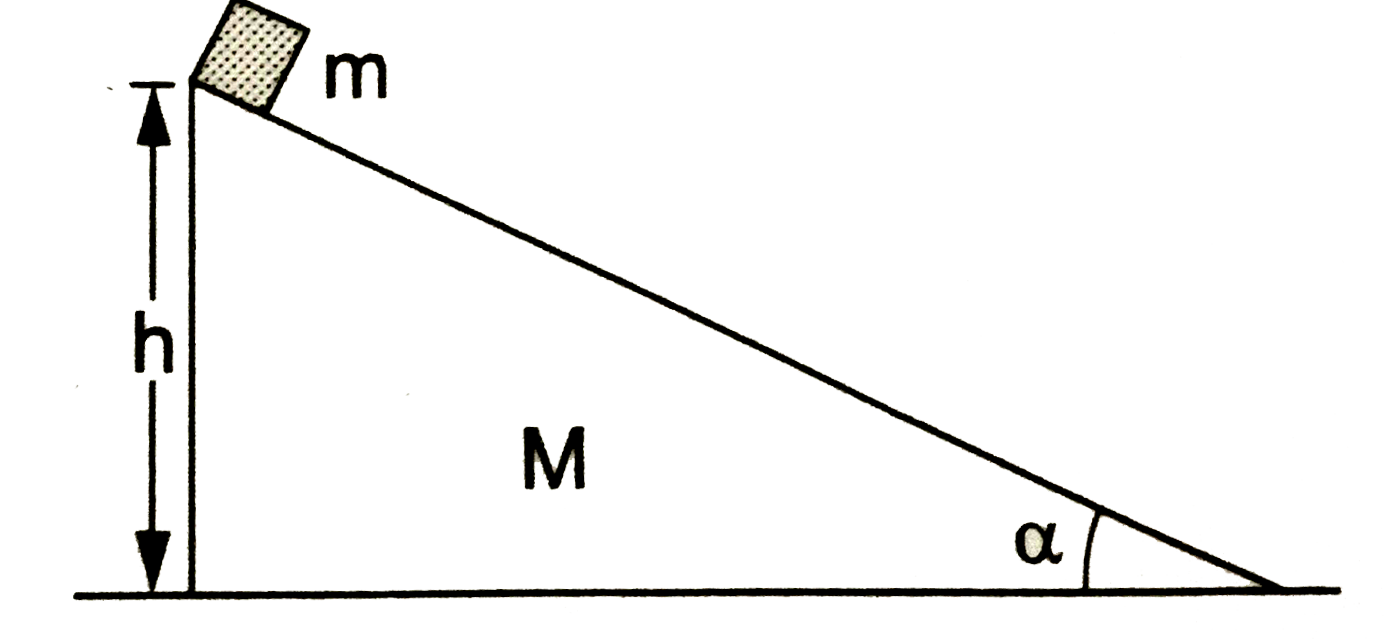
06:53View Solutionm द्रव्यमान का एक गुटका M द्रव्यमान के एक त्रिभुजाकार गुटक पर रखा हुआ है, जो स्वयं एक क्षैतिज सतह पर रखा गया है चित्र
जब छोटा गुटका क्षैतिज सतह पर पहुँचता है, उस समय त्रिभुजाकार गुटके का वेग ज्ञात करें। सभी सतहों को घर्षणरहित मानें।
 View Solution
View Solutionm द्रव्यमान का एक छोटा गुटका M द्रव्यमान के एक बड़े गुटके पर रखा हुआ है। इस बड़े गुटके की सतह दाहिने किनारे के पास क्षैतिज है तथा धीरे-धीरे मुड़ती हुई ऊर्ध्वाधर हो जाती है। बड़ा गुटका एक क्षैतिज सतह पर फिसल सकता है। छोटे गुटके को बड़े गुटके पर v चाल से चलाकर निकाय को स्वतंत्र छोड़ दिया जाता है। सभी सतहों को घर्षणरहित मानें।
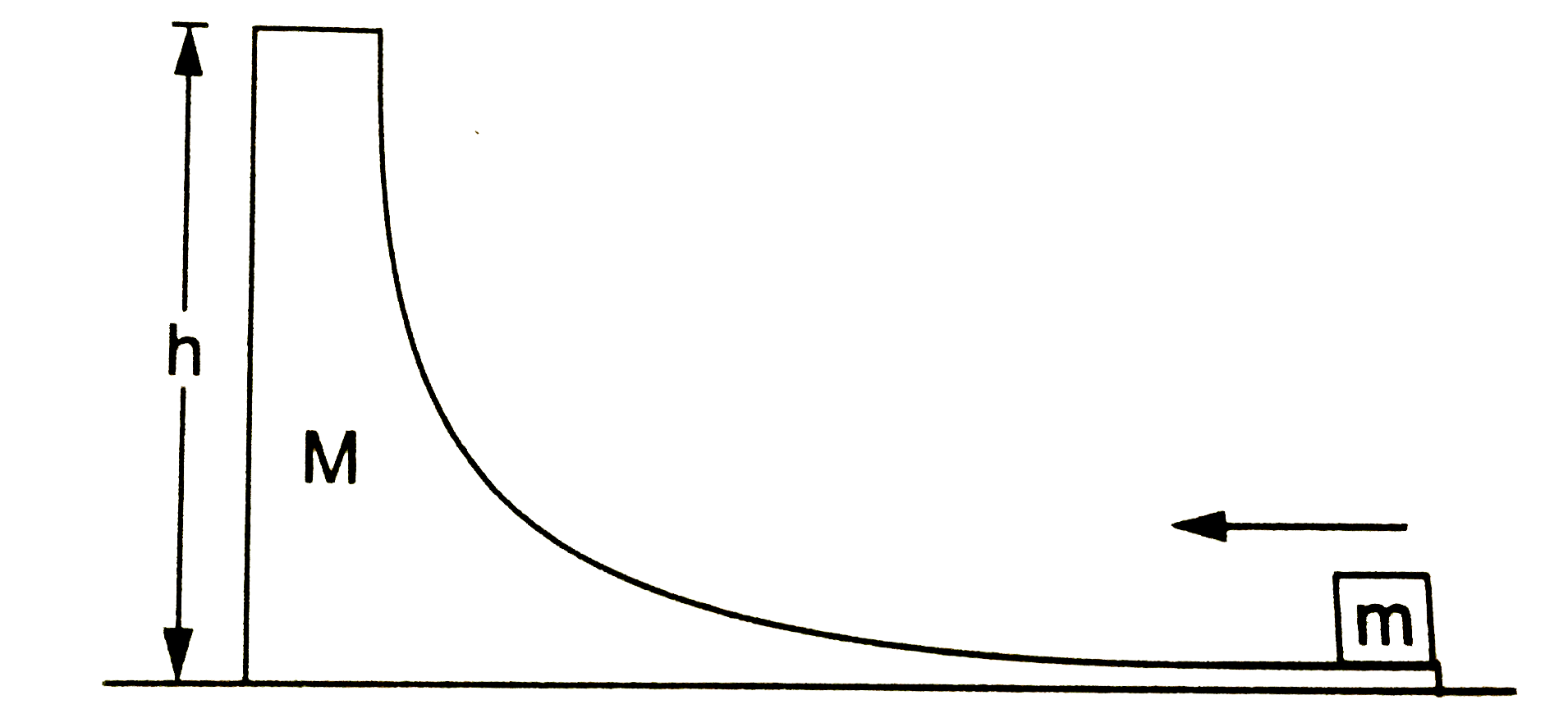
जब छोटा गुटका ऊर्ध्वाधर भाग पर पहुँचकर फिसल रहा हो, उस समय बड़े गुटके की चाल ज्ञात करें।06:50View Solutionm द्रव्यमान का एक छोटा गुटका M द्रव्यमान के एक बड़े गुटके पर रखा हुआ है। इस बड़े गुटके की सतह दाहिने किनारे के पास क्षैतिज है तथा धीरे-धीरे मुड़ती हुई ऊर्ध्वाधर हो जाती है। बड़ा गुटका एक क्षैतिज सतह पर फिसल सकता है। छोटे गुटके को बड़े गुटके पर चाल से चलाकर निकाय को स्वतंत्र छोड़ दिया जाता है। सभी सतहों को घर्षणरहित मानें।
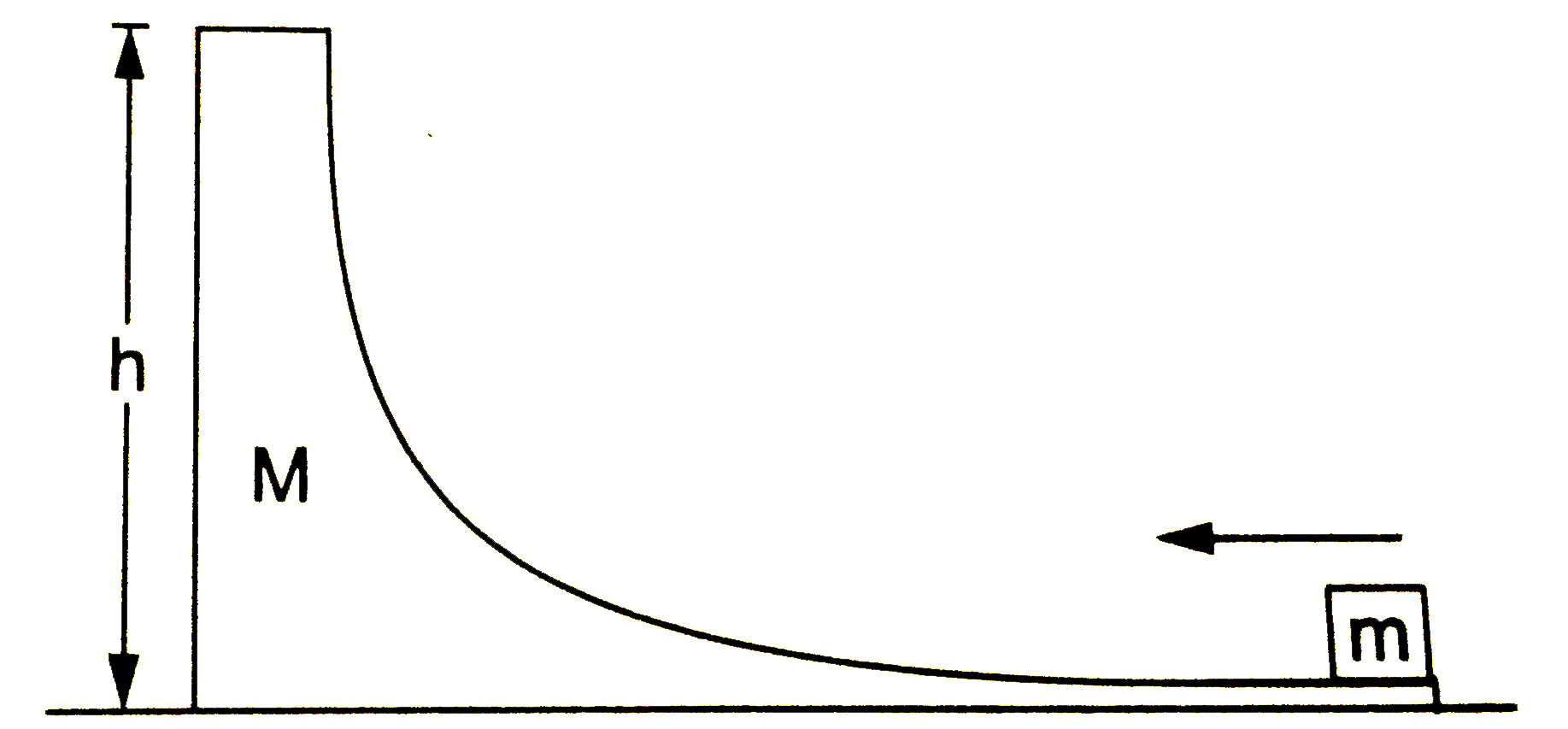
छोटे गुटके द्वारा तय की गई अधिकतम ऊँचाई ज्ञात कीजिए।03:56View SolutionA prism of mass M is placed on a horizontal surface. A block of mass m slides on the prism, which in tum slides on the horizontal surface. Assuming all surfaces to be frictionless, find the acceleration of block with respect to prism.
 02:58View Solution
02:58View SolutionAll the surfaces shown in figure are assumed to be frictionless. The block of mass m slides on the prism which in turn slides backward on the horizontal surface. Find the acceleration of the smaller block with respect to the prism.
 View Solution
View SolutionA block of mass M is placed on the top of a bigger block of mass 10 M as shown in figure. All the surfaces are frictionless. The system is released from rest, then the distance moved by the bigger block at the instant the smaller block reaches the ground :
 View Solution
View Solution

