A tunnel is dug along a chord of the earth a perpendicular distance $\frac{R}{2}$ from the earth's centre. The wall of the tunnel may be assumed to be frictionless. Find the force exerted by the wall on a particle of mass m when it is at a distance x from the centre of the tunnel.Solution in Tamil

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let the distance from center of earth to mass m then
$\to d^{2} = x^{2} = (\frac{R^{2}}{4}) = \frac{4 x^{2} + R^{2}}{3}$
$\to d = (\frac{1}{2}) \sqrt{4 x^{2} + R^{2}}$
M be mass of the earth $m^{1}$ , the mass of the sphere of radius $\frac{d}{2}$
$t h e n M (\frac{4}{3}) π R^{3} ρ$
$M^{1} = (\frac{4}{3}) π d^{3} ρ$
$or \frac{M^{1}}{M} = \frac{d^{3}}{R^{3}}$

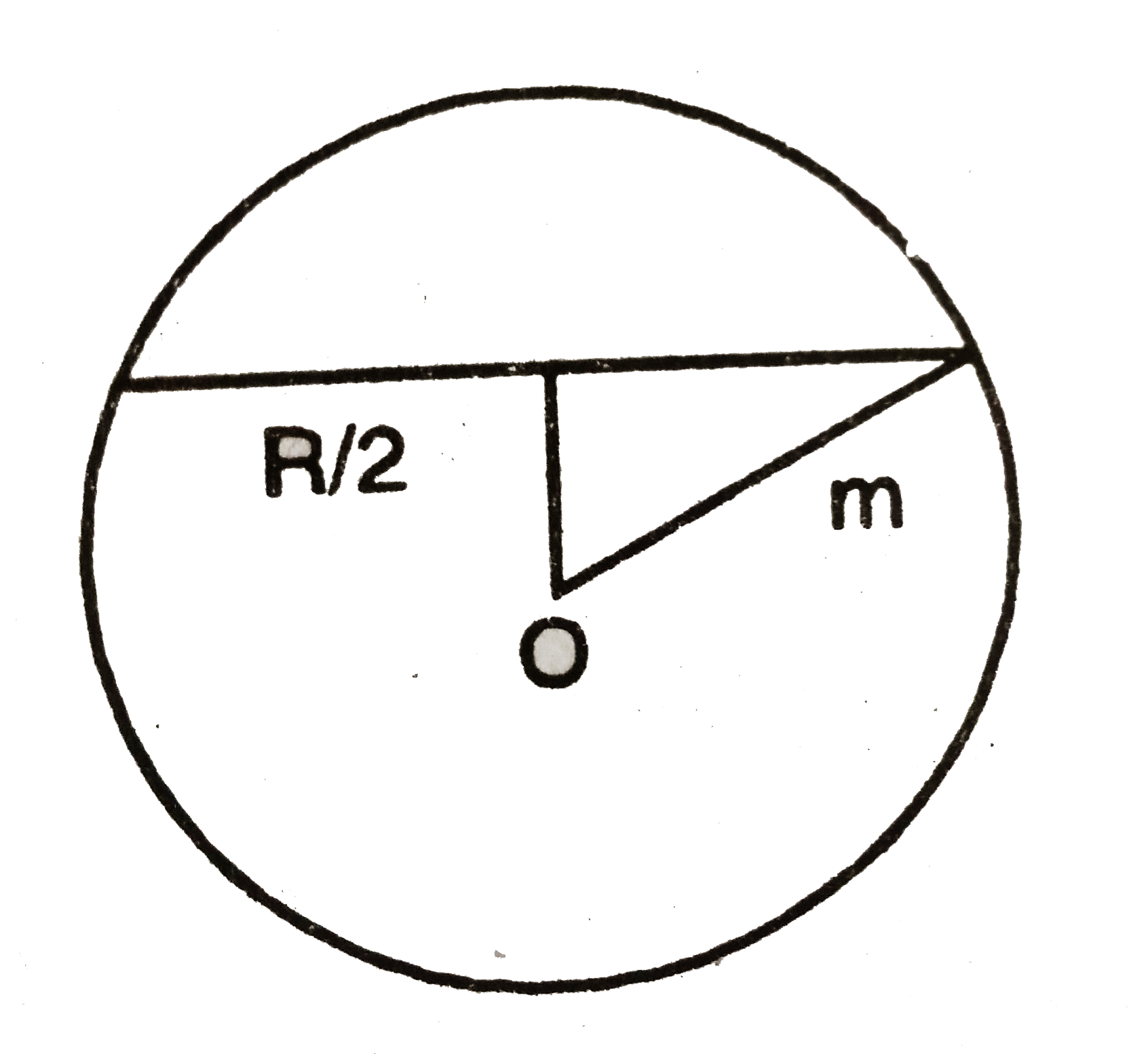
$∴$ GravitatioN/Al force in m,
$F = \frac{G M^{1} m}{d^{2}}$
$= \frac{G d^{2} M m}{R^{3} d^{2}} = \frac{G m m}{R^{3}} d$
NOrmal force exerted by the wall
$= F cos θ$
$= \frac{G M m d}{R^{3}} \times \frac{R}{2 d} = \frac{G M m d}{2 R^{2}}$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

HC VERMA-GRAVITATION-Exercises

Home
Ask Doubt
Profile