Two steel rods and an aluminum rod of equal length l0 and equal cross section are joined rigidly at their ends as shown in the figure below. All the rods are in a state of zero tension at 0∘C. Find the length of the system when the temperature is raised to θ. Coefficient of linear expansion of aluminum and steel are αaandαs respectively. Young's modulus of aluminum is Ya and of steel is Ys.
 Solution in Tamil
Solution in Tamil
Let the initial length of the system at 0∘C
=l0
When temperature changes by θ
Final length of the steel at system temperature.
So, strain of the system=l−(l0)l0
Steel
Aluminium
Steel
But the total strain of the system
= total stress of system/ total Young's modulus of system
Now, total stress due to two steel rod
+ stress due to aluminium
=(γsαsθ)+(γsαsθ)+((γAl)(αAl)θ)
=(2γsαsθ)+(γAlαAlθ)
Now Young's modulus system
=γs+γs+γAl=(2γs+γAl
∴Stra∈ofsystem=(2γsαsθ)+γAlαAlθ2γs+γAl
l−ll0=(2γsαsθ)+(γAlαAlθ)2γs+γAl
l= (l_0)[ 1+((2gamma_s alpha_s theta)+(2gamma_(Al) alpha_(Al) theta) / (2 gamma_s+ gamma_(Al))
Topper's Solved these Questions
Similar Questions
A spinning wheel is brought in contact with an identical wheel spinning identical speed. The wheels slow down under the action of friction. Which of the following energies of the frist wheel decrease.
View SolutionTwo spherical balls of mass 10 hg are placed 10 cm apart. Find gravitational force of attraction between them
View SolutionA uniform slab of dimension 10cm×10cm×1cm is kept between two heat reservoir at temperatures 10∘C and 90∘C. The larger surface areas touch the reservoirs. The thermal conductivity of the material is 0.80Wm−1C−1 . Find the amount of heat flowing through the slab per minute.
View SolutionTwo Aluminium rods and a steel rod of equal cross-sectional area and equal length l_(0) are joined rigidly side by side as shown in figure. Initially the rods are at 0^(@)C . Find the length of the rod at the temperature theta if young's modulus of elasticity of the aluminium and steel are Y_(a) and Y_(s) respectively and coefficient of linear expansion of aluminum and steel are alpha_(a) and alpha_(s) respectively. |{:("Aluminium"),("Steel"),("Aluminium"):}|
View SolutionAn iron rod of length 50 cm is joined at an end to an aluminum rod of length 100 cm. All measurements refer to 20^(@)C . The coefficients of linear expansion of iron and aluminum are 12xx10^(-6)//^(@)C and 24xx10^(-6)//^(@)C , respectively. The average coefficient of expansion of composite system is :
View SolutionA meter scale calibarated for temperature T is used to measure the length of a steel rod. Length of steel rod measured by metal scale at temperature 2T is L. Length of the rod measured by metal scale at temperature T is [αs:Coefficient of linear expansion of metal scale, αR : Coefficient of linear expansion of steel rod]
View SolutionTwo same length rods of brass and steel of equal cross-sectional area are joined end to end as shown in figure and supported between two rigid vertical walls. Initially the rods are unstrained. If the temperature of system is raised by Δt. Find the displacement of the junction of two rods. Given that the coefficient of linear expansion and young's modulus of brass and steel are aphab.αs(αb>αs),Yb and Ys respectively.
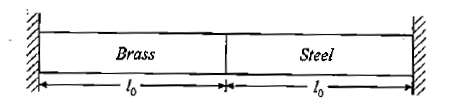
View Solutionदो छड़े एक l1 की एल्युमिनियम छड़ एवं दूसरी l2 लम्बाई की स्टील छड़, एक साथ जोड़ी गई हैं, संयुक्त छड़ की लम्बाई l1+l2 है। एल्युमीनियम एवं स्टील के रेखीय प्रसार गुणांक क्रमशः αa एवं αs है। जब संयुक्त छड़ का ताप t.∘C से बढ़ाया जाता है तो प्रत्येक छड़ की लम्बाई वृद्धि समान है तब अनुपात l1(l1+l2) है
View SolutionTwo rods of equal length and cross- sectional area have their Young's moduli Y_1 and Y_2 respectively. IF the rods are joined end to end, then the equivalent Young's modulus of the combined rod system is
View SolutionA steel rod of length L_0 . has a cross sectional area A. The force required to stretch this rod by the same amount as the expansion produced by heating it through DeltaT is (coefficient of linear expansion of steel is alpha and young's modulus for steel is Y ).
View SolutionWhat should be the lengths of steel and copper rod so that the length of steel rod is 10 cm longer than the copper rod at all the temperatures. Coefficients of linear expansion for copper and steel are αCu=1.7×10−5 0C−1andαsteel=1.1×10−5∘C−1
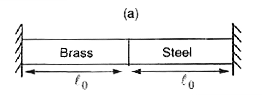
View SolutionTwo rods of same length made of brass and steel of equal cross-sectional area are joined end to end as shown in figure (a) and supported between two rigid vertical walls. Initially the rods are unstrained. If the temperature of system is raised by ΔT, find the displacement of the junction of the rods. Given that the coefficients of linear expansion and Young.s modulus of brass and steel are αb,αs(αb>αs),ybandys respectively.

View SolutionTwo steel rods and an aluminium rod of equal length l_(0) and equal corss-sectional area are joined rigidly at their ends. All the rods are in state of zero tension at 0^(0)C . Find the length of the system when the temperature is raised to theta . Coefficient of liner expansion of aluminium and steel are alpha_(a) and alpha_(s) respectively Young.s modulus of aluminium and steel are Y_(a) and Y_(s) .
View SolutionTwo rods of same length made of brass and steel of equal cross-sectional area are joined end to end as shown in figure ( a) and supported between two rigid vertical walls. Initially the rods are unstrained. If the temperature of system is raised by ΔT, find the displacement of the junction of the rods. Given that the coefficients of linear expansion and Young's modulus of brass and steel are αb,αs(αb>αs),ybandys respectively.
View Solutionएक इस्पात की छड़ तथा एक अन्य एल्युमीनियम की छड़ का तापमान 0∘C से 100∘C तक बढ़ाने पर दोनों छड़ों की लम्बाईयों में समान वृद्धि होती है। यदि इस्पात की छड़ की लम्बाई 1 मी है तो एल्युमीनियम छड़ की लम्बाई लगभग है ( as=12×10−5 प्रति ∘C तथा αA=1.71×10−5 प्रति ∘C)
View Solutionएक इस्पात की छड़ तथा एक अन्य एल्युमीनियम की छड़ का तापमान 0∘C से 100∘C तक बढ़ाने पर दोनों छड़ों की लम्बाईयों में समान वृद्धि होती है। यदि इस्पात की छड़ की लम्बाई 1 मी है तो एल्युमीनियम छड़ की लम्बाई लगभग है ( as=12×10−5 प्रति ∘C तथा αA=1.71×10−5 प्रति ∘C)
View Solution
