Two identical thin rings each of radius R are coaxially placed at distance R apart . If Q1andQ2 are the charges uniformly spread on the two rings , the work done is moving a charge from centre of one ring to the order is :
zero
q(Q1−Q2)(√2−1)√2.4πε0R
q√2(Q1+Q2)4πε0R
q(Q1Q2)(√2+1)√2.4ε0R
The correct Answer is:B
W=q(VA−VB)
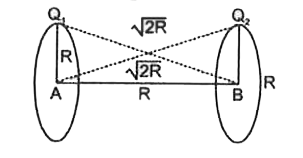
VA=14πε0[Q1R+Q2√2R]
VB=14πε0[Q2R+Q1√2R]
Then W=q(Q1−Q2)√2.4πε0R.(√2−1) .
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - LEVEL-III
23 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Recent Competitive Questions
8 Videos
Similar Questions
Two identical thin rings, each of radius R, are coaxially placed a distance R apart. Q1 and Q2 are respectively the charges uniformly spread on the two rings. The work done in moving a charge q fróm the centre of one ring to that of the other is (√x−y)q(Q2−Q1)4√2πɛ0R. Find (x−y)
View SolutionTwo identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If Q1 coulomb, and Q2 coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View Solution
Knowledge Check
Two identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If Q1 coulomb, and Q2 coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
A(a) zeroB(b) q(Q1−Q2)(√2−1)(4√2πε0R)C(c) q√2(Q1+Q2)(4πε0R)D(d) q(Q1+Q2)(√2+1)(4√2πε0R)Two identical thin rings each of radius R are coaxially placed at a distance R. If the rings have a uniform mass distribution and each has masses 2m and 4m respectively, then the work done in moving a mass m from centre of one ring to that of the other is
AzeroB√2Gm2R(1−√2)CGm2√2R(√2−1)DGm2√2RTwo identical thin ring each of radius R are co-axially placed at a distance R. If the ring have a uniform mass distribution and each has mass m1 and m2 respectively, then the work done in moving a mass m from the centre of one ring to that of the other is :
AzeroBGm(m1−m2)(√2−1)√2RCGm√2(m1+m2)RDGm1m(√2+1)m2R
Two identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If Q1 coulomb, and Q2 coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View SolutionTwo identical thin rings each of radius R are coaxially placed at a distance R. If the rings have a uniform mass distribution and each has masses 2m and 4m respectively, then the work done in moving a mass m from centre of one ring to that of the other is
View SolutionTwo identical thin ring each of radius R are co-axially placed at a distance R. If the ring have a uniform mass distribution and each has mass m1 and m2 respectively, then the work done in moving a mass m from the centre of one ring to that of the other is :
View SolutionTwo identical rings PandQ of radius 0.1m are mounted coaxially at a distance 0.5m apart. The charges on the two rings are 2μCand4μC, respectively. The work done in transferring a charge of 5μC from the center of P to that of Q is.
View SolutionTwo identical rings PandQ of radius 0.1m are mounted coaxially at a distance 0.5m apart. The charges on the two rings are 2μCand4μC, respectively. The work done in transferring a charge of 5μC from the center of P to that of Q is.
View Solution
