A point charge 0.140nC is placed on the apex of a cone of semi-vertical angle 30∘. Find the electric flux (in SI units) through the base of cone.
The correct Answer is:1
ϕ=Q2ε0(1−cosθ)
=0.14×10−92×8.85×10−12[1−√32]
=0.14×10−9×0.133972×8.85×10−12=0.14×133.972×8.85=18.7552×8.85=1.059
Similar Questions
A point charge q is placed on the apex of a cone of semi-vertex angle θ. Show that the electric flux through the base of the cone is q(1−cosθ)/2ε0.
 . View Solution
. View SolutionA point charge q is placed on the apex of a cone of semi-vertex angle θ. Show that the electric flux through the base of the cone is q(1−cosθ)/2ε0.
 . View Solution
. View Solution
Knowledge Check
- Question 1 - Select One
a point charge q is placed at the centre of a cylinder of length L and radius R the electric flux through the curved surface of the cylinder is
Aqε0L√L2+R2Bqε0L√L2+2R2Cqε0L√L2+4R2Dq2ε0 - Question 2 - Select One
A point charge Q is placed at one of the vertices of a cubical block. The electric flux flowing through this cube is
AQ6ε0BQ4ε0CQ8ε0DQε0
A point charge q is placed on the top of a cone of semi vertex angle θ. Show that the electric flux through the base of cone is q(1−cosθ)2ε0
View Solutionpoint charge q is placed on the top of a cone of semi vertex angle theta .The electric flux through the base of the cone is
View SolutionA point charge Q is placed at the centre of a hemisphere. Find the electric flux passing through flat surface of hemisphere.
View SolutionA point charge Q is placed at the centre of a hemisphere. Find the ratio of electric flux passing through curved andplane surface of the hemisphere.
View SolutionA point charge q is placed at a distance d from the centre of a circular disc of radius R. Find electric flux flowing through the disc due to that charge
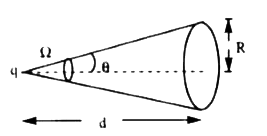 View Solution
View SolutionA point charge q is placed at a distance d form the center of circular disc of radius R. Find electric flux through the disc due to that charge
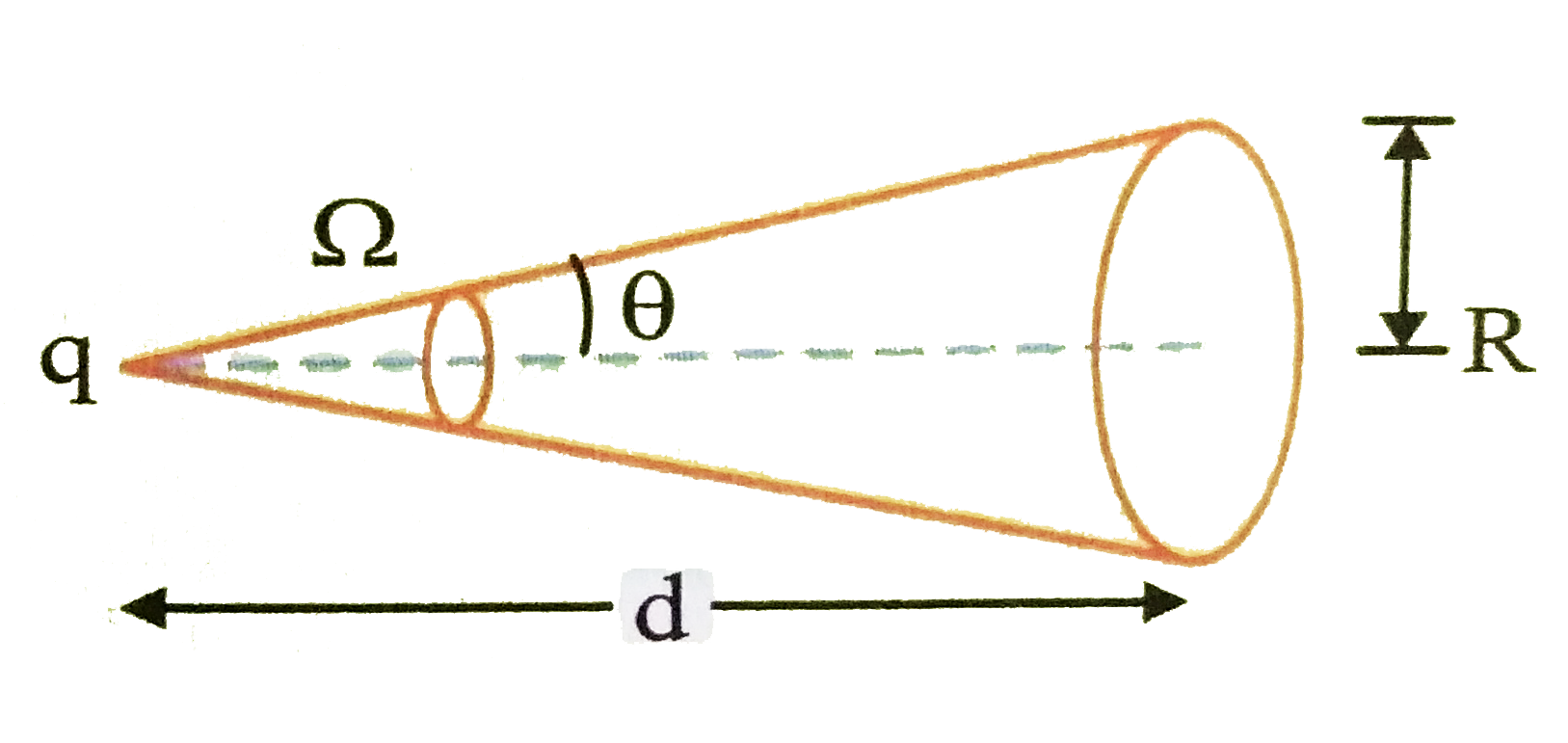 View Solution
View Solution

