The position of a particle moving along the x-axis at certain times is given below:
t(s)0123x(m)−20616
Which of the following describes the motion correctly?
Uniform, accelerated
Uniform, decelerated
Non-uniform, accelerated
There is not enough data for generalization
The correct Answer is:C
Instantaneous velocity v=ΔxΔt
By using the data from the table
v1=0−(−2)1=2m/s
v2=6−01=6m/s
v3=16−61=10m/s
So motion is non-uniform but accelerated.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Position of particle moving along x-axis is given as x=2+5t+7t2 then calculate :
AVelocity (i.edxdt) of particleBInitial velocity(i.edxdt att=0)CVelocity at t=2secDAccleration(i.ed2xdt2) of particleThe position of a particle moving along x-axis is given by x=10t−2t2. Then the time (t) at which it will momentily come to rest is
AZeroB2.5 sC5 sD10 s
The position of a particle along x-axis at certain time is given below
Determine the following
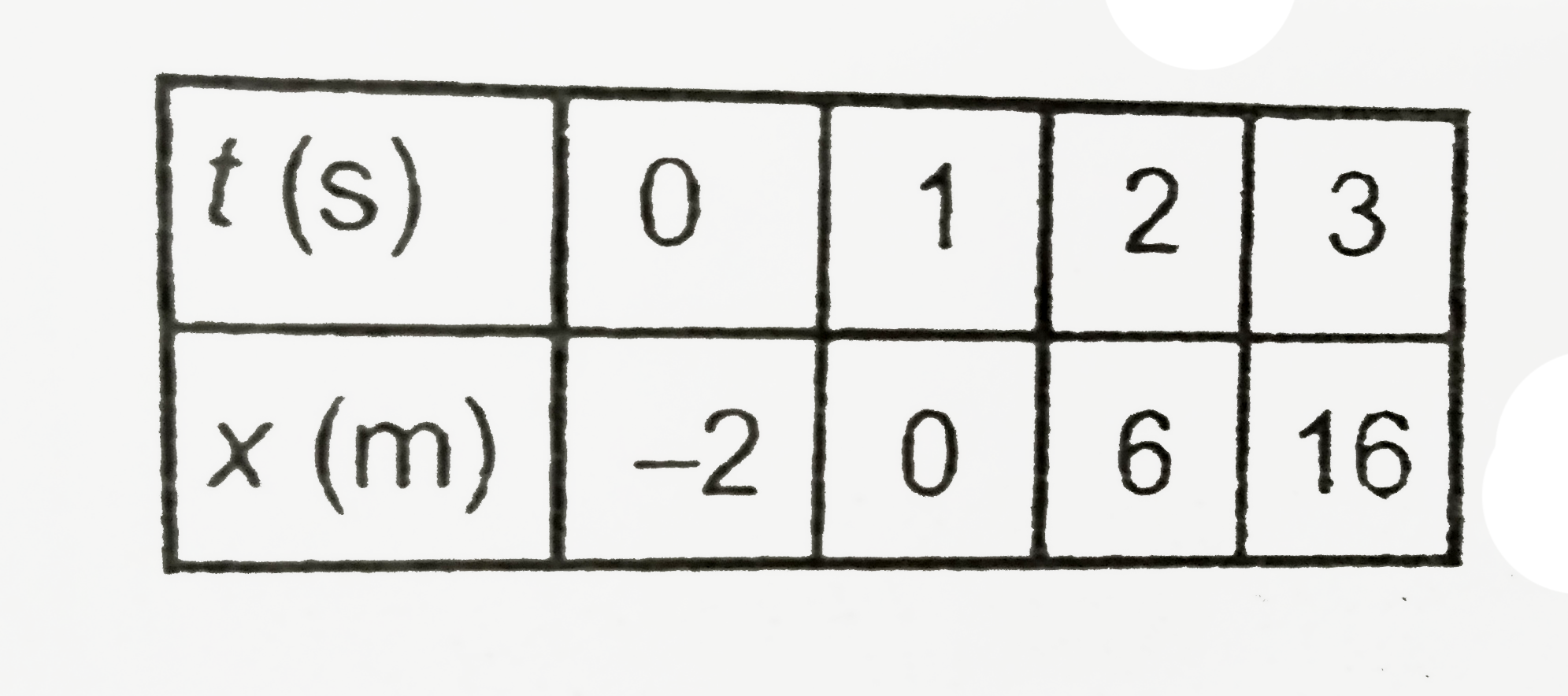
(a) Average velocity of particle in first 3 seconds.
(b) Position of particle at the end of 4th second if particle continue the trend.
(c) Acceleration of particle at time t = 3 s
(d) Type of motion
View SolutionThe position of a particle along x-axis at certain time is given below
Determine the following
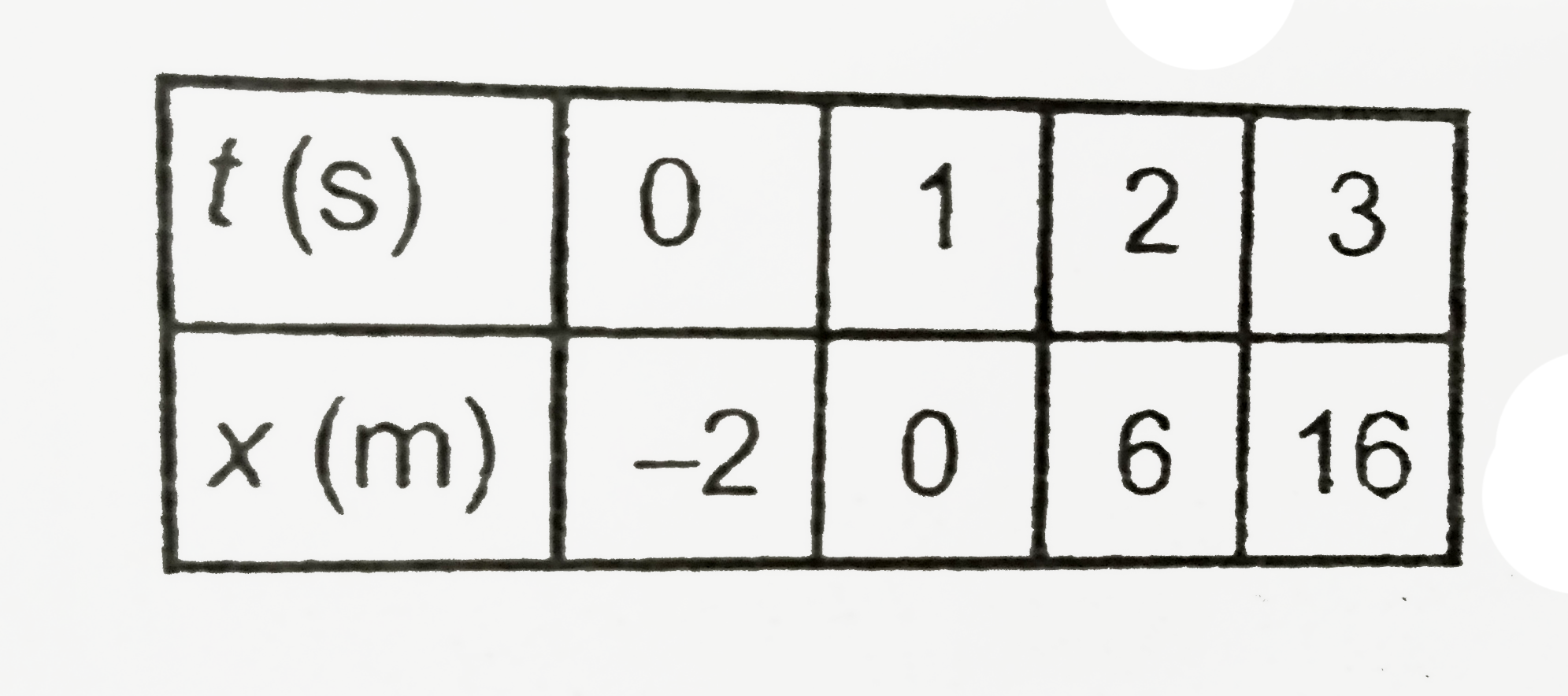
(a) Average velocity of particle in first 3 seconds.
(b) Position of particle at the end of 4th second if particle continue the trend.
(c) Acceleration of particle at time t = 3 s
(d) Type of motion
View SolutionThe position of particle moving along the x-axis veries with time t as x=6t−t2+4. Find the time-interval during which the particle is moving along the positive x-direction.
View SolutionThe position of a particle moving along x-axis is given by x=10t−2t2. Then the time (t) at which it will momentily come to rest is
View SolutionThe position of a body moving along x-axis at time t is given by x=(t2−4t+6)m. The velocity of the body at time t = 3s is
View SolutionThe position (x) of a particle of mass 1 kg moving along x-axis at time t is given by (x=12t2) metre. Find the work done by force acting on it in time interval from t=0 to t=3 s.
View Solution
