A force is linearly increasing with time, on a block of mass m placed on the horizontal surface. The friction coefficient from the block and the ground is u. The graph of friction force and time is drawn. Which of the following graph is correct.




The correct Answer is:d
To solve the problem, we need to analyze the situation step by step.
Step 1: Understand the Forces Acting on the Block
- We have a block of mass m placed on a horizontal surface.
- An external force F is applied to the block, which increases linearly with time. We can express this as F=k⋅t, where k is a constant.
- The friction force f acting on the block opposes the motion and is given by f=μ⋅N, where N is the normal force. For a horizontal surface, N=mg.
Step 2: Calculate the Maximum Static Friction
- The maximum static friction force can be calculated as:
fmax=μ⋅mg
- For example, if μ=0.1 and m=10kg, then:
fmax=0.1⋅10⋅9.8≈0.98N
Step 3: Determine When the Block Starts Moving
- The block will start moving when the applied force F equals the maximum static friction force fmax.
- If F=k⋅t, we set k⋅t=fmax to find the time t when the block starts moving:
t=fmaxk
Step 4: Analyze the Friction Force Over Time
- Before the block starts moving (for t<tmax), the friction force will equal the applied force, which increases linearly.
- Once the block starts moving (for t≥tmax), the friction force becomes kinetic friction, which is constant and equal to fmax.
Step 5: Draw the Graph of Friction Force vs. Time
- For the time interval 0 to tmax, the friction force increases linearly with time.
- After tmax, the friction force remains constant at fmax.
Conclusion: Identify the Correct Graph
- The correct graph of friction force vs. time will show a linear increase until tmax and then a plateau at the maximum static friction force.
- We have a block of mass m placed on a horizontal surface.
- An external force F is applied to the block, which increases linearly with time. We can express this as F=k⋅t, where k is a constant.
- The friction force f acting on the block opposes the motion and is given by f=μ⋅N, where N is the normal force. For a horizontal surface, N=mg.
- The maximum static friction force can be calculated as:
fmax=μ⋅mg
- For example, if μ=0.1 and m=10kg, then:
fmax=0.1⋅10⋅9.8≈0.98N
- The block will start moving when the applied force F equals the maximum static friction force fmax.
- If F=k⋅t, we set k⋅t=fmax to find the time t when the block starts moving:
t=fmaxk
- Before the block starts moving (for t<tmax), the friction force will equal the applied force, which increases linearly.
- Once the block starts moving (for t≥tmax), the friction force becomes kinetic friction, which is constant and equal to fmax.
- For the time interval 0 to tmax, the friction force increases linearly with time.
- After tmax, the friction force remains constant at fmax.
- The correct graph of friction force vs. time will show a linear increase until tmax and then a plateau at the maximum static friction force.
Topper's Solved these Questions
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - CONSOLIDATED EXERCISE.
1 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - CONSOLIDATED EXERCISE. (MULTIPAL-CHOICE QUESTION WITH ONE OR MORE THAN ONE CORRECT ANSWER)
3 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES (Read the given statements and select the correct option.)
1 Videos
- ELASTICITYBOOK - CENGAGE PHYSICSCHAPTER - ELASTICITYEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
- SOUNDBOOK - CENGAGE PHYSICSCHAPTER - SOUNDEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISE
10 Videos
Similar Questions
A block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA block of mass m lying on a rough horizontal surface of friction coefficient μ is pulled by a force F as shown , the limiting friction between the block and surface will be
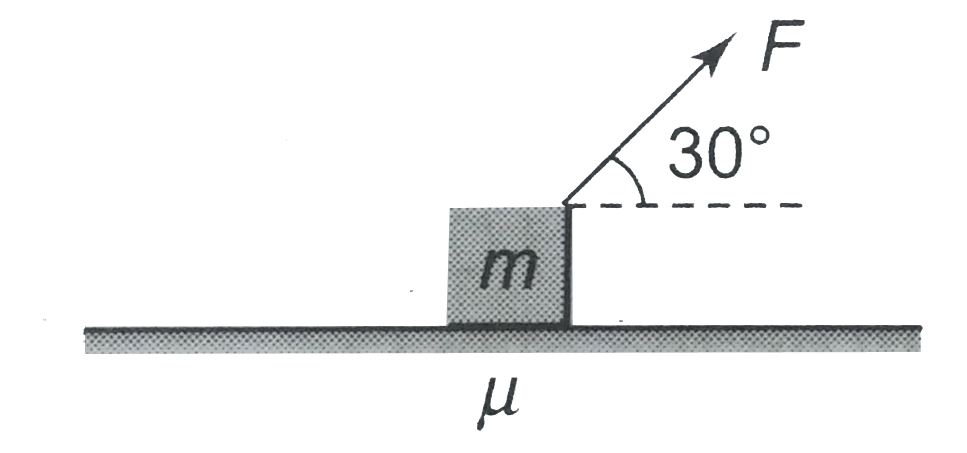
View Solution
Knowledge Check
A block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
A12B√3C1√3DNone
A force F=2t is spplied at t=0 sec to the block of mass m placed on a rough horizontal surface. The coefficient of static and kinetic friction between the block and surface are μS and μk rspectively. Which of the following graphs best represents the acceleration vs time of the block(μS>μk)?A B
B C
C D
D
A block of mass m is pulled by a force of constant power P placed on a rough horizontal plane. The friction coefficient between the block and the surface is μ. Then
AThe maximum velocity of the block during the motion is PμmgBThe maximum velocity of the block during the motion is P2μmgCThe block's speed is never decreasing and finally becomes constantDThe speed of the block first increases to a maximum value and then decreases
A force F accelerates a block of mass m on horizontal surface. The coefficient of friction between the contact surface is . The acceleration of m will be -
View SolutionA horizontal force F acts on the block of mass m and the block remains stationary, thr value of friction force is.
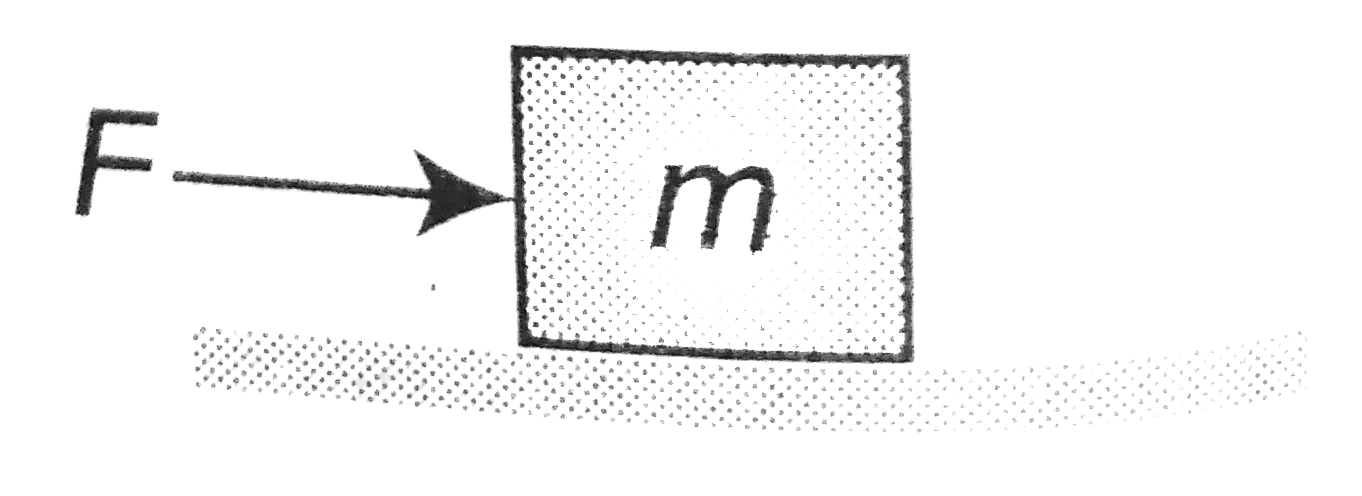
View SolutionA block of mass m is pulled by a constant powert P placed on a rough horizontal plane. The friction coefficient the block and surface is μ. The maximum velocity of the block is.
View SolutionA block is sliding on a rough horizontal surface. If the contact force on the block is √2 times the frictional force, the coefficient of friction is
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View Solution
