The ratio of μk (kinetic friction) and μs (state friction) is
greater than 1
less than 1
equal to 1
depends on condition
The correct Answer is:b
less than 1
Topper's Solved these Questions
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - CONSOLIDATED EXERCISE.
1 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - CONSOLIDATED EXERCISE. (MULTIPAL-CHOICE QUESTION WITH ONE OR MORE THAN ONE CORRECT ANSWER)
3 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES (Read the given statements and select the correct option.)
1 Videos
- ELASTICITYBOOK - CENGAGE PHYSICSCHAPTER - ELASTICITYEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISES
10 Videos
- SOUNDBOOK - CENGAGE PHYSICSCHAPTER - SOUNDEXERCISE - OLYMPIAD AND NTSE LEVEL EXERCISE
10 Videos
Similar Questions
Knowledge Check
A block of mass M is sliding down the plane. Coefficient of static friction is μs and kinetic friction is μk. Then friction force acting on the block is :
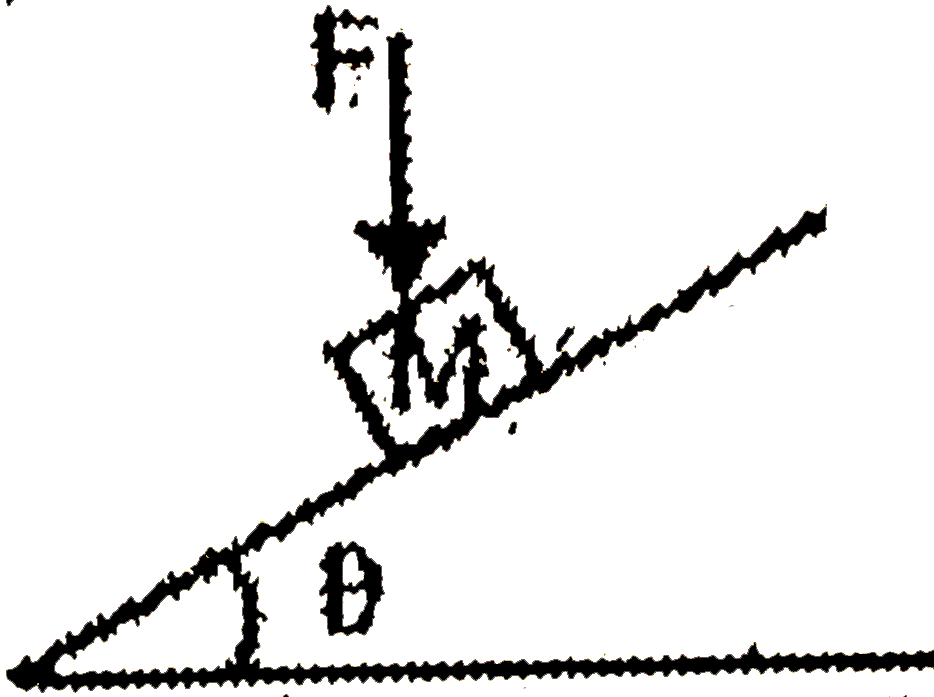 A(F+Mg)sinθBμk(F+Mg)cosθCμsMgcosθD(Mg+F)sinθ
A(F+Mg)sinθBμk(F+Mg)cosθCμsMgcosθD(Mg+F)sinθWhich is true for rolling friction (μr), static friction (μs) and kinetic friction (μk) ?
Aμs>μk>μrBμs<μk<μrCμs<μk>μrDμs>μk>μrIf coefficient of static friction is μs and coefficient of kinetic friction is μk , which one is correct ?
Aμs=μkBμs>μkCμs<μkDμs≤μk
A block of mass m rests on a rough horizontal plane having coefficient of kinetic friction μk and coefficient of static friction μs. The spring is in its natural, when a constant force of magnitude P=5μkmg4 starts acting on the block. The spring force F is a function of extension x as F=kx2.( Where k is spring constant )
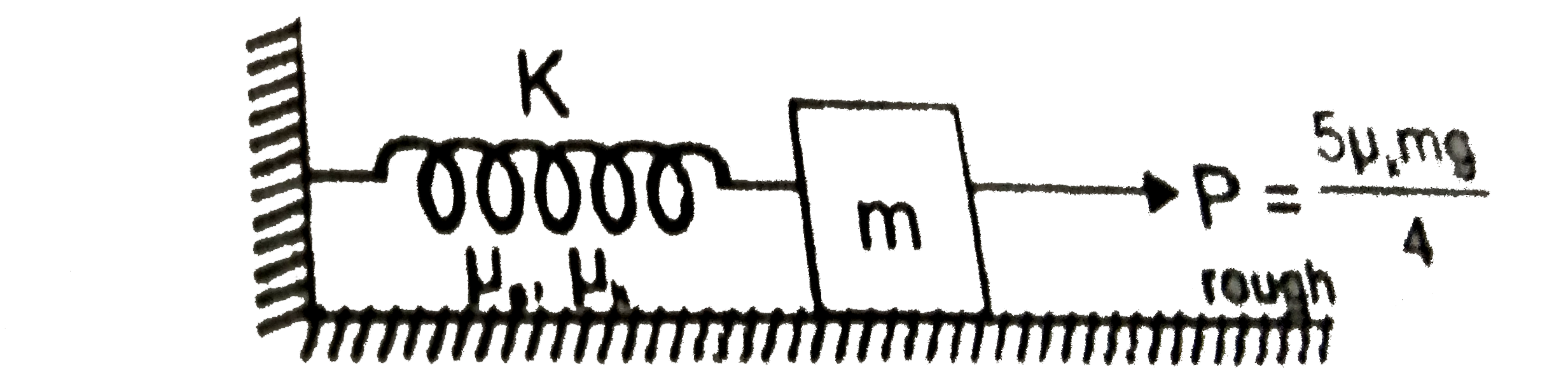
(a) Comment on the relation between μs and μk for the motion to start.
(b) Find the maximum extension in the spring ( Assume the force P is sufficient make the block move ).
View SolutionA block of mass m rests on a rough horizontal plane having coefficient of kinetic friction μk and coefficient of static friction μs. The spring is in its natural, when a constant force of magnitude P=5μkmg4 starts acting on the block. The spring force F is a function of extension x as F=kx2.( Where k is spring constant )

(a) Comment on the relation between μs and μk for the motion to start.
(b) Find the maximum extension in the spring ( Assume the force P is sufficient make the block move ).
View SolutionCoefficient of kinetic friction and coefficient of static friction between two blocks is μk and μs respectively. In general
View SolutionCoefficient of kinetic friction and coefficient of static friction between two blocks is μk and μs respectively. In general
View SolutionIn the Fig. shown a time dependent force F expressed as F=kt is applied on a block of mass 5 kg. Coefficient of static and kinetic friction is μs=0.4 and μk=0.3. Motion begins when t=2 sec draw a acceleration vs time graph for block. (m=5kg,μs=0.4,μk=0.3)

View Solution
