The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC=32∘ and ∠AOB=70∘, then ∠DBC is equal to Solution in Punjabi
24∘
86∘
38∘
32∘
Step by step video & image solution for The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If /_DAC = 32^@ and /_AOB=70^@, then /_DBC is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Three angles of a quadrilteral are respectively 50∘ and 110∘ and 100∘ . Find the fourth angles.
View SolutionDefine a trial.
View SolutionDefine
Statistics as a subject.
View SolutionIn a parallelogram ABCD diagonals AC and BD intersect at O and AC=6.8cm and BD=13.6cm. Find the measures of OC and OD.
View Solutiondiagonal of a cocyclic parallelogram ABCD is AC and BD intersection at point R .then
View SolutionThe diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC=32∘and∠AOB=70∘, then ∠DBC is equal to
View SolutionDiagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA= 3 cm and OD = 2 cm, determine the lengths of AC and BD.
View SolutionThe diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC=32∘and∠AOB=70∘, find ∠DBC.
View SolutionABCD एक समांतर चतुर्भुज है तथा उसके विकर्ण AC और BD एक दूसरे को O बिंदु पर काटते हैं। जिससे ∠DAC=32∘ और ∠AOB=70∘ है तो ∠DBC का मान कया होगा?
View SolutionThe diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If angleDAC=30^(@) and angleAOB=80^(@) , then angleDBC is equal to
View SolutionThe diagonals of the parallelogram ABCD intersect each other at point O. If OC = 6 cm and OB = 8 cm, then give the values of AC and BD.
View SolutionABCD is a parallelogram. The diagonals AC and BD intersect each other at ‘O’. Prove that ar(ΔAOD)=ar(ΔBOC) . (Hint: Congruent figures have equal area)
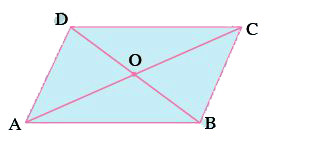
View SolutionABCD is a parallelogram. The diagonals AC and BD intersect each other at ‘O’. Prove that ar(ΔAOD)=ar(ΔBOC) . (Hint: Congruent figures have equal area)
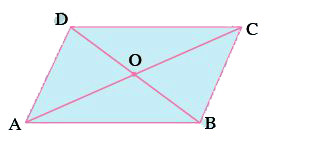
View SolutionABCD is a parallelogram. The diagonals AC and BD intersect each other at ‘O’. Prove that ar(ΔAOD)=ar(ΔBOC) . (Hint: Congruent figures have equal area)
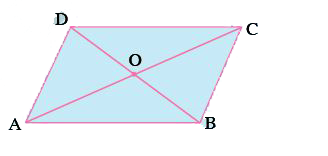
View SolutionDiagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA= 3 cm and OD = 2 cm, determine the lengths of AC and BD.
View SolutionIn cyclic quadrilateral ABCD, Diagonals AC and BD intersect at P. If ∠DBC=70∘ and ∠BAC=30∘ then find ∠BCD
View Solution
