D,E and F are mid-points of the sides BC, CA and AB respectively of △ABC. Prove that
ar(∣∣gmBDEF)=12ar(△ABC)Solution in Punjabi
Step by step video & image solution for D,E and F are mid-points of the sides BC, CA and AB respectively of triangleABC. Prove that ar(||gmBDEF)= 1/2 ar (triangleABC) by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the figure. Show that ABCD is a parallelogram.
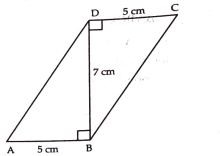 Calculate the area of ||gm ABCD.
Calculate the area of ||gm ABCD.
View SolutionThe radius of a circle is 8 cm and the length of its chords is 12 cm. Fid the distance of the from the centre.
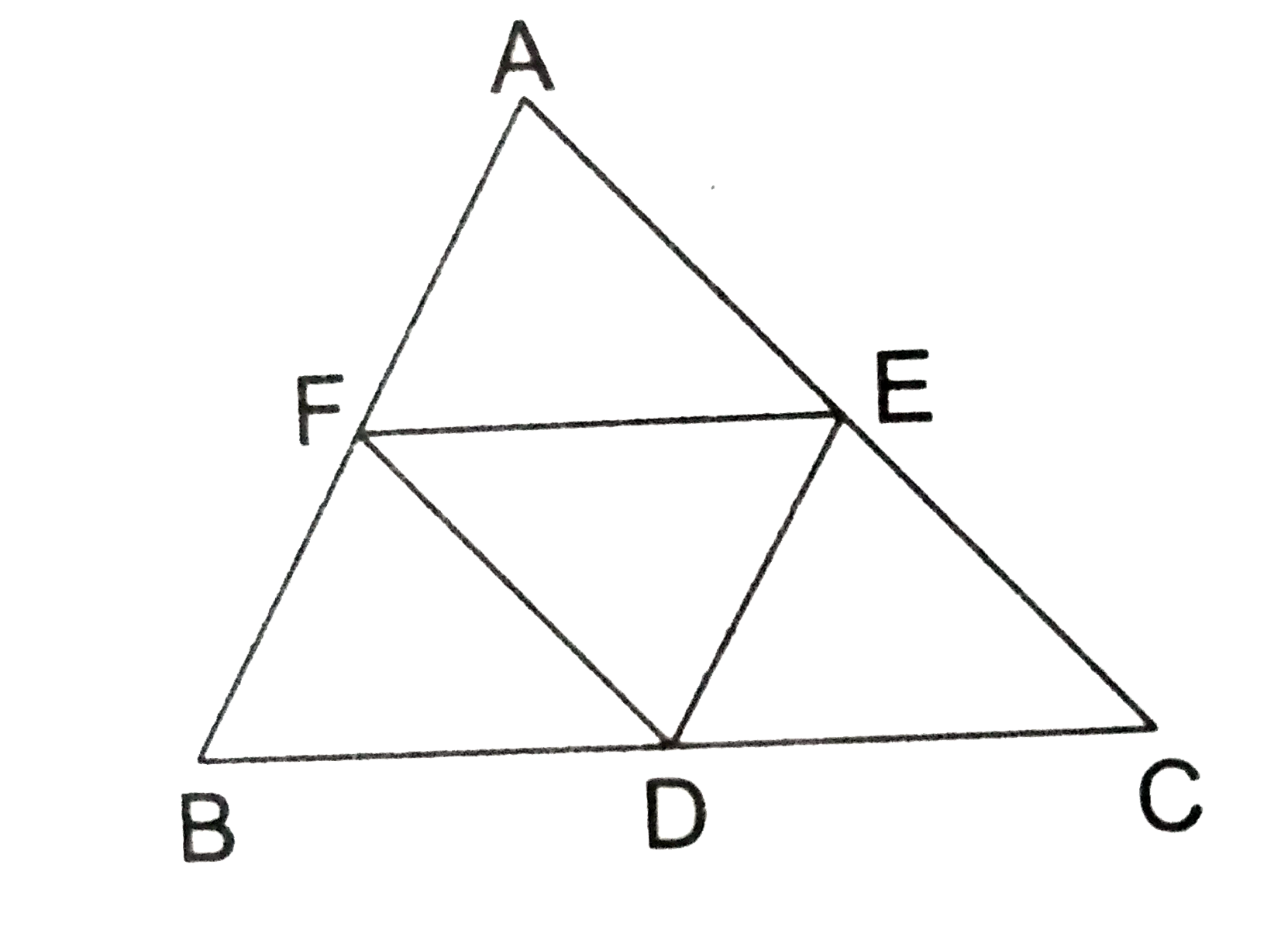
View SolutionD, E, F are the midpoints of the sides BC, CA and AB respectively of △ABC. Prove that
(i) BDEF is a ||gm,
(ii) ar(△DEF)=14ar(△ABC)and
(iii) ar(||gm BDEF)=12ar(△ABC)
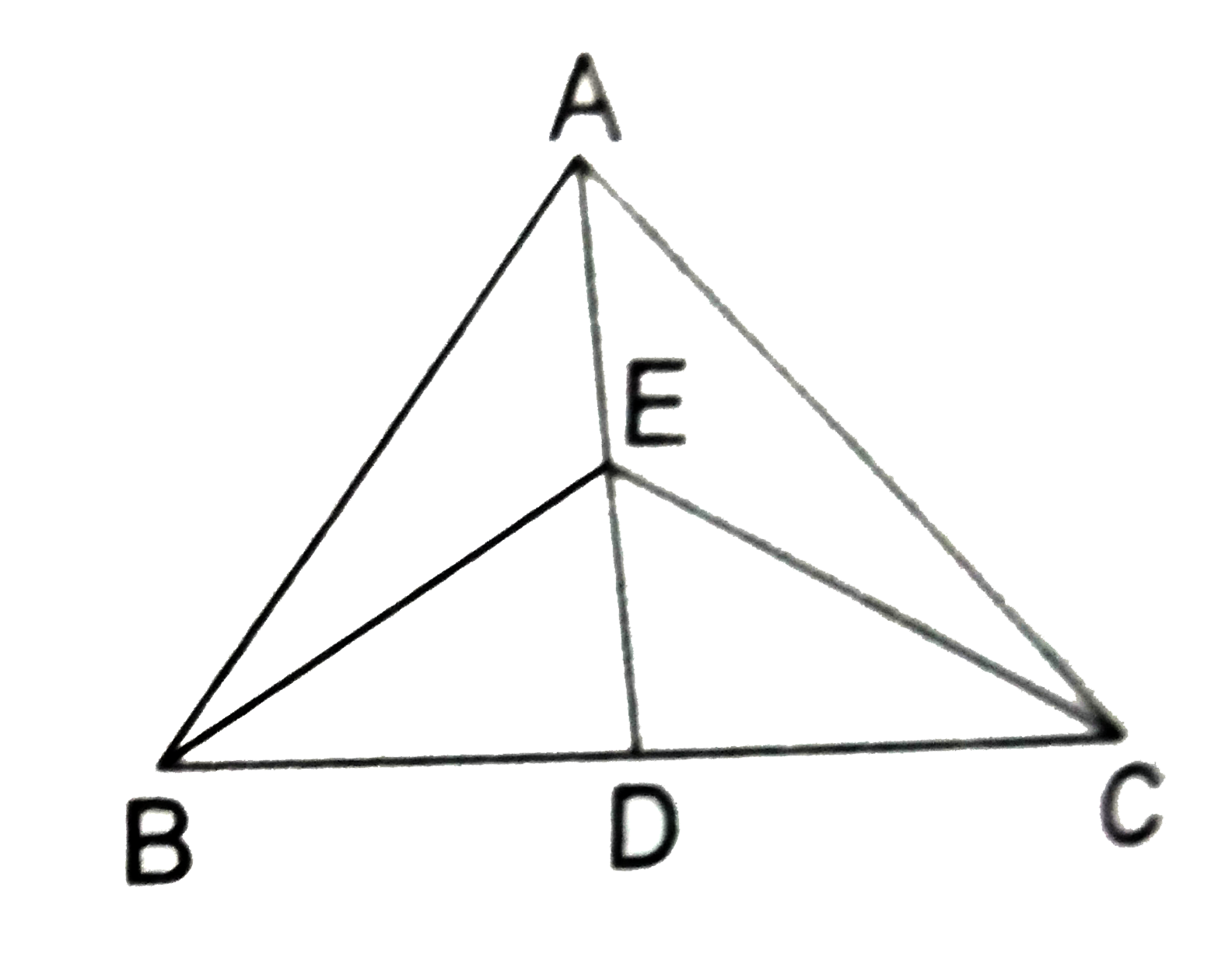
View SolutionThe vertex A of △ABC is joined to a point D on the side BC. The midpoint of AD is E.
Prove that ar(△BEC)=12ar(△ABC).
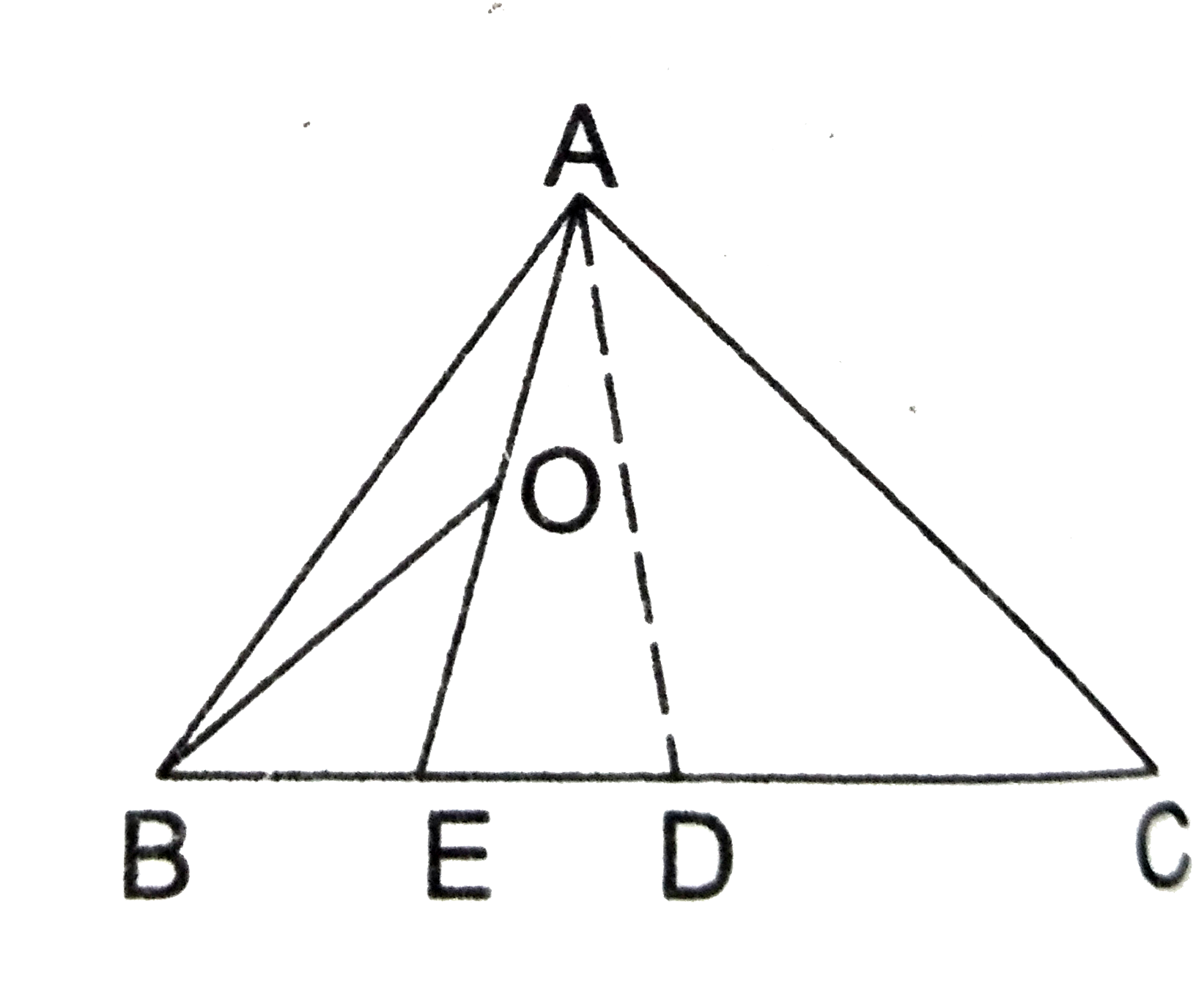
View SolutionD is the midpoint of side BC of △ABC and E is the midpoint of BD. If O is the midpoint of AE,
prove that ar(△BOE)=18ar(△ABC).
View SolutionIf D, E, F are the mid-points of the sides BC, CA and AB respectively of △ABC, then ¯¯¯¯¯¯AD+23¯¯¯¯¯¯BE+13¯¯¯¯¯¯CF=
View SolutionIf D(2,1,0),E(2,0,0)andF(0,1,0) are mid - points of the sides BC,CA and AB of △ABC , respectively , Then , the centroid of ΔABC is
View SolutionAn isosceles triangleABC In AB = AC. If D, E and F are the midpoints of the sides BC, CA and AB respectively, prove that DE = DF
View SolutionD, E and F are respectively the mid-points of sides AB. BC and CA of △ABC. Find the ratio of the areas of △DEF and △ABC.
View SolutionIn △ABC, point D lies on side BC. E is the midpoint of AD. Prove that,
ar(EBC)=12ar(ABC)
View SolutionD.E और F क्रमशः ΔABC की भुजाओं BC,CAऔरAB के मध्य बिंदु है, तो ar(DEF) = ?
View SolutionD,E and F are respectively the mid-points of sides AB,BC and CA of △ABC.Find the ratio of the areas of △DEFand△ABC.
View SolutionD, E and F are respectively the mid-points of the sides BC, CA and AB of a DeltaABC . Show that ar(triangleDEF)=1/4ar(triangleABC)
View SolutionD, E and F are respectively the mid-points of the sides BC, CA and AB of a DeltaABC . Show that ar(BDEF) = 1/2ar(triangleABC)
View SolutionD,E and F are mid-points of the sides BC, CA and AB respectively of triangleABC . Prove that ar(triangleDEF) = 1/4 ar (triangleABC)
View Solution