ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24cm2, then ar (ABC) = 24cm2.Solution in Punjabi
Step by step video & image solution for ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm^2, then ar (ABC) = 24 cm^2. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
Similar Questions
In the figure. Show that ABCD is a parallelogram.
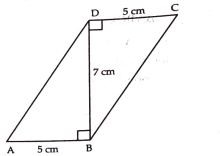 Calculate the area of ||gm ABCD.04:16View Solution
Calculate the area of ||gm ABCD.04:16View SolutionThe radius of a circle is 8 cm and the length of its chords is 12 cm. Fid the distance of the from the centre.
03:14View SolutionABCD is a parallelogram and X is the mid-point of AB. (AXCD)=24cm2, then ar(ABC)=24cm2.
01:34View SolutionThe area of the parallelogram ABCD is 90CM2. Find
(i) ar (ABEF) (ii) ar (ΔABD)
(iii) ar (ΔBEF)
 01:54View Solution
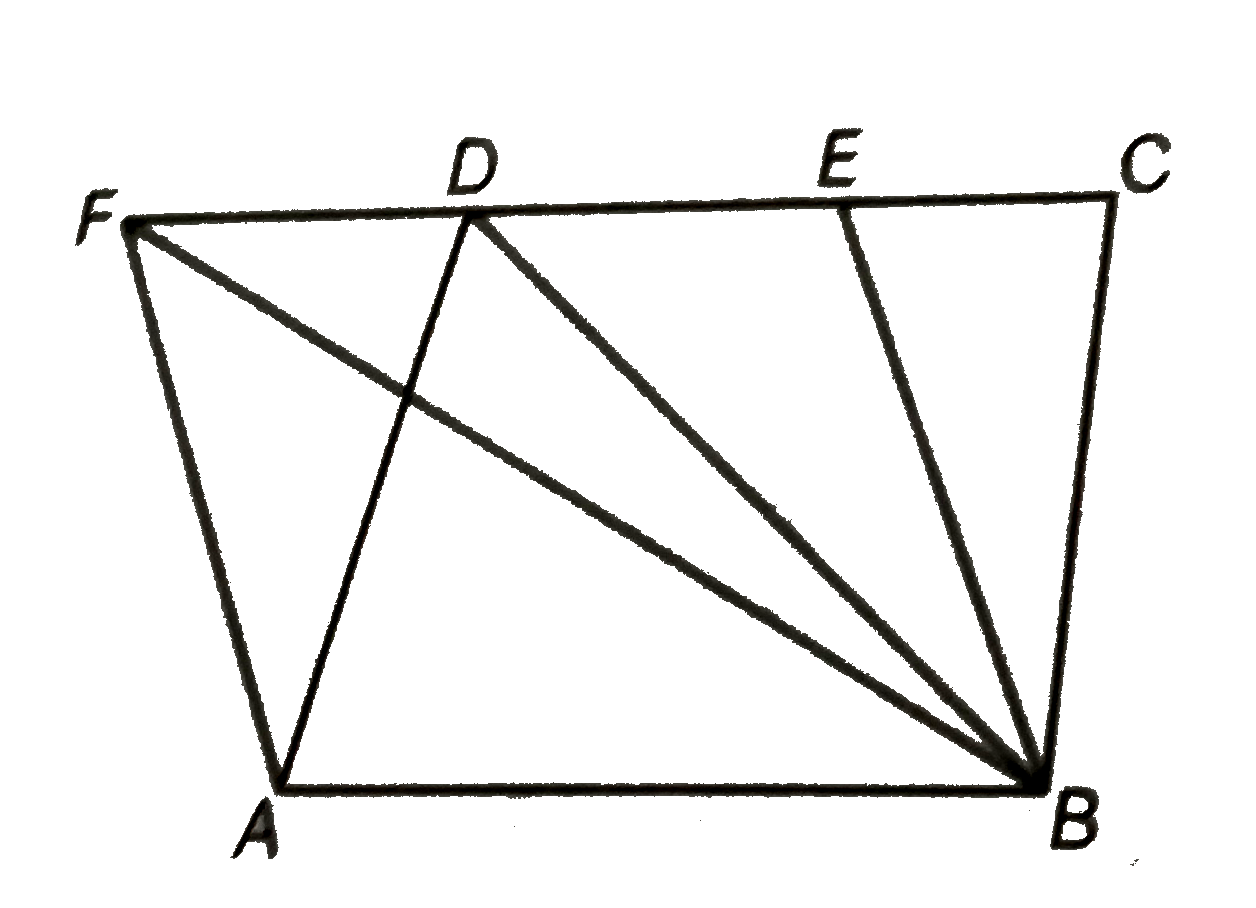
01:54View SolutionIn the given figure, ABCD and ABEF are parallelograms such that ar(quad. EABC) 17cm2 and ar(||gm ABCD) =25cm2. Then ar(△BCF) = ?
 04:08View Solution
04:08View SolutionABCD एक समान्तर चतुर्भुज और X भुजा AB का मध्य-बिंदु है । यदि ar(AXCD)=24cm2 है, तो ar(ABC)=24cm2 है।
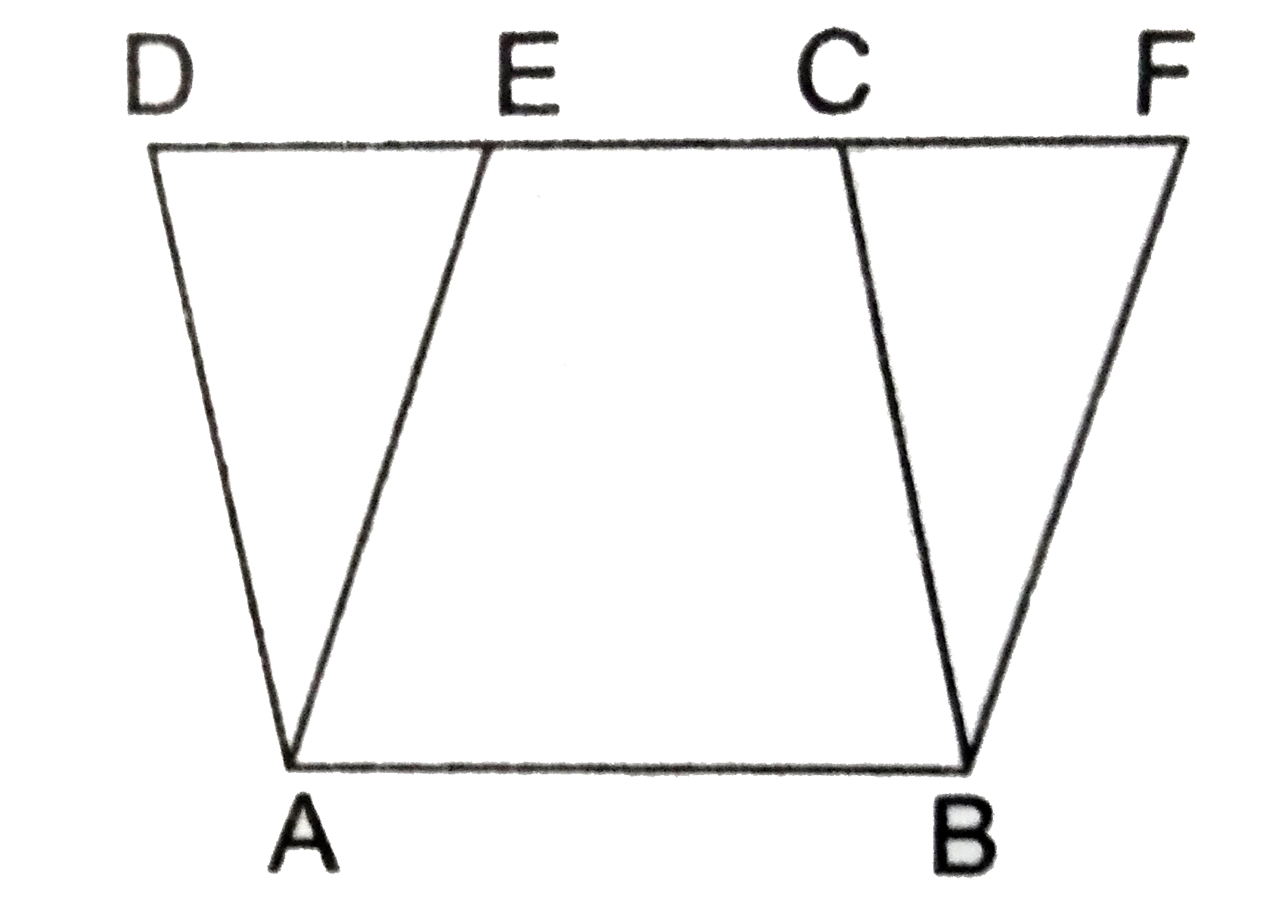
04:12View SolutionIn the figure ABCD and ABFE are Parallelograms then find ar (ΔBCF).
If ar (ABCE)=18cm2
ar (ABCD)=25cm2
 04:32View Solution
04:32View SolutionABCD is a parallelogram and X is the mid-point of AB. (AXCD)=24cm2, then ar(ABC)=24cm2.
01:32View SolutionThe area of the parallelogram ABCD is 90CM2. Find
(i) ar (ABEF) (ii) ar (ΔABD)
(iii) ar (ΔBEF)
 03:38View Solution
03:38View SolutionABCD is a square . If ar(ABCD)=36 cm2 . Then AB=____ cm.
05:05View SolutionFor parallelogram ABCD, ar(ABCD)=48 cm2 . Then ar(ABC)=___ cm2
01:58View SolutionIn figure, ABCD and EFGD are two parallelograms and G is the mid-point of side CD. Then, ar (DPC) = (1)/(2) ar(EFGD).
01:45View SolutionIn the given fig.
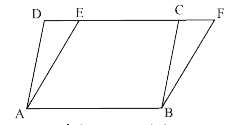
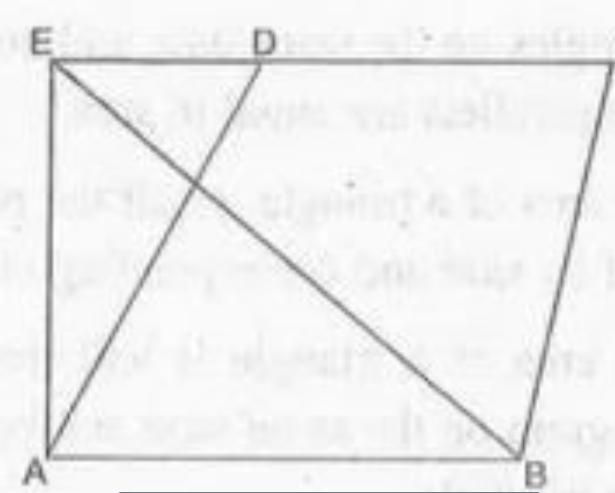 ABCD is a parallelogram and ABE is a triangle. Also AB∣∣CE. If ar (ABCD) = 60cm2 then ar (ΔABE) is :03:23View Solution
ABCD is a parallelogram and ABE is a triangle. Also AB∣∣CE. If ar (ABCD) = 60cm2 then ar (ΔABE) is :03:23View SolutionABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24cm2, then ar (ABC) = 24cm2.
03:39View SolutionABCD is a parallelogram. If ar (ABC) = 18 cm2, then are (ABCD) = …………….. cm2
03:11View SolutionIn the given figure, ABCD and ABFE are parallelograms such that ar(quad. EABC) =19 cm2 and ar(||gm ABCD) = 28 cm2. Then, ar(△BCF) is
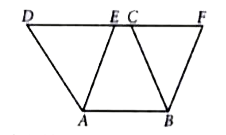 04:10View Solution
04:10View Solution

