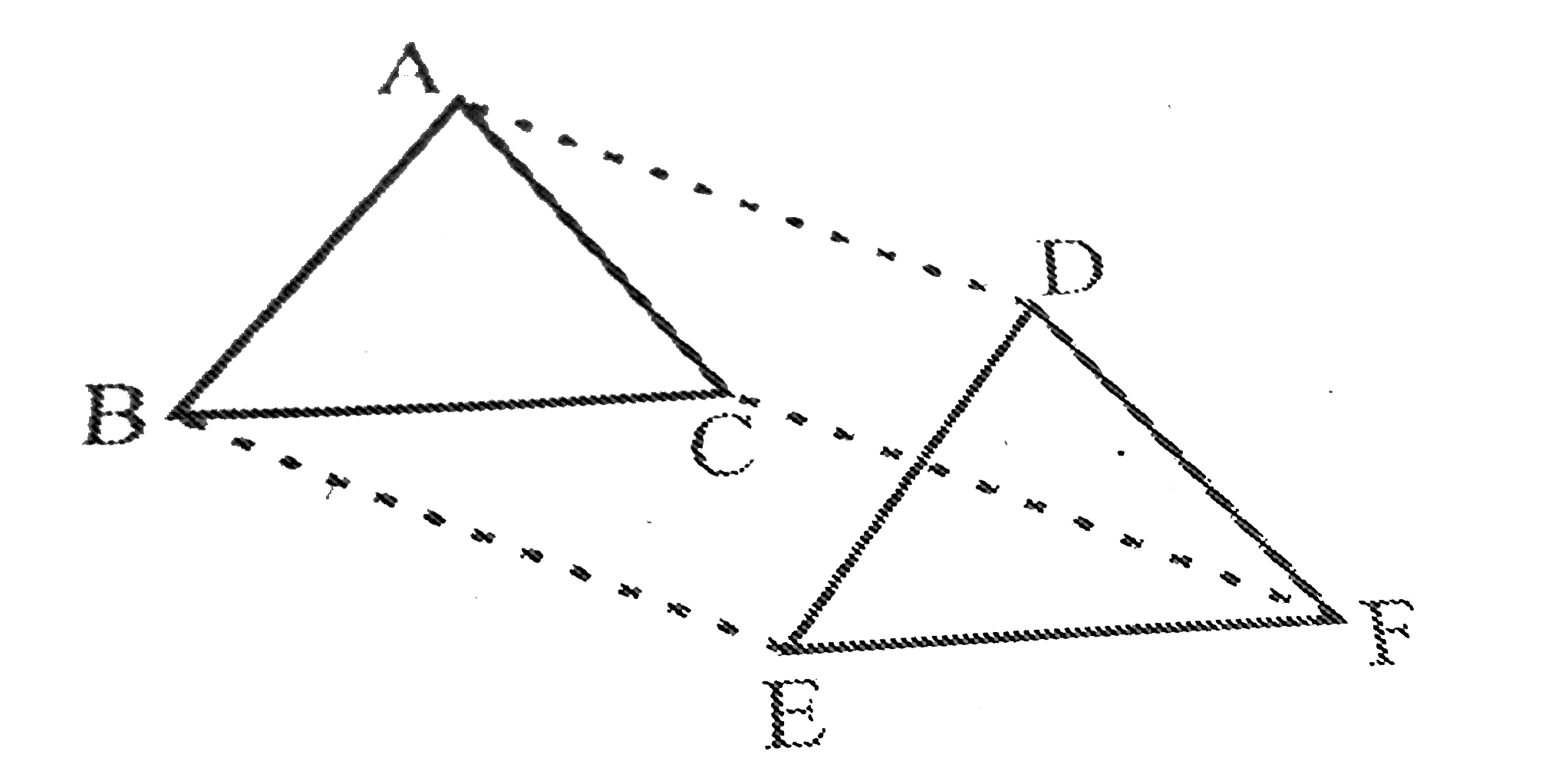
A,B,C and D,E,f are two of collinear points. Prove that AD||CFSolution in Punjabi
Step by step video & image solution for A,B,C and D,E,f are two of collinear points. Prove that AD||CF by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLESBOOK - MODERN PUBLICATIONCHAPTER - CIRCLESEXERCISE - EXERCISE
94 Videos
- AREA OF PARALLELOGRAMS AND TRIANGLESBOOK - MODERN PUBLICATIONCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - EXERCISE
83 Videos
- CONSTRUCTIONSBOOK - MODERN PUBLICATIONCHAPTER - CONSTRUCTIONSEXERCISE - EXERCISE
69 Videos
Similar Questions
The radius of a circle is 8 cm and the length of its chords is 12 cm. Fid the distance of the from the centre.
View SolutionIn the figure. Show that ABCD is a parallelogram.
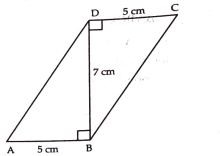 Calculate the area of ||gm ABCD.
Calculate the area of ||gm ABCD.
View SolutionDraw a line segment of length 7.3 cm using a ruler.
View SolutionIn ΔABCand ΔDEF, AB = DE, AB ∣∣ DE, BC = EF and BC ∣∣ EF. Vertices A, B and C are joined to vertices D, E and F respectively (see Fig. 8.22).Show that
(i) quadrilateral ABED is a parallelogram
(ii) quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) ∆ ABC ≅ ∆ DEF.
View SolutionABCD is a parallelogram, G is the point on AB such that AG=2GB,E is a point of DC such that CE=2DE and F is the point of BC such that BF=2FC. Prove that: ar(EFC)=12ar(EBF)
View SolutionAD, BE and CF are the medians of triangle ABC whose centroid is G. If the points A, F, G and E are concyclic, then prove that 2a2=b2+c2
View SolutionABCD is a parallelogram. A circle passing through C and D intersects AD and BC at the points E and F. Prove that the four points E, A, B, F are concyclic.
View SolutionProve that the points (a, b), (c, d) and (a-c, b-d) are collinear, if ad = bc.
View SolutionWrite 'T' for true and 'F' for false in case of each of the following statements
If points A,B,C are collinear and points C,D,E are collinear then the points A,B,C,D,E are collinear.
View SolutionIn ΔABCand ΔDEF, AB = DE, AB ∣∣ DE, BC = EF and BC ∣∣ EF. Vertices A, B and C are joined to vertices D, E and F respectively (see Fig. 8.22).Show that
(i) quadrilateral ABED is a parallelogram
(ii) quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) quadrilateral ACFD is a parallelogram
(v) AC = DF
(vi) ∆ ABC ≅ ∆ DEF.

View SolutionAD, BE and CF are the medians of triangle ABC whose centroid is G. If the points A, F, G and E are concyclic, then prove that 2a2=b2+c2
View SolutionThe diagonal BD of a parallelogram ABCD intersects the segment AE at the point F, where E is any point on the side BC . Prove that DF⋅EF=FB⋅FA.
View SolutionIn Figure, DE∣∣AC and DF∣∣AE . Prove that EFBF=ECBE .
View SolutionTwo circle intersect in A and B and AC and AD are respectively the diameters of the circles. Prove that C,B,D are collinear.
View SolutionIn Figure, A, B, C and D, E, F are two sets of collinear points. Prove that AD∣∣CF
View SolutionProve that the points (a, b), (c, d) and (a-c, b-d) are collinear, if ad = bc.
View Solution
