The motion of a particle of mass m is given by x=0 for t<0s,x(t)=Asin4πt for 0<t<(14)s
(A>0) and x=0 for t>(14)s.
Step by step video & image solution for The motion of a particle of mass m is given by x=0 for tlt0s,x(t)=Asin4pit for 0lttlt((1)/(4))s (Agt0) and x=0 for tgt((1)/(4))s. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Fig. shows the position-time graph of a particle of mass 4 kg. What is the (i) force acting on the particle for t<0,t<4s,0<t<4s? (ii) impulse at t = 0 and t = 4 s? Assume that the motion is one dimensional.
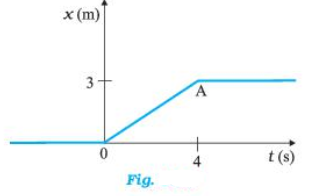 View Solution
View SolutionThe figure shows the position time graph of a particle of mass 40 kg. What is the force acting on the particle of 0<t<4 s
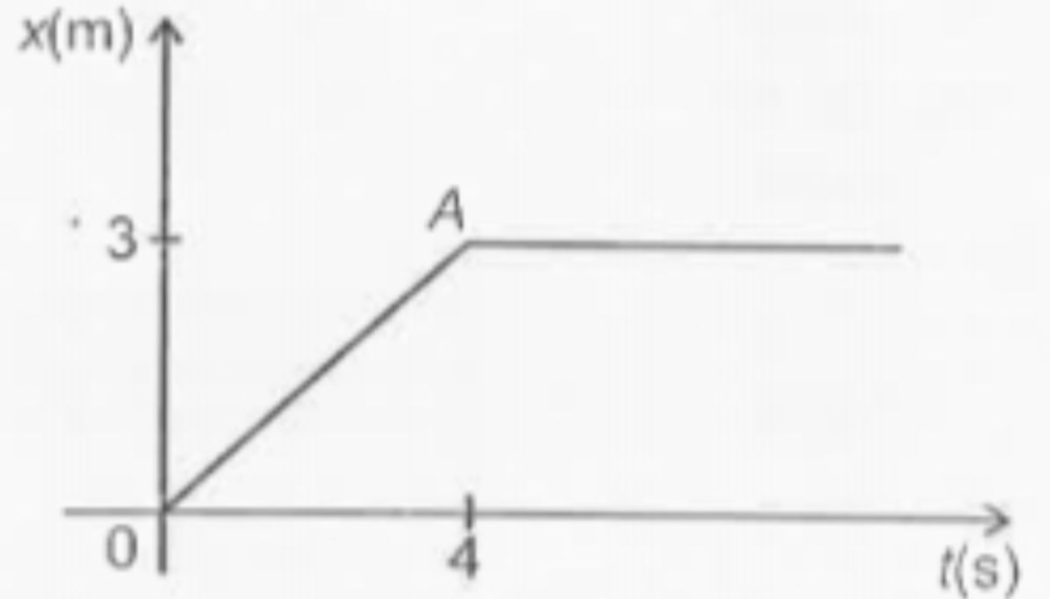 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Figure shows the position-time graph of a particle of mass 4kg. Let the force on the particle for t<0 , 0<tlt,4s , t>4s be F1,F2 and F3 respectively. Then
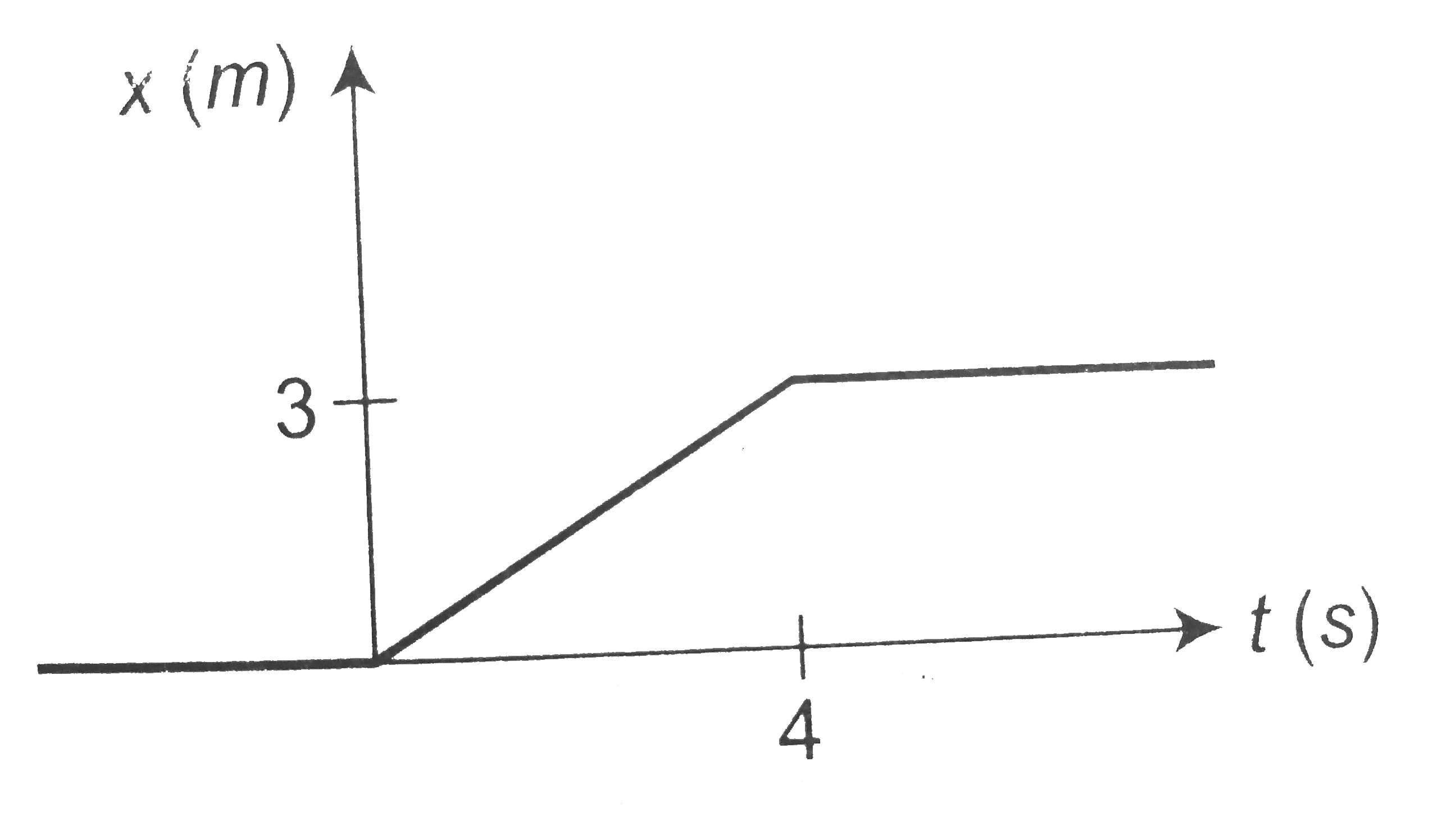 AF1=F2=F3=0BF1>F2=F3CF1>F2>F3DF1<F2<F3
AF1=F2=F3=0BF1>F2=F3CF1>F2>F3DF1<F2<F3
The equation of a wave on a string of linear mass density 0.04kgm−1 is given by
y=0.02(m)sin[2π(t0.04(s)−x0.50(m))].
Then tension in the string isView SolutionIf velocity of a particle is given by v=(2t+3)m/s. Then average velocity in interval 0≤t≤1 s is :
View SolutionThe acceleration of a particle moving in straight line is defined by the relation a=−4x(−1+14x2), where a is acceleration in m/s2 and x is position in meter. If velocity v=17m/s when x=0, then the velocity of the particle when x=4 meter is :
View SolutionThe body moves along the x−axis . At time t=0 the velocity of particle is +0.5m/s. The acceleration of particle in different time interval is given as:
a=⎧⎪ ⎪⎨⎪ ⎪⎩−1m/s2 0s≤t≤3s−2m/s2 3s<t<6s+4m/s2 6s≤t≤10sView SolutionVelocity time equation of a particle moving in a straight line is v=2t−4 for t≤2s and v=4−2t for t>2.The distance travelled by the particle in the time interval from t=0 to t=4s is (Here t is in second and v in m/s)
View SolutionThe position of a particle moving on X-axis is given by
x=At3+Bt2+Ct+D.
The numerical values of A,B,C,D are 1,4,-2 and 5 respectively and I units are used. Find a. the dimensions of A,B, C and D b. the velocity of the particle at t=4s, the acceleration of he particle at t=4s, d. The average velocity during the interval t=0 to t=4s, the average accelerationduring the interval t=0 to t=4s.View SolutionAcceleration of a particle is given by
a=1/V
Where V is the velocity . Find
(i) Velocity of particle at time t, given that v = 10 m/s at t = 0.
(ii) Velocity of particle when its position is x, given that speed is 3 m/s at x = 0.View Solution
