A current I flows along a triangular loop having sides of equal length a. What should be the strength of magnetic field at the centre of the loop?
A
B
C
D
Text Solution
The correct Answer is:B
By using the Biot Savart.s law,
a = length of side of equilateral triangle
r = perpendicular distance of each side from centroid
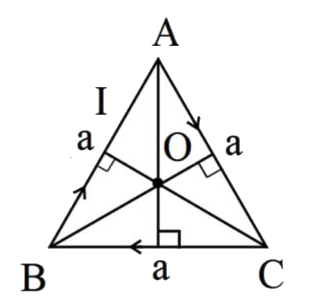
Take case of AB segment, angle made by A and B at centroid i.e.
Since all the segments are identical, magnetic field due to each of them will be equal in magnitude and direction.
Using Right hand rule, we can see that magnetic field due to any single wire will be into the plane. So magnetic field due to all the three segments can be simply added.
|
Topper's Solved these Questions
- NTA TPC JEE MAIN TEST 42BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 42EXERCISE - PHYSICS (SUBJECTIVE NUMERICAL)
10 Videos
- NTA TPC JEE MAIN TEST 41BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 41EXERCISE - PHYSICS
30 Videos
- NTA TPC JEE MAIN TEST 43 BOOK - NTA MOCK TESTSCHAPTER - NTA TPC JEE MAIN TEST 43 EXERCISE - PHYSICS
30 Videos
Similar Questions
Knowledge Check
A current 1 flows along a triangle loop having sides of equal length a. The strength of magnetic field at the centre of the loop is :
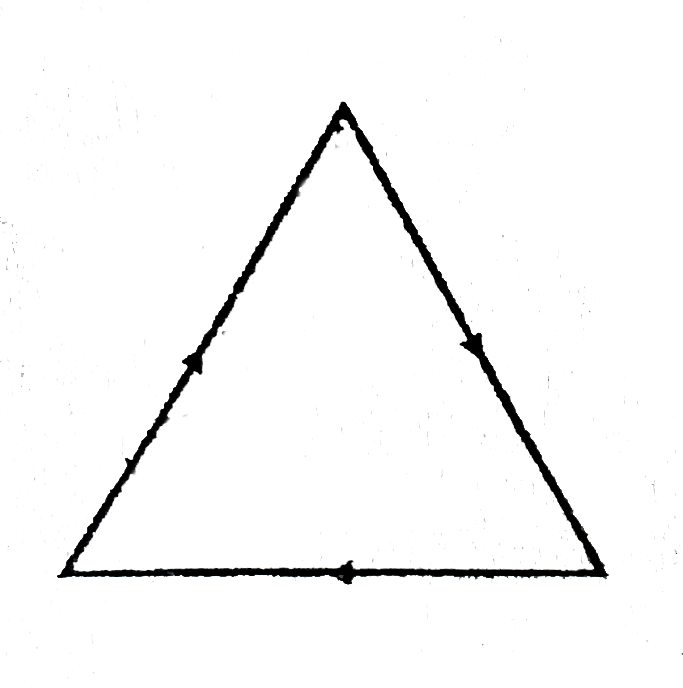 A3μ0I2πaB9μ0I2πaC3√3μ0I2πaD3μ0I4πa
A3μ0I2πaB9μ0I2πaC3√3μ0I2πaD3μ0I4πaA current 1 flows along a triangle loop having sides of equal length a. The strength of magnetic field at the centre of the loop is :
 A3μ012πaB9μ0I2πaC(3√3μ0I)/(2πa)D3μ0I4πa
A3μ012πaB9μ0I2πaC(3√3μ0I)/(2πa)D3μ0I4πaA rectangular loop of metallic wire is of length a and breadth b and carries a current i. The magnetic field at the centre of the loop is
Aμ0i4π8√a2+b2abBμ0i4π4√a2+b2abCμ0i4π2√a2+b2abDμ0i4π√a2+b2ab
A square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is (dependence on L)
View SolutionA square loop of side a carries a current I. The magnetic field at the center of the loop is
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View Solution
