Consider a unit cube which is acted upon by an equal tensile force of F on all the six surfaces of the cube. The increase in the length of each side is fount to be -FY–xσFY where Y, σ are Young's modulus and Poisson's ratio, respectively. Now, Find the value of X=?
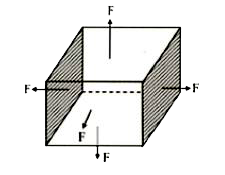
Tensile strain on each face =lL=FAY
For A=1 sq Unit
lL=FY
Leteral strain due to force acting on perpendicular face =−σ×lL
=−σ×FY
As force is subjected from both directions
Lateral Strain =−2σFY
Total increase in length is attributed to both
tensile and lateral strain.
Increase in length of each side
=FY+(−2σFY)
=(1−2σ)FY
⇒x=2
Topper's Solved these Questions
Similar Questions
Find the center of mass(x,y,z) of the following structure of four identical cubes if the length of each side of a cube is 1 unit.

View SolutionA unifrom bar of Length 'L' and cross sectional area 'A' is subjected to a tesnile load 'F','Y' be the Young's modulus and 'σ' be the Poisson's ratio then volumeteric strain is
View Solution
Knowledge Check
On all the six surfaces of a unit cube, equal tensile force of F is applied. The increase in length of each side will (Y = Young's module, σ= Poission's ratio)
AFY(1−σ)BFY(1+σ)CF(1−2σ)YDFY(1−2σ)Find the center of mass(x,y,z) of the following structure of four identical cubes if the length of each side of a cube is 1 unit.
 A(1/2,1/2,1/2B(1/3,1/3,1/3)C(3/4,3/4,3/4)D(1/2,3/4,1/2)
A(1/2,1/2,1/2B(1/3,1/3,1/3)C(3/4,3/4,3/4)D(1/2,3/4,1/2)A wire of length L and radius r is loaded with a weigth Mg. If y and σ denote the Youngs modulus and Poisson's ratio of the material of the wire respectively. Then the decreases in the radius of the wire is given by
AΔr=σπrMgYBr=MgrσπYCΔr=MgσπrYDΔr=MgYπrσ
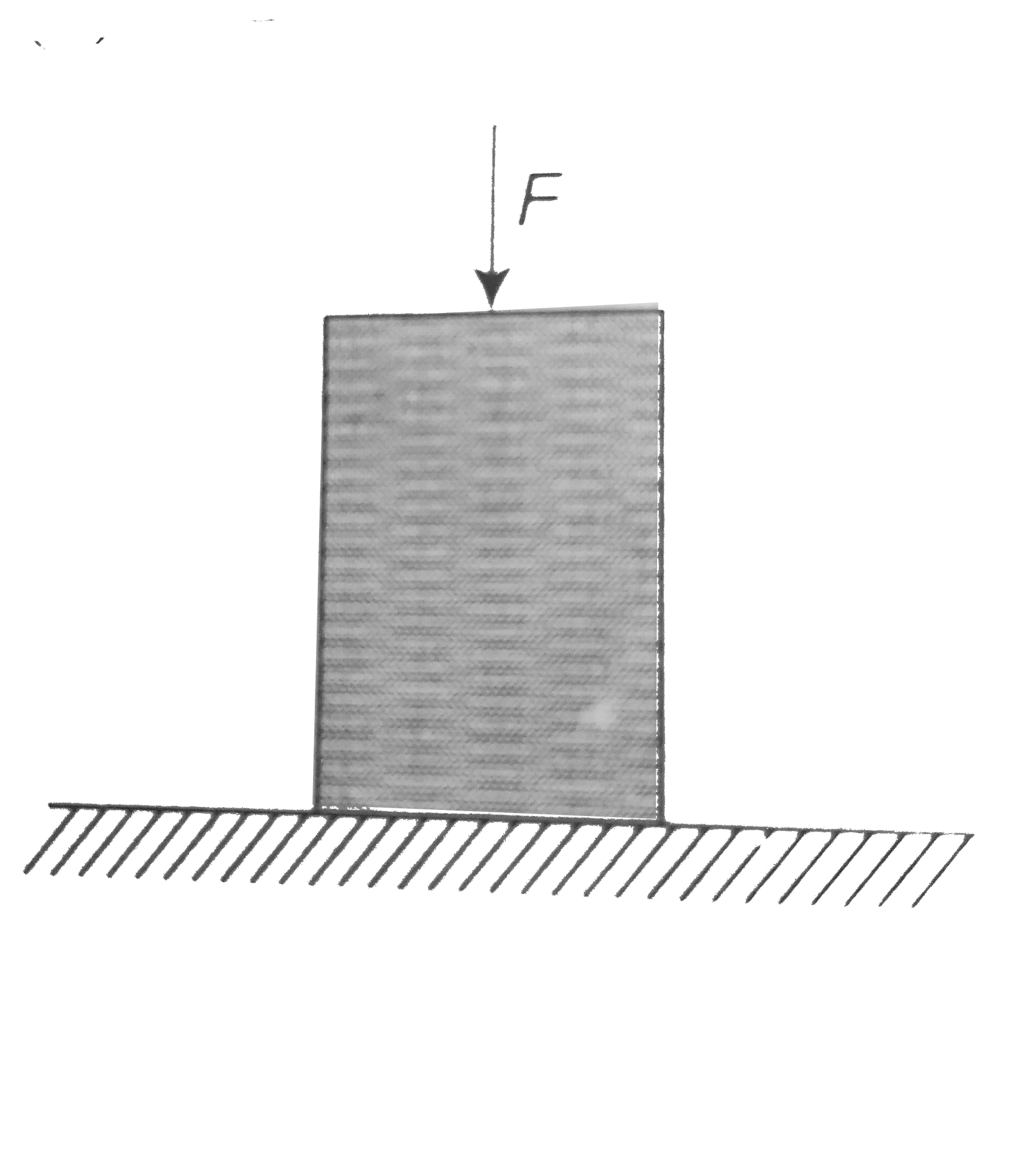
A metal cylinder of length L is subjected to a uniform compressive to a uniform compressive force F as shown in the figure. The material of the cylinder has Young's modulus Y and poisson's ratio σ The change in volume of the cylinder is
View Solution
A metal cylinder of length L is subjected to a uniform compressive to a uniform compressive force F as shown in the figure. The material of the cylinder has Young's modulus Y and poisson's ratio σ The change in volume of the cylinder is
View SolutionYoung's modulus of the material of a wire is Y. ON pulling the wire by a force F, the increase in its length is x. The potential energy of the stretched wire is
View Solutionf(x)=y+1y, where y>0. If y increases in value, then f (x)
View SolutionA uniform rod of length (L) and area of cross-section (A) is subjected to tensil load (F). If σ be the Poisson's ratio and Y be the Young's modulus of the material of the rod, then find the volumetric strain produced in the rod.
View Solution
