What is the maximum acceleration of a platform that oscillates at amplitude 2.50cm and frequency 6.60Hz?
To find the maximum acceleration of a platform that oscillates with a given amplitude and frequency, we can follow these steps:
Step 1: Convert Amplitude to Meters
The amplitude is given as 2.50 cm. We need to convert this to meters for our calculations.
Amplitude (A)=2.50cm=2.50×10−2m
Step 2: Identify the Frequency
The frequency (f) is given as 6.60 Hz.
f=6.60Hz
Step 3: Calculate Angular Frequency
Angular frequency (ω) is related to the frequency (f) by the formula:
ω=2πf
Substituting the value of f:
ω=2π×6.60Hz
Calculating this gives:
ω≈41.47rad/s
Step 4: Use the Formula for Maximum Acceleration
The maximum acceleration (A_max) in simple harmonic motion (SHM) can be calculated using the formula:
Amax=ω2A
Substituting the values of ω and A:
Amax=(41.47)2×(2.50×10−2)
Calculating this gives:
Amax≈43.0m/s2
Conclusion
The maximum acceleration of the platform is approximately:
43.0m/s2
---
The amplitude is given as 2.50 cm. We need to convert this to meters for our calculations.
The frequency (f) is given as 6.60 Hz.
Angular frequency (ω) is related to the frequency (f) by the formula:
The maximum acceleration (A_max) in simple harmonic motion (SHM) can be calculated using the formula:
The maximum acceleration of the platform is approximately:
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Practice Questions57videos
OSCILLATIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - OSCILLATIONSEXERCISE - Checkpoint8videos
OSCILLATIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - MOTION IN TWO AND THREE DIMENSIONSEXERCISE - PRACTICE QUESTIONS (MATRIX-MATCH)10videos
MOTION IN TWO AND THREE DIMENSIONS
- BOOK - RESNICK AND HALLIDAYCHAPTER - PHOTONS AND MATTER WAVESEXERCISE - PRACTICE QUESTIONS(Integer Type)4videos
PHOTONS AND MATTER WAVES
Similar Questions
A certain resonance curve describes a mechanical oscillating system with logarithmic damping decrement λ=1.60. For this curve find the ration of the maximum displacemetn amplitude to the displacement amplitude at a very low frequency.
View SolutionWrite the equation of a stationary wave whose constituting waves have velocity, amplitude and frequency as 150 cm/s, 10 cm and 50 Hz respectively.
View Solution
Knowledge Check
- Question 1 - Select One
If α and β denote the maximum velocity and maximum acceleration of a simple harmonic oscillator, then its amplitude of vibration is
Aα2βBβ2αCa2/βDβ2/α - Question 2 - Select One
A particle is executing simple harmonic motion with an amplitude of 0.02 metre and frequency 50 Hz . The maximum acceleration of the particle is
A100m/s2B100π2m/s2C100m/s2D200π2m/s2
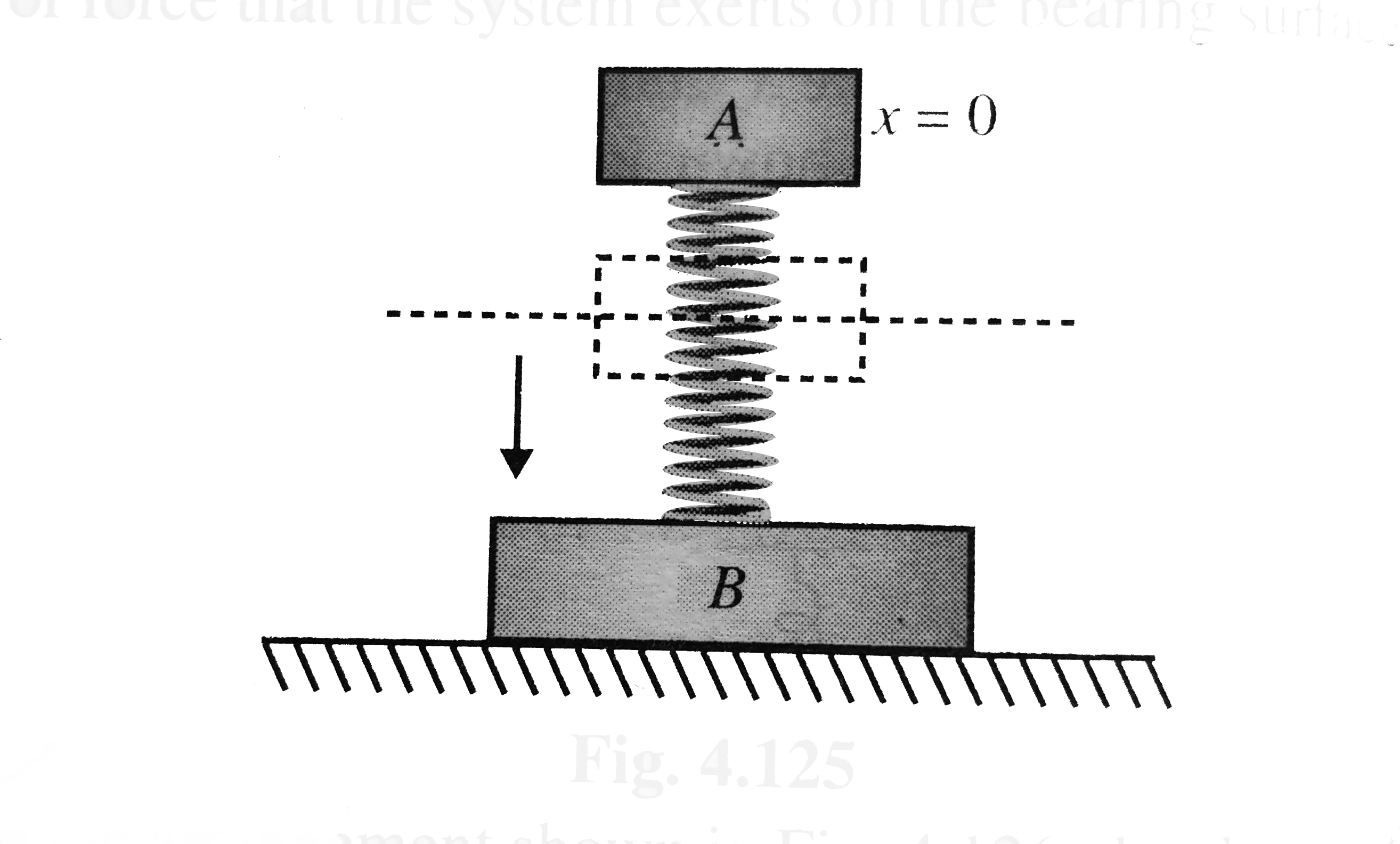
A body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
 View Solution
View SolutionA particle is executing linear S.H.M. with a maximum velocity of 50 cm/s and a maximum acceleration of 40 cm/s2. Calculate the amplitude and the period of oscillation.
View SolutionAn object kept on the platform a horizontal platform executing SHM, up and down with amplitude 80 cm. At what frequency will the object just lose contact with the platform?
View SolutionA block is placed on a platform which is making oscillations with amplitude of 10cm along a vertical line.The least period of oscillation of platform so that it will not get separated from the platform is
View SolutionThe consitutent waves of a stationary wave on a string fixed at two ends are amplitude 6 cm, frequency 50Hz and velocity 80cms−1. Write down the equation of stationary wave.
View SolutionThe maximum acceleration of a simple harmonic oscillator is a0 and maximum velocity is v0. What is the displacement amplitude?
View Solution

