Assertion (A): We use thick wire in the secondary coil of a step down transformer to reduce the production of heat.
Reason (R): If the plane of armature is parallel to the lines of force of magnetic field the magnitude of induced EMF is maximum.
Both (A) and (R) are true and (R) is the correct explanation of (A).
Both (A) and (R) are true but (R) is not the correct explanation of (A).
(A) is true but (R) is false.
(A) is false and (R) is also false.
To analyze the assertion and reason provided in the question, we will break down the concepts involved in both statements.
1. Understanding the Assertion (A):
- The assertion states that we use thick wire in the secondary coil of a step-down transformer to reduce the production of heat.
- In a transformer, the wire's thickness is related to its resistance. A thicker wire has a lower resistance compared to a thinner wire.
- According to Joule's law, the heat produced in a conductor is given by the formula H=I2Rt, where H is the heat produced, I is the current, R is the resistance, and t is the time.
- By using a thick wire with lower resistance, we can minimize the heat produced in the coil due to the current flowing through it.
2. Understanding the Reason (R):
- The reason states that if the plane of the armature is parallel to the lines of force of the magnetic field, the magnitude of induced EMF is maximum.
- The induced EMF in a coil is given by Faraday's law of electromagnetic induction, which states that the induced EMF is proportional to the rate of change of magnetic flux through the coil.
- The magnetic flux Φ is given by Φ=B⋅A⋅cos(θ), where B is the magnetic field strength, A is the area, and θ is the angle between the magnetic field lines and the normal to the surface of the coil.
- When the plane of the armature is parallel to the magnetic field lines, θ=0 degrees, which means cos(0)=1. This configuration maximizes the magnetic flux and thus maximizes the induced EMF.
3. Conclusion:
- The assertion is correct because using a thick wire reduces heat production due to lower resistance.
- The reason is also correct because the maximum induced EMF occurs when the plane of the armature is parallel to the magnetic field lines.
- Therefore, both the assertion and the reason are correct, but they are not directly related to each other.
Final Answer:
Both Assertion (A) and Reason (R) are correct, but the reason does not explain the assertion.
- The assertion states that we use thick wire in the secondary coil of a step-down transformer to reduce the production of heat.
- In a transformer, the wire's thickness is related to its resistance. A thicker wire has a lower resistance compared to a thinner wire.
- According to Joule's law, the heat produced in a conductor is given by the formula H=I2Rt, where H is the heat produced, I is the current, R is the resistance, and t is the time.
- By using a thick wire with lower resistance, we can minimize the heat produced in the coil due to the current flowing through it.
- The reason states that if the plane of the armature is parallel to the lines of force of the magnetic field, the magnitude of induced EMF is maximum.
- The induced EMF in a coil is given by Faraday's law of electromagnetic induction, which states that the induced EMF is proportional to the rate of change of magnetic flux through the coil.
- The magnetic flux Φ is given by Φ=B⋅A⋅cos(θ), where B is the magnetic field strength, A is the area, and θ is the angle between the magnetic field lines and the normal to the surface of the coil.
- When the plane of the armature is parallel to the magnetic field lines, θ=0 degrees, which means cos(0)=1. This configuration maximizes the magnetic flux and thus maximizes the induced EMF.
- The assertion is correct because using a thick wire reduces heat production due to lower resistance.
- The reason is also correct because the maximum induced EMF occurs when the plane of the armature is parallel to the magnetic field lines.
- Therefore, both the assertion and the reason are correct, but they are not directly related to each other.
Both Assertion (A) and Reason (R) are correct, but the reason does not explain the assertion.
Topper's Solved these Questions
- SAMPLE PAPER 9 BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 9 EXERCISE - SECTION - C
3 Videos
- SAMPLE PAPER 9 BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 9 EXERCISE - SECTION - C
3 Videos
- SAMPLE PAPER 7BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 7EXERCISE - SECTION-C
6 Videos
- SAMPLE PAPER SELF- ASSESSMENT -(10)BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER SELF- ASSESSMENT -(10)EXERCISE - SECTION-B
17 Videos
Similar Questions
At which position of the plane of the rotating coil with the direction of magnetic field the e.m.f induced in the coil is maximum ?
View SolutionA coil of 50 turns, each of area 0.12 m2 is rotated at a constant speed of 600 revolutioins per minute in a uniform magnetic field of induction 0.02 T about an axis in the plane of the coil and perpendicular to the direction of the field. The maximum emf induced in a coil is
View Solution
Knowledge Check
Assertion : We use a thick wire in the secondary of a step down transformer to reduce the production heat .
Reason : When the plane of the armature is parallel to the lines of force of magnetic field , the magnitude of induced e.m.f. is maximum .AIf both the assertion and reason are true and reason is correct explanation of the assertion .BIf both the assertion and reason are true but reason is not a correct explanation of the assertion .CIf the assertion is true but the reason is false.DIf both assertion and reason are false.Assertion (A) : A commercial motor uses a soft iron core on which the coil carrying current in wound.
Reason (R) : Presence of soft iron core enhances the power of the motor as it increase the magnetic field .ABoth (A) and (R) are true and (R) is correct explanation of the assertionBBoth (A) and (R) are true but (R) is not the correct explanation of the assertion.C(A) is true but (R) is false.D(A) is false but (R) is true.Assertion A conducting loop is rotated in a uniform magnetic field with constant angular velocity ω as shown in figure. At time t = 0, plane of the loop is perpendicular to the magnetic field. Induced emf produced in the loop is maximum when plane of loop is parallel to magnetic field.
Reason When plane of loop is parallel to magnetic field, then magnetic flux passing through the loop is zero.
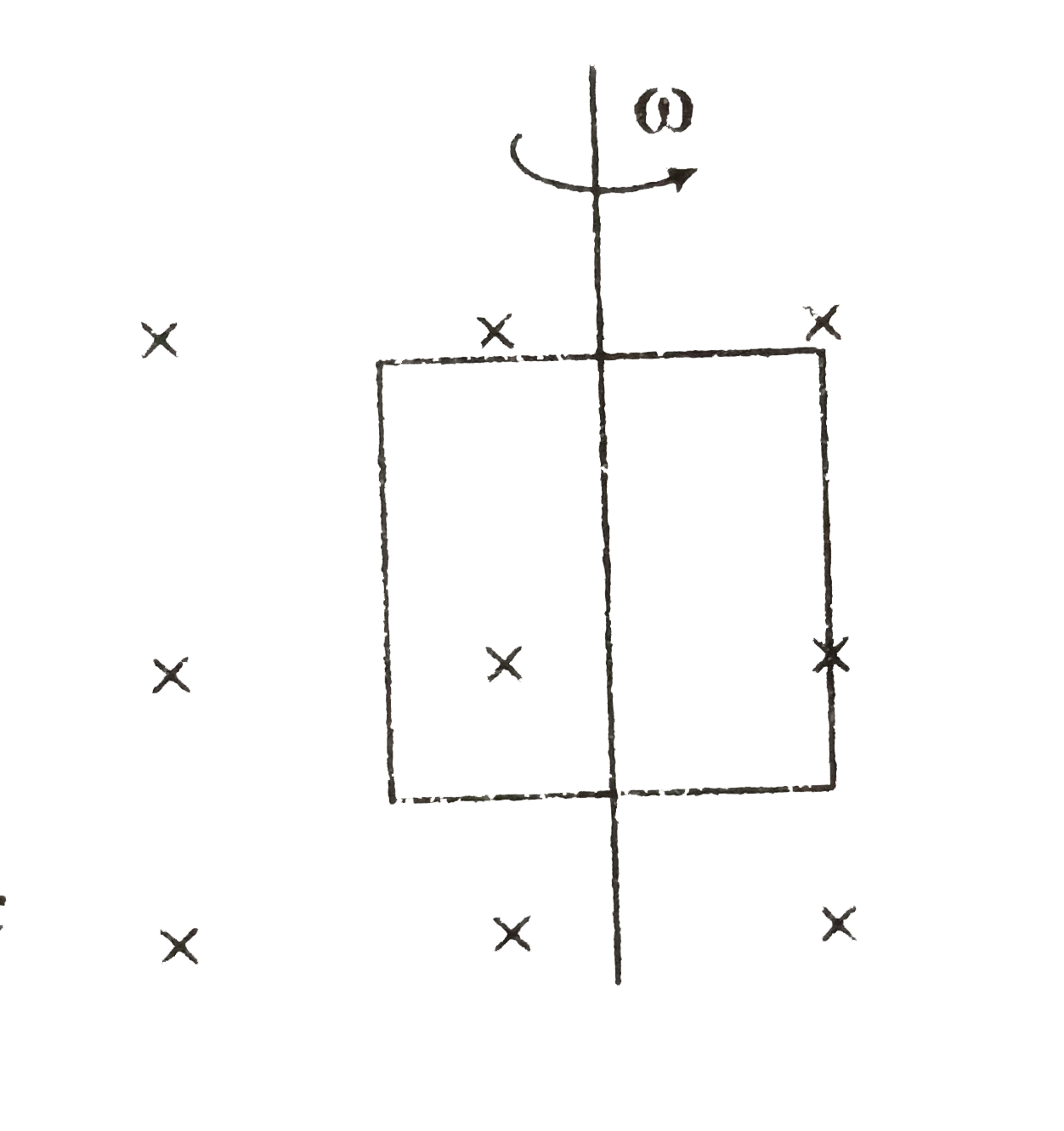 AIf both Assertion and Reason are corrent and Reason is the corrent explanation of Assertion.BIf both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.CIf Assertion is true but Reason is false.DIf Assertion is false but Reason is ture.
AIf both Assertion and Reason are corrent and Reason is the corrent explanation of Assertion.BIf both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.CIf Assertion is true but Reason is false.DIf Assertion is false but Reason is ture.
Assertion : Step-down transformer can also be used as step-up transformer.
Reason: Ratio of voltage across primary and secondary coils is the same as ratio of respective number of turns.
View SolutionAssertion (A): Whenever the magnetic flux linked with a closed coil changes there will be an induced emf as well as an induced current.
Reason (R ) : Accroding to Faraday, the induced emf is inversely proportional to the rate of change of magnetic flux linked with a coil.
View SolutionAssertion (A): An alpha particle placed in a magnetic field will not experience any force, if it moves in the magnetic field parallel to field lines.
Reason (R): The force is zero if current and field are in the same direction.
View SolutionAssertion : A given transformer can be used to step-up to step-down the voltage.
Reason : The output voltage depends upon the ratio of the number of turns of the two coils of the transformer.
View SolutionConsider a conducting circular loop placed in a magentic filed as shown. When magnetic field changes with time, magentic flux also changes and emf is induced.
e=−dϕdt
If resistance of loop is R then induced current.
i=eR
For Current, charge must have come into motion. Magnetic force cannot make the statinoary charges to move. Actually there is an induced electric field in the conductor caused by changing magnetic flux, which make the change to move
∫→E.d→l=e=−dϕdt
This induced electric field is non-electrostatic by nature. line integral of →E around a closed path is non-zero
A square non- conducting loop 20 cm on a side is placed in a magnetic field The centre of side AB coincides with the centre of magnetic field The magnetic field is increasing at the rate of 2T/s. Find the magnitude of line integral of induced electric field along path BC.
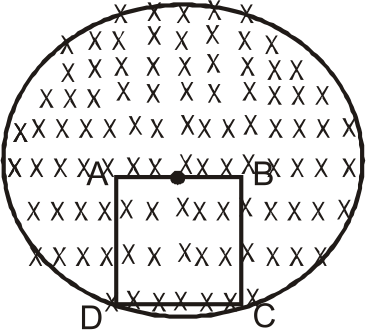
View Solution
