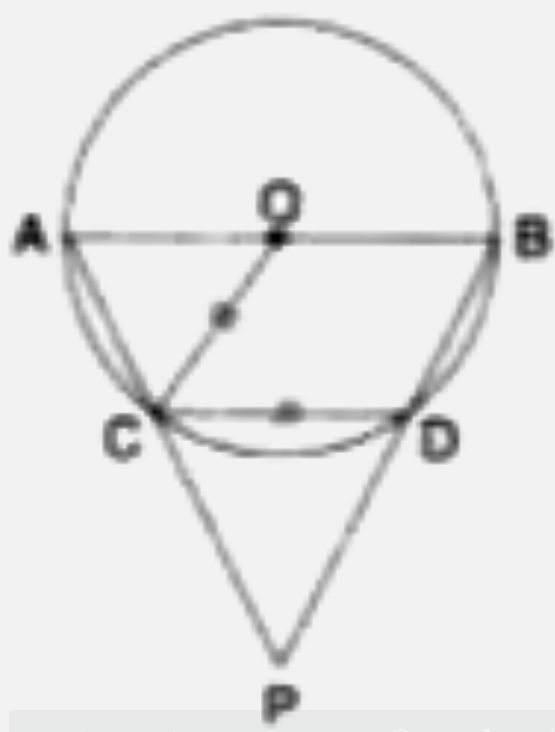
AB is a diameter of a circle with centre O. CD is a chord equal to the radius of the circle. AC and BD are produced to meet at P. Then the measure of $∠ A P B$ is:

A

$120^{\circ}$

B

$30^{\circ}$

C

$60^{\circ}$

D

$90^{\circ}$

Text Solution

Verified by Experts

The correct Answer is:C

|

Updated on:21/07/2023

Class 14 MATHS PRACTICE SET 7

Similar Questions

AB is diameter of a circle with centre 'O'. CD is a chord which is equal to the radius of the circle. AC and BD are extended so that they intersect each other at point P. Then find the value of $∠ A P B$ .
View Solution
AB is a diameter of a circle with centre O. Chord CD is equal to radius OC. AC and BD produced intersect at P. Prove that : $∠ A P B = 60^{\circ}$

View Solution

Knowledge Check

Question 1 - Select One
AB is a diameter of the circle, CD is a chord equal to the radius of the circle. AC and BD when extended intersect at a point E. Find the $∠ A E B$ .
A $30^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $45^{\circ}$
Question 2 - Select One
AB is a diameter of the circle with centre $O$ and chord $C D$ is equal to radius $O C$ (fig). $A C$ and $B D$ proudced meet at P. Prove that $∠ C P D = 60^{\circ}$ .

A $60^{\circ}$
B $30^{\circ}$
C $90^{\circ}$
D $160^{\circ}$

Recommended Questions

Home
Ask Doubt
Profile