Define angular velocity (ω). Derive v = r ω.
Angular velocity (ω) : Rate of change of angular displacement is . called angular velocity.
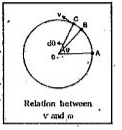
Relation between linear velocity (v) and angular velocity (ω) : Let a particle P.is moving along circumference of a circle of radius .. with uniform speed ya Let:it is initially at the position A, during a small time Δ t it goes to al new position sáy C from B. Angle subtended during this small interval is say d θ
By definition angular velocity
ω=LtΔt→0ΔθΔt=dθdt
But linear velocity v = LtΔt→0BCδt
When θ is small arc BC = r dθ
⇒v=rdθdt
∴v=r.ω(∴dθdt=ω)
Topper's Solved these Questions
- MODEL PAPER 2BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 2EXERCISE - SECTION C
3 Videos
- MODEL PAPER 2BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 2EXERCISE - SECTION C
3 Videos
- MODEL PAPER 13BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 13EXERCISE - SECTION - C
4 Videos
- MODEL PAPER 3 BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - MODEL PAPER 3 EXERCISE - SECTION-C
3 Videos
Similar Questions
Particles AandB move with constant and equal speeds in a circle as shown in (Fig. 5.164). Find the angular velocity of the particle A with respect to B, if the angular velocity of particle Aw.r.t.O is ω.
 .
.
View SolutionParticles AandB move with constant and equal speeds in a circle as shown in (Fig. 5.164). Find the angular velocity of the particle A with respect to B, if the angular velocity of particle Aw.r.t.O is ω.
 .
.
View Solution
Knowledge Check
A satellite of mass m revolves revolves round the earth of mass M in a circular orbit of radius r with an angular velocity ω. If the angular velocity is ω/8 then the radius of the orbit will be
A4rB2rC8rDrA sphere is moving on a smooth surface with angular velocity ω and linear velocity v. If collides elastically with another identical sphere B kept at rest. Neglecting friction everywhere, let VA and ωA be linear and angular velocities of sphere A after the impact and let VB and ωB be the corresponding parameters for spehre B. Then
AVA=0,ωA=0BVB=v,ωB=0CVB=0,ωB=ωDωA=0,ωB=ωA particle is moving in a circular orbit of radius r1 with an angular velocity ω1. It jumps to another circular orbit of radius r2 and attains an angular velocity ω2. IF r2=0.5r1 and assuming that no external torque is applied to that system, then, the angular velocity ω2 is given by
Aω2=4ω1Bω2=3ω1Cω2=2ω1Dω2=ω1
A ody is in pure rotation. The linear speed v of a particle, the distance r of the particle from the axis and the angular velocity ω of the body are related as ω=vr. Thus
View SolutionA body is in pure rotation. The linear speed 'v' of a particle, the distance 'r' of the particle from the axis and the angular velocity ω of the body are related as ω=vr. Thus
View SolutionA ody is in pure rotation. The linear speed v of a particle, the distance r of the particle from the axis and the angular velocity ω of the body are related as ω=vr. Thus
View SolutionA sphere is moving on a smooth surface with angular velocity ω and linear velocity v. If collides elastically with another identical sphere B kept at rest. Neglecting friction everywhere, let VA and ωA be linear and angular velocities of sphere A after the impact and let VB and ωB be the corresponding parameters for spehre B. Then
View SolutionAssertion: Vector product of two vectors is an axial vector.
Reason: If →v= instantaneous Velocity, →r= radius vector and →ω= angular velocity, then →ω=→v×→r.
View Solution
