Find the moment of inertia of a solid cylinder of mass M and radius R about a line parallel to the axis of the cylinder and on the surface of the cylinder.
To find the moment of inertia of a solid cylinder of mass M and radius R about a line parallel to the axis of the cylinder and on the surface of the cylinder, we can use the parallel axis theorem. Here’s a step-by-step solution:
Step 1: Moment of Inertia about the Central Axis
First, we need to find the moment of inertia of the solid cylinder about its central axis (the axis that goes through the center and is perpendicular to the circular face). The formula for the moment of inertia I of a solid cylinder about its central axis is given by:
Icentral=12MR2
Step 2: Identify the Distance to the New Axis
Next, we need to determine the distance d from the central axis to the new axis, which is parallel to the central axis and located on the surface of the cylinder. Since the new axis is on the surface, the distance d is equal to the radius R of the cylinder.
d=R
Step 3: Apply the Parallel Axis Theorem
The parallel axis theorem states that the moment of inertia about a new axis parallel to the original axis is given by:
I=Icentral+Md2
Where:
- I is the moment of inertia about the new axis,
- Icentral is the moment of inertia about the central axis,
- M is the mass of the cylinder,
- d is the distance between the two axes.
Step 4: Substitute the Values
Now we can substitute the values we have into the equation:
I=12MR2+MR2
Step 5: Simplify the Expression
Combining the terms gives us:
I=12MR2+1MR2=12MR2+22MR2=32MR2
Final Result
Thus, the moment of inertia of the solid cylinder about a line parallel to the axis of the cylinder and on the surface of the cylinder is:
I=32MR2
---
First, we need to find the moment of inertia of the solid cylinder about its central axis (the axis that goes through the center and is perpendicular to the circular face). The formula for the moment of inertia I of a solid cylinder about its central axis is given by:
Next, we need to determine the distance d from the central axis to the new axis, which is parallel to the central axis and located on the surface of the cylinder. Since the new axis is on the surface, the distance d is equal to the radius R of the cylinder.
The parallel axis theorem states that the moment of inertia about a new axis parallel to the original axis is given by:
- I is the moment of inertia about the new axis,
- Icentral is the moment of inertia about the central axis,
- M is the mass of the cylinder,
- d is the distance between the two axes.
Now we can substitute the values we have into the equation:
Combining the terms gives us:
Thus, the moment of inertia of the solid cylinder about a line parallel to the axis of the cylinder and on the surface of the cylinder is:
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Worked Out Examples
29 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Short Answer
22 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
Knowledge Check
Moment of inertia of a hollow cylinder of mass M and radius r about its own axis is
A23Mr2BMr2C13Mr2D12Mr2Moment of inertia of a hollow cylinder of mass M and radius R, about the axis of cylinder is
A12MR2BMR2C23MR2D25MR2The moment of inertia of cylinder of radius a, mass M and height h about an axis parallel to the axis of the cylinder and distance b from its centre is :
A12M(a2+2b2)B12M(2a2+b2)C12M(a2+b2)D12M(a23+b212)
The moment of inertia of a cylinder of mass 1000gm and radius 20cm about the axis AB shown in figure is

View SolutionThe radius of gyration of a solid cylinder of mass M and radius R about its own axis is
View SolutionMoment of inertia of a cylinder of mass M, length L and radius R about an axis passing through its centre and perpendicular to the axis of the cylinder is I=M(R24+L212). If such a cylinder is to be made for a given mass of a material, the ratio L/R for it to have minimum possible I is :
View SolutionWhat is moment of inertia of a cylinder of radius r, along its height?
View SolutionFind the moment of inertia of a circular disk or solid cylinder of radius R about the axis through the centre and perpendicular to the flat surface.
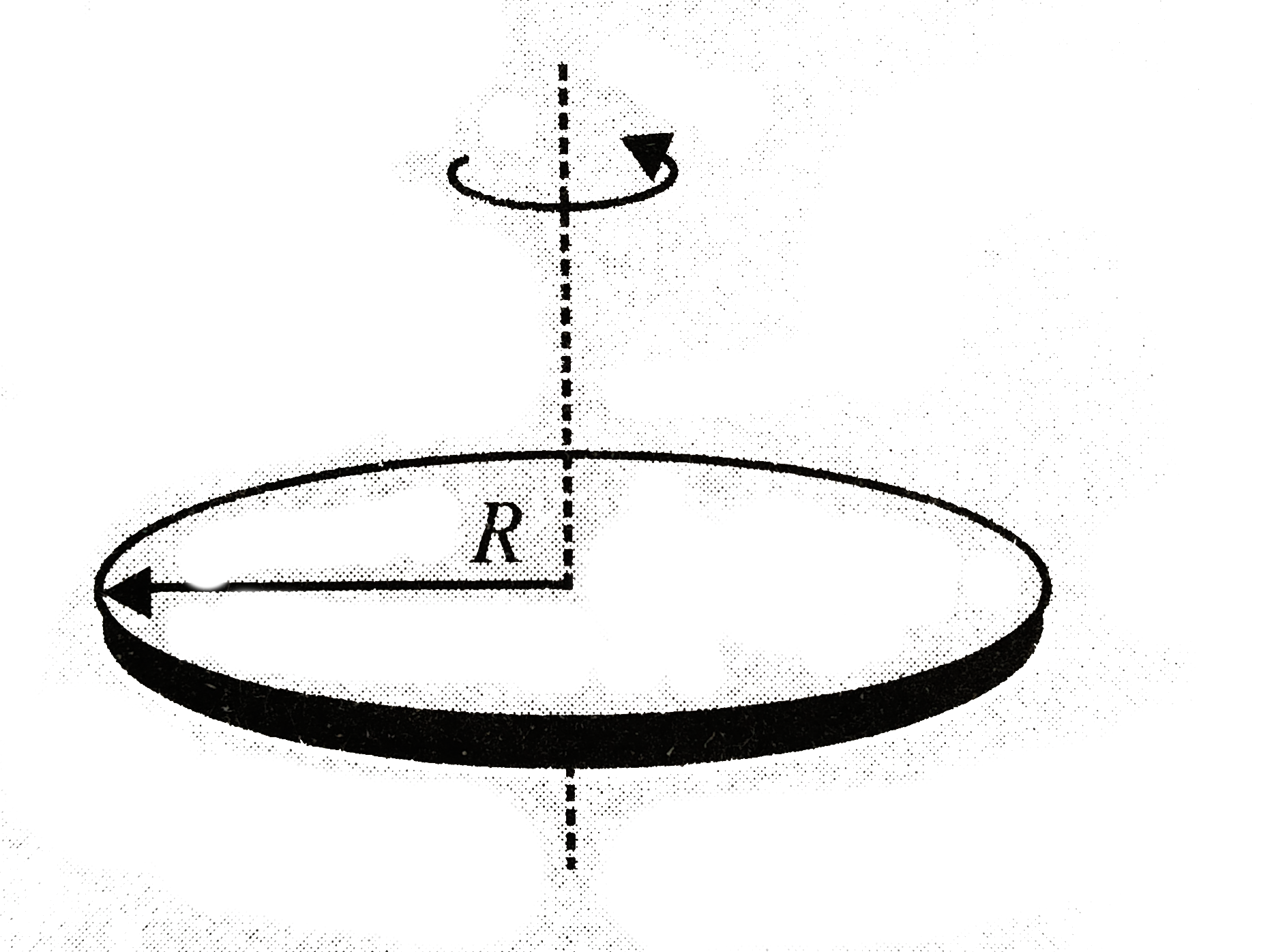
View Solution
